Question-168348

Question Number 168348 by mnjuly1970 last updated on 08/Apr/22

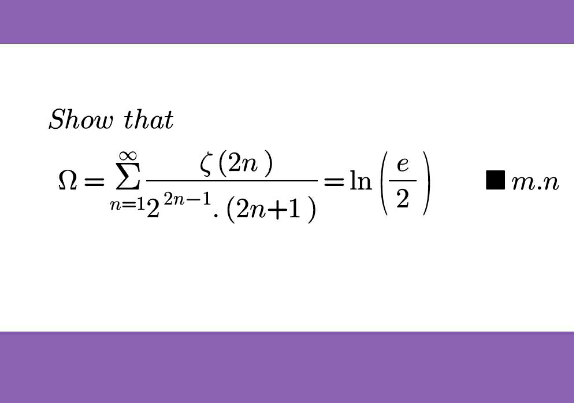
Answered by mathman1234 last updated on 09/Apr/22

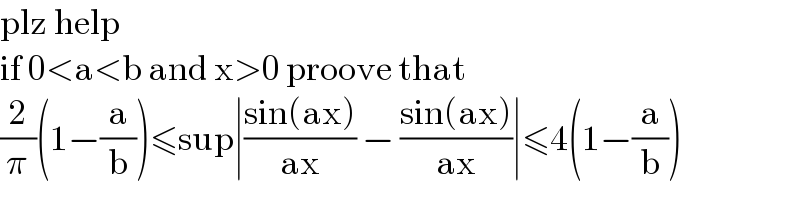
plz help if 0<a<b and x>0 proove that (2/π)(1−(a/b))≤sup∣((sin(ax))/(ax)) − ((sin(ax))/(ax))∣≤4(1−(a/b))

$$\mathrm{plz}\:\mathrm{help} \\ $$$$\mathrm{if}\:\mathrm{0}<\mathrm{a}<\mathrm{b}\:\mathrm{and}\:\mathrm{x}>\mathrm{0}\:\mathrm{proove}\:\mathrm{that} \\ $$$$\frac{\mathrm{2}}{\pi}\left(\mathrm{1}−\frac{\mathrm{a}}{\mathrm{b}}\right)\leqslant\mathrm{sup}\mid\frac{\mathrm{sin}\left(\mathrm{ax}\right)}{\mathrm{ax}}\:−\:\frac{\mathrm{sin}\left(\mathrm{ax}\right)}{\mathrm{ax}}\mid\leqslant\mathrm{4}\left(\mathrm{1}−\frac{\mathrm{a}}{\mathrm{b}}\right) \\ $$

Commented by mr W last updated on 09/Apr/22

$${please}\:{don}'{t}\:{put}\:{your}\:{new}\:{question}\:{in} \\ $$$${existing}\:{threads}\:{of}\:{other}\:{people}'{s} \\ $$$${questions}!\:{please}\:{open}\:{a}\:{new}\:{post} \\ $$$${for}\:{your}\:{own}\:{question}! \\ $$

Answered by mindispower last updated on 11/Apr/22

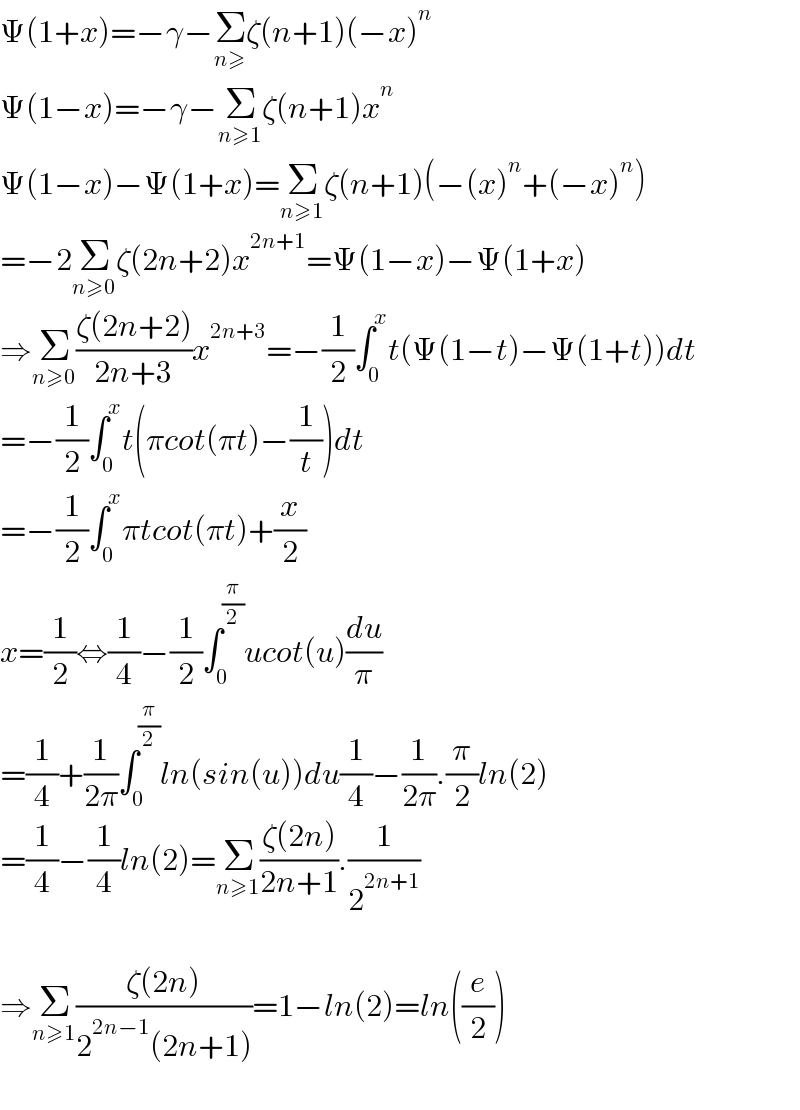
Ψ(1+x)=−γ−Σ_(n≥) ζ(n+1)(−x)^n Ψ(1−x)=−γ−Σ_(n≥1) ζ(n+1)x^n Ψ(1−x)−Ψ(1+x)=Σ_(n≥1) ζ(n+1)(−(x)^n +(−x)^n ) =−2Σ_(n≥0) ζ(2n+2)x^(2n+1) =Ψ(1−x)−Ψ(1+x) ⇒Σ_(n≥0) ((ζ(2n+2))/(2n+3))x^(2n+3) =−(1/2)∫_0 ^x t(Ψ(1−t)−Ψ(1+t))dt =−(1/2)∫_0 ^x t(πcot(πt)−(1/t))dt =−(1/2)∫_0 ^x πtcot(πt)+(x/2) x=(1/2)⇔(1/4)−(1/2)∫_0 ^(π/2) ucot(u)(du/π) =(1/4)+(1/(2π))∫_0 ^(π/2) ln(sin(u))du(1/4)−(1/(2π)).(π/2)ln(2) =(1/4)−(1/4)ln(2)=Σ_(n≥1) ((ζ(2n))/(2n+1)).(1/2^(2n+1) ) ⇒Σ_(n≥1) ((ζ(2n))/(2^(2n−1) (2n+1)))=1−ln(2)=ln((e/2))

$$\Psi\left(\mathrm{1}+{x}\right)=−\gamma−\underset{{n}\geqslant} {\sum}\zeta\left({n}+\mathrm{1}\right)\left(−{x}\right)^{{n}} \\ $$$$\Psi\left(\mathrm{1}−{x}\right)=−\gamma−\underset{{n}\geqslant\mathrm{1}} {\sum}\zeta\left({n}+\mathrm{1}\right){x}^{{n}} \\ $$$$\Psi\left(\mathrm{1}−{x}\right)−\Psi\left(\mathrm{1}+{x}\right)=\underset{{n}\geqslant\mathrm{1}} {\sum}\zeta\left({n}+\mathrm{1}\right)\left(−\left({x}\right)^{{n}} +\left(−{x}\right)^{{n}} \right) \\ $$$$=−\mathrm{2}\underset{{n}\geqslant\mathrm{0}} {\sum}\zeta\left(\mathrm{2}{n}+\mathrm{2}\right){x}^{\mathrm{2}{n}+\mathrm{1}} =\Psi\left(\mathrm{1}−{x}\right)−\Psi\left(\mathrm{1}+{x}\right) \\ $$$$\Rightarrow\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{\zeta\left(\mathrm{2}{n}+\mathrm{2}\right)}{\mathrm{2}{n}+\mathrm{3}}{x}^{\mathrm{2}{n}+\mathrm{3}} =−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{{x}} {t}\left(\Psi\left(\mathrm{1}−{t}\right)−\Psi\left(\mathrm{1}+{t}\right)\right){dt} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{{x}} {t}\left(\pi{cot}\left(\pi{t}\right)−\frac{\mathrm{1}}{{t}}\right){dt} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{{x}} \pi{tcot}\left(\pi{t}\right)+\frac{{x}}{\mathrm{2}} \\ $$$${x}=\frac{\mathrm{1}}{\mathrm{2}}\Leftrightarrow\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ucot}\left({u}\right)\frac{{du}}{\pi} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}\pi}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left({sin}\left({u}\right)\right){du}\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}\pi}.\frac{\pi}{\mathrm{2}}{ln}\left(\mathrm{2}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{4}}{ln}\left(\mathrm{2}\right)=\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\zeta\left(\mathrm{2}{n}\right)}{\mathrm{2}{n}+\mathrm{1}}.\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}{n}+\mathrm{1}} } \\ $$$$ \\ $$$$\Rightarrow\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\zeta\left(\mathrm{2}{n}\right)}{\mathrm{2}^{\mathrm{2}{n}−\mathrm{1}} \left(\mathrm{2}{n}+\mathrm{1}\right)}=\mathrm{1}−{ln}\left(\mathrm{2}\right)={ln}\left(\frac{{e}}{\mathrm{2}}\right) \\ $$$$ \\ $$

Commented by mnjuly1970 last updated on 13/Apr/22

$$\:\:\:\:{grateful}\:{sir}\:\:{power}… \\ $$

Commented by mindispower last updated on 13/Apr/22

$${withe}\:{Pleasur}\:{sir}\:{Have}\:{nice}\:{Day} \\ $$$${Ramadan}\:{Mobarak} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply