Question Number 37344 by math khazana by abdo last updated on 12/Jun/18
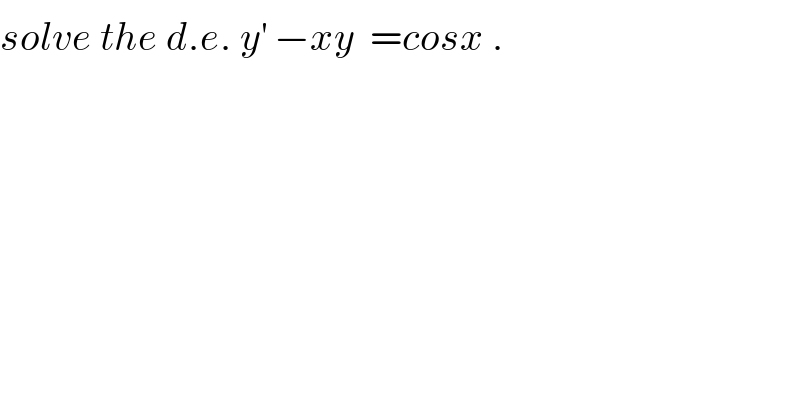
$${solve}\:{the}\:{d}.{e}.\:{y}^{'} \:−{xy}\:\:={cosx}\:. \\ $$
Commented by math khazana by abdo last updated on 12/Jun/18
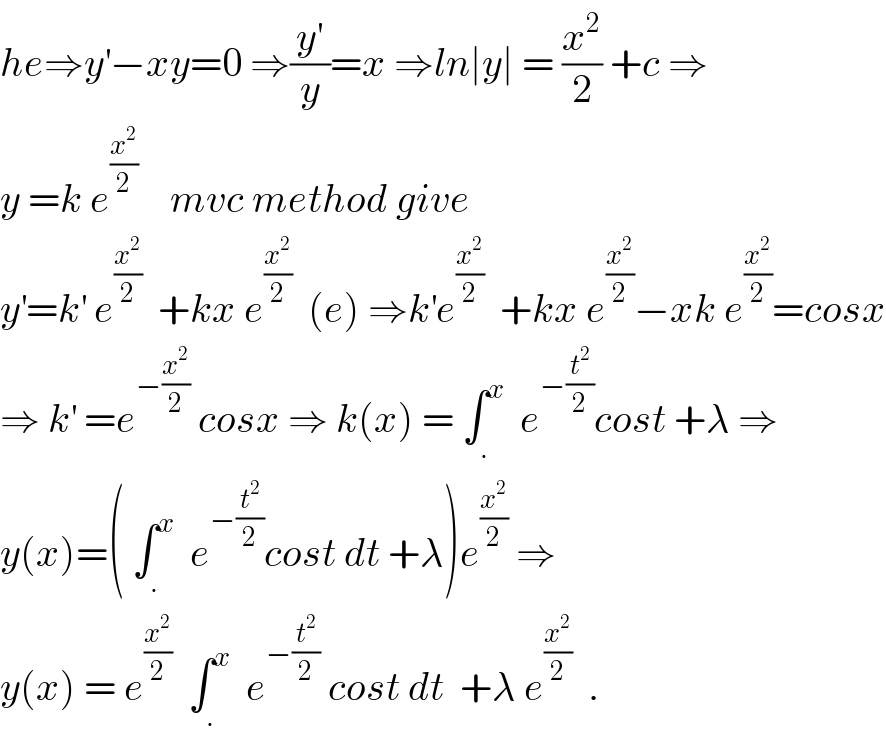
$${he}\Rightarrow{y}^{'} −{xy}=\mathrm{0}\:\Rightarrow\frac{{y}^{'} }{{y}}={x}\:\Rightarrow{ln}\mid{y}\mid\:=\:\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\:+{c}\:\Rightarrow \\ $$$${y}\:={k}\:{e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} \:\:\:\:{mvc}\:{method}\:{give}\: \\ $$$${y}^{'} ={k}^{'} \:{e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} \:\:+{kx}\:{e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} \:\:\left({e}\right)\:\Rightarrow{k}^{'} {e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} \:\:+{kx}\:{e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} −{xk}\:{e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} ={cosx} \\ $$$$\Rightarrow\:{k}^{'} \:={e}^{−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} \:{cosx}\:\Rightarrow\:{k}\left({x}\right)\:=\:\int_{.} ^{{x}} \:\:{e}^{−\frac{{t}^{\mathrm{2}} }{\mathrm{2}}} {cost}\:+\lambda\:\Rightarrow \\ $$$${y}\left({x}\right)=\left(\:\int_{.} ^{{x}} \:\:{e}^{−\frac{{t}^{\mathrm{2}} }{\mathrm{2}}} {cost}\:{dt}\:+\lambda\right){e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} \:\Rightarrow \\ $$$${y}\left({x}\right)\:=\:{e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} \:\:\int_{.} ^{{x}} \:\:{e}^{−\frac{{t}^{\mathrm{2}} }{\mathrm{2}}} \:{cost}\:{dt}\:\:+\lambda\:{e}^{\frac{{x}^{\mathrm{2}} }{\mathrm{2}}} \:\:. \\ $$
