Question Number 3682 by Yozzii last updated on 19/Dec/15

$$\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{r}\mathrm{2}^{{r}} }=? \\ $$
Commented by RasheedSindhi last updated on 19/Dec/15
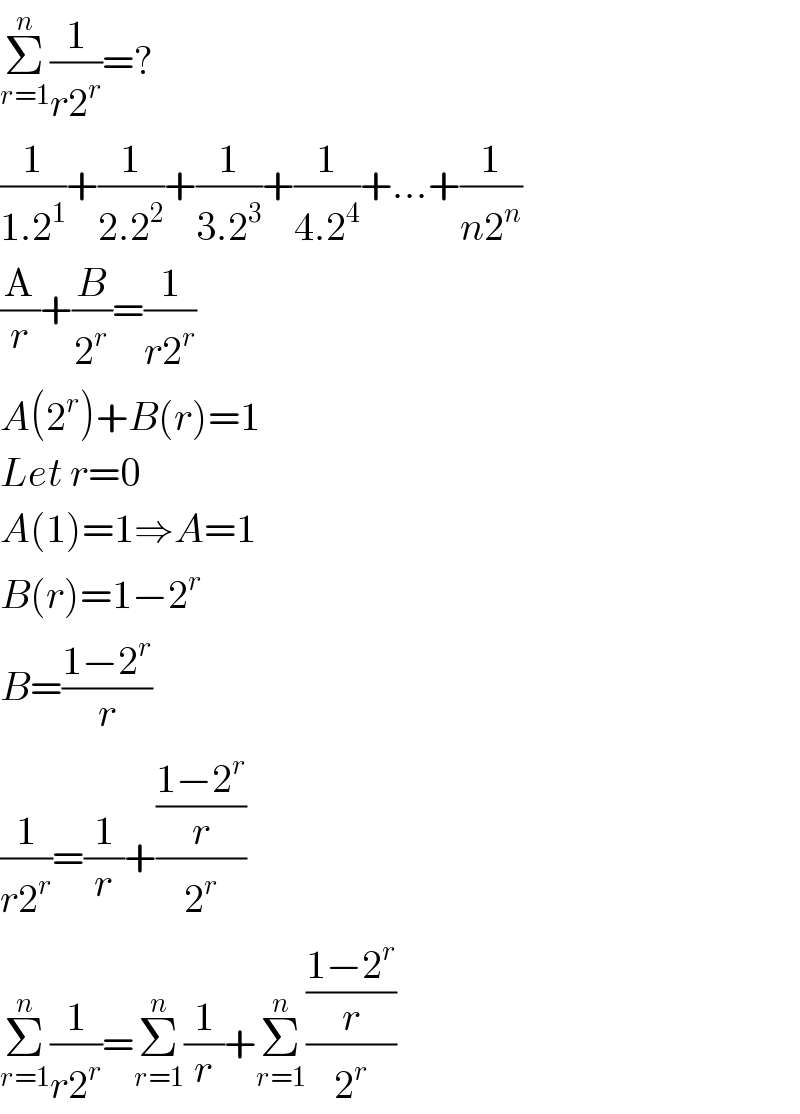
$$\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{r}\mathrm{2}^{{r}} }=? \\ $$$$\frac{\mathrm{1}}{\mathrm{1}.\mathrm{2}^{\mathrm{1}} }+\frac{\mathrm{1}}{\mathrm{2}.\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}.\mathrm{2}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{4}.\mathrm{2}^{\mathrm{4}} }+…+\frac{\mathrm{1}}{{n}\mathrm{2}^{{n}} } \\ $$$$\frac{\mathrm{A}}{{r}}+\frac{{B}}{\mathrm{2}^{{r}} }=\frac{\mathrm{1}}{{r}\mathrm{2}^{{r}} } \\ $$$${A}\left(\mathrm{2}^{{r}} \right)+{B}\left({r}\right)=\mathrm{1} \\ $$$${Let}\:{r}=\mathrm{0} \\ $$$${A}\left(\mathrm{1}\right)=\mathrm{1}\Rightarrow{A}=\mathrm{1} \\ $$$${B}\left({r}\right)=\mathrm{1}−\mathrm{2}^{{r}} \\ $$$${B}=\frac{\mathrm{1}−\mathrm{2}^{{r}} }{{r}} \\ $$$$\frac{\mathrm{1}}{{r}\mathrm{2}^{{r}} }=\frac{\mathrm{1}}{{r}}+\frac{\frac{\mathrm{1}−\mathrm{2}^{{r}} }{{r}}}{\mathrm{2}^{{r}} } \\ $$$$\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{r}\mathrm{2}^{{r}} }=\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{r}}+\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\frac{\mathrm{1}−\mathrm{2}^{{r}} }{{r}}}{\mathrm{2}^{{r}} } \\ $$
Commented by Yozzii last updated on 19/Dec/15

$${y}={q}^{−{r}−\mathrm{1}} \Rightarrow\int_{\mathrm{1}} ^{\mathrm{2}} {q}^{−{r}−\mathrm{1}} {dq}=\frac{\mathrm{1}}{−{rq}^{{r}} }\mid_{\mathrm{1}} ^{\mathrm{2}} \\ $$$$\Rightarrow\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\left\{\int_{\mathrm{1}} ^{\mathrm{2}} \left({q}^{−\mathrm{1}} \right)^{{r}+\mathrm{1}} {dq}\right\}=−\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\left(\frac{\mathrm{1}}{{r}\mathrm{2}^{{r}} }−\frac{\mathrm{1}}{{r}}\right) \\ $$$$\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{rq}^{{r}} }=\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{r}}+\int_{\mathrm{1}} ^{\mathrm{2}} \left({q}^{−\mathrm{1}} \right)^{\mathrm{2}} \underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\left({q}^{−\mathrm{1}} \right)^{{r}−\mathrm{1}} {d}\overset{} {{q}} \\ $$$$\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{rq}^{{r}} }=\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{r}}+\underset{\mathrm{1}} {\int}^{\mathrm{2}} \left({q}^{−\mathrm{1}} \right)^{\mathrm{2}} \frac{\left({q}^{−{n}} −\mathrm{1}\right)}{{q}^{−\mathrm{1}} −\mathrm{1}}{dq} \\ $$$${S}\left({n}\right)={H}\left({n}\right)+\int_{\mathrm{1}} ^{\mathrm{2}} \left({q}^{−\mathrm{1}} \right)^{\mathrm{2}} \frac{\mathrm{1}−{q}^{{n}} }{{q}^{{n}} \left(\mathrm{1}−{q}\right)/{q}}{dq} \\ $$$${S}\left({n}\right)−{H}\left({n}\right)=\int_{\mathrm{1}} ^{\mathrm{2}} \frac{\mathrm{1}−{q}^{{n}} }{{q}^{{n}+\mathrm{1}} \left(\mathrm{1}−{q}\right)}{dq} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by Rasheed Soomro last updated on 19/Dec/15

$$\frac{\mathrm{1}}{{r}\mathrm{2}^{{r}} }=\frac{{A}}{{r}}+\frac{{B}_{\mathrm{1}} }{\mathrm{2}}+\frac{{B}_{\mathrm{2}} }{\mathrm{2}^{\mathrm{2}} }+\frac{{B}_{\mathrm{3}} }{\mathrm{2}^{\mathrm{3}} }+…+\frac{{B}_{{r}} }{\mathrm{2}^{{r}} } \\ $$$${A}\left(\mathrm{2}^{{r}} \right)+{B}_{\mathrm{1}} \left({r}\mathrm{2}^{{r}−\mathrm{1}} \right)+{B}_{\mathrm{2}} \left({r}\mathrm{2}^{{r}−\mathrm{2}} \right)+{B}_{\mathrm{3}} \left({r}\mathrm{2}^{{r}−\mathrm{3}} \right)+…+{B}_{{r}} \left({r}\right)=\mathrm{1} \\ $$$${A}\:{can}\:{be}\:{determined}\:{by}\:{r}=\mathrm{0} \\ $$$${B}_{\mathrm{1}} ,{B}_{\mathrm{2}} ,…{B}_{{r}} =??? \\ $$$${How}\:{can}\:{we}\:{determine}\:{partial}\:{fractions}\:{of}\:\:\frac{\mathrm{1}}{{r}\mathrm{2}^{{r}} }\:? \\ $$
Commented by 123456 last updated on 19/Dec/15

$$\mathrm{i}\:\mathrm{think}\:\mathrm{it}\:\mathrm{cant}\:\mathrm{be}\:\mathrm{made} \\ $$$$\mathrm{or}\:\mathrm{the}\:\mathrm{coeficients}\:\mathrm{A},\mathrm{B}… \\ $$$$\mathrm{doenst}\:\mathrm{be}\:\mathrm{constant} \\ $$
Commented by prakash jain last updated on 19/Dec/15

$${S}\left({n}\right)={H}\left({n}\right)+..\:\mathrm{has}\:\mathrm{may}\:\mathrm{have}\:\mathrm{mistake}\:\mathrm{somewhere} \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\:{S}\left({n}\right)=\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\mathrm{1}}{{r}\centerdot\mathrm{2}^{{r}} }=\mathrm{ln}\:\left(\mathrm{2}\right) \\ $$
Commented by prakash jain last updated on 19/Dec/15

$$\underset{{i}=\mathrm{2}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{x}^{{i}} }=\frac{\mathrm{1}/{x}^{\mathrm{2}} }{\mathrm{1}−\mathrm{1}/{x}},\:\:\:\:\:\:\:\:\:\mid{x}\mid>\mathrm{1} \\ $$$$\mathrm{integrate} \\ $$$$−\underset{{i}=\mathrm{2}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left({i}−\mathrm{1}\right){x}^{{i}−\mathrm{1}} }=\int\frac{\mathrm{1}}{{x}\left({x}−\mathrm{1}\right)}=\mathrm{ln}\:\left({x}−\mathrm{1}\right)−\mathrm{ln}\:{x} \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left({i}\right){x}^{{i}} }=\int\frac{\mathrm{1}}{{x}\left({x}−\mathrm{1}\right)}=−\mathrm{ln}\:\left({x}−\mathrm{1}\right)+\mathrm{ln}\:{x} \\ $$$${x}=\mathrm{2} \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\frac{\mathrm{1}}{{i}\mathrm{2}^{{i}} }=\mathrm{ln}\:\mathrm{2} \\ $$
