Question Number 103512 by bemath last updated on 15/Jul/20
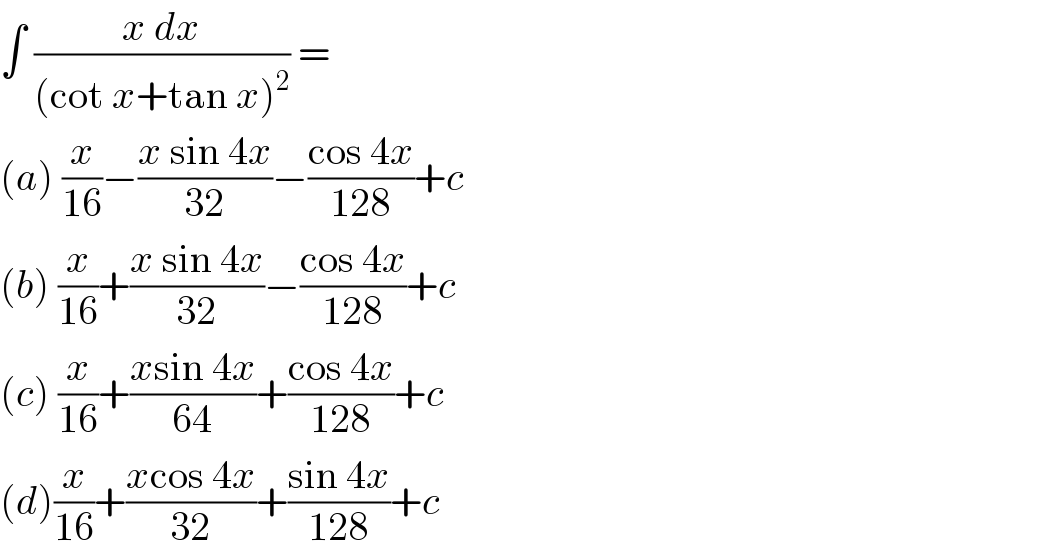
$$\int\:\frac{{x}\:{dx}}{\left(\mathrm{cot}\:{x}+\mathrm{tan}\:{x}\right)^{\mathrm{2}} }\:= \\ $$$$\left({a}\right)\:\frac{{x}}{\mathrm{16}}−\frac{{x}\:\mathrm{sin}\:\mathrm{4}{x}}{\mathrm{32}}−\frac{\mathrm{cos}\:\mathrm{4}{x}}{\mathrm{128}}+{c} \\ $$$$\left({b}\right)\:\frac{{x}}{\mathrm{16}}+\frac{{x}\:\mathrm{sin}\:\mathrm{4}{x}}{\mathrm{32}}−\frac{\mathrm{cos}\:\mathrm{4}{x}}{\mathrm{128}}+{c} \\ $$$$\left({c}\right)\:\frac{{x}}{\mathrm{16}}+\frac{{x}\mathrm{sin}\:\mathrm{4}{x}}{\mathrm{64}}+\frac{\mathrm{cos}\:\mathrm{4}{x}}{\mathrm{128}}+{c} \\ $$$$\left({d}\right)\frac{{x}}{\mathrm{16}}+\frac{{x}\mathrm{cos}\:\mathrm{4}{x}}{\mathrm{32}}+\frac{\mathrm{sin}\:\mathrm{4}{x}}{\mathrm{128}}+{c} \\ $$
Commented by bobhans last updated on 15/Jul/20
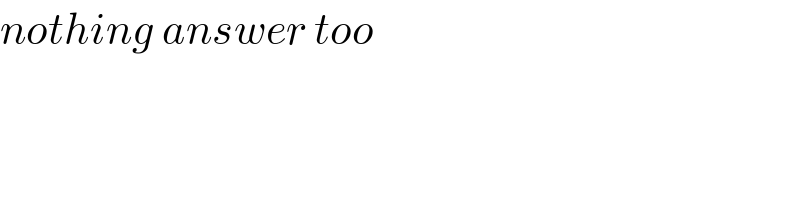
$${nothing}\:{answer}\:{too} \\ $$
Commented by bemath last updated on 15/Jul/20
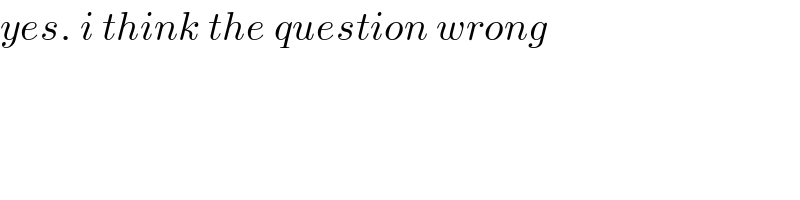
$${yes}.\:{i}\:{think}\:{the}\:{question}\:{wrong} \\ $$
Answered by Dwaipayan Shikari last updated on 15/Jul/20
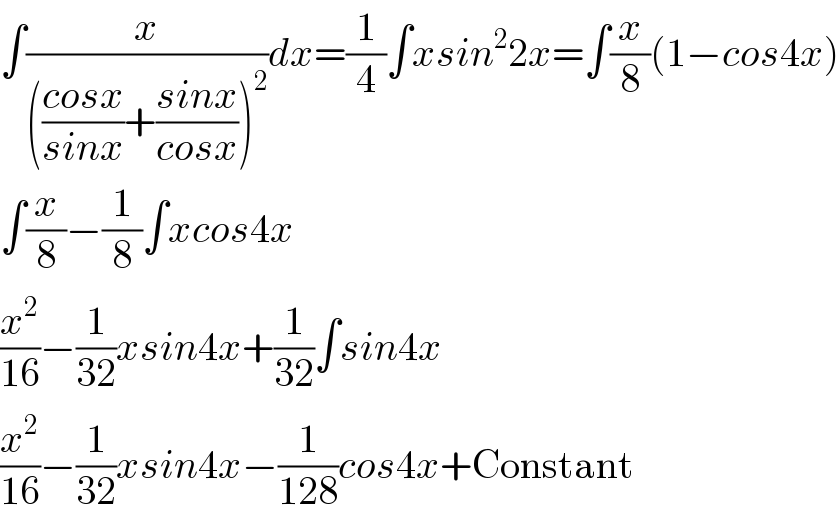
$$\int\frac{{x}}{\left(\frac{{cosx}}{{sinx}}+\frac{{sinx}}{{cosx}}\right)^{\mathrm{2}} }{dx}=\frac{\mathrm{1}}{\mathrm{4}}\int{xsin}^{\mathrm{2}} \mathrm{2}{x}=\int\frac{{x}}{\mathrm{8}}\left(\mathrm{1}−{cos}\mathrm{4}{x}\right) \\ $$$$\int\frac{{x}}{\mathrm{8}}−\frac{\mathrm{1}}{\mathrm{8}}\int{xcos}\mathrm{4}{x} \\ $$$$\frac{{x}^{\mathrm{2}} }{\mathrm{16}}−\frac{\mathrm{1}}{\mathrm{32}}{xsin}\mathrm{4}{x}+\frac{\mathrm{1}}{\mathrm{32}}\int{sin}\mathrm{4}{x} \\ $$$$\frac{{x}^{\mathrm{2}} }{\mathrm{16}}−\frac{\mathrm{1}}{\mathrm{32}}{xsin}\mathrm{4}{x}−\frac{\mathrm{1}}{\mathrm{128}}{cos}\mathrm{4}{x}+\mathrm{Constant} \\ $$
Answered by bramlex last updated on 15/Jul/20
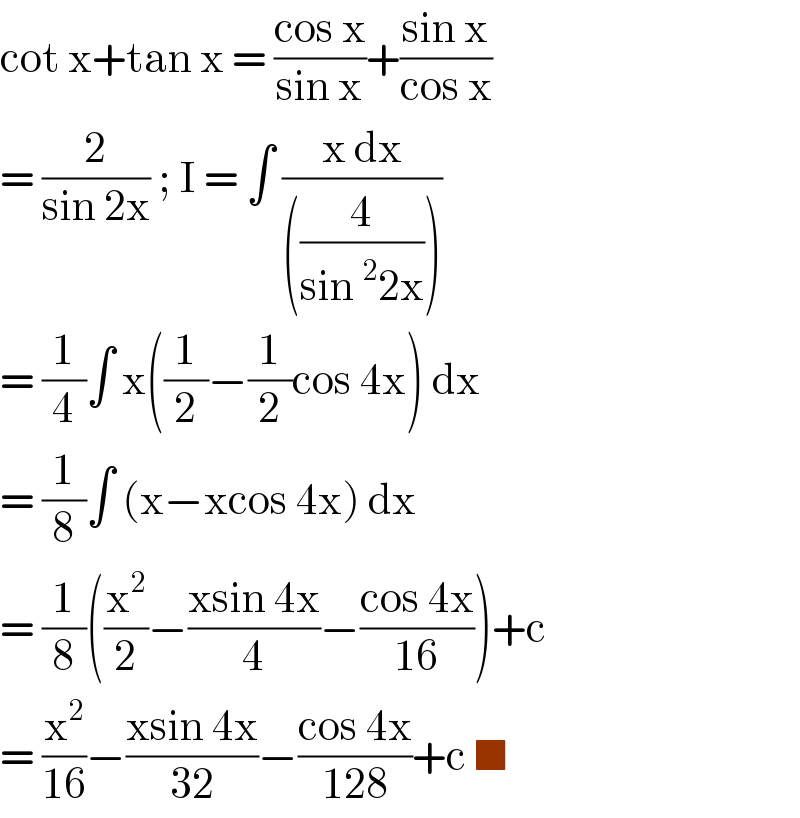
$$\mathrm{cot}\:\mathrm{x}+\mathrm{tan}\:\mathrm{x}\:=\:\frac{\mathrm{cos}\:\mathrm{x}}{\mathrm{sin}\:\mathrm{x}}+\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{cos}\:\mathrm{x}} \\ $$$$=\:\frac{\mathrm{2}}{\mathrm{sin}\:\mathrm{2x}}\:;\:\mathrm{I}\:=\:\int\:\frac{\mathrm{x}\:\mathrm{dx}}{\left(\frac{\mathrm{4}}{\mathrm{sin}\:^{\mathrm{2}} \mathrm{2x}}\right)} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{4}}\int\:\mathrm{x}\left(\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\mathrm{4x}\right)\:\mathrm{dx}\: \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{8}}\int\:\left(\mathrm{x}−\mathrm{xcos}\:\mathrm{4x}\right)\:\mathrm{dx}\: \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{8}}\left(\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}−\frac{\mathrm{xsin}\:\mathrm{4x}}{\mathrm{4}}−\frac{\mathrm{cos}\:\mathrm{4x}}{\mathrm{16}}\right)+\mathrm{c} \\ $$$$=\:\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{16}}−\frac{\mathrm{xsin}\:\mathrm{4x}}{\mathrm{32}}−\frac{\mathrm{cos}\:\mathrm{4x}}{\mathrm{128}}+\mathrm{c}\:\blacksquare \\ $$
