Menu Close

x-4-23x-2-18x-40-0-solve-for-x-

Tinku Tara
Pin




Question Number 65853 by ajfour last updated on 05/Aug/19
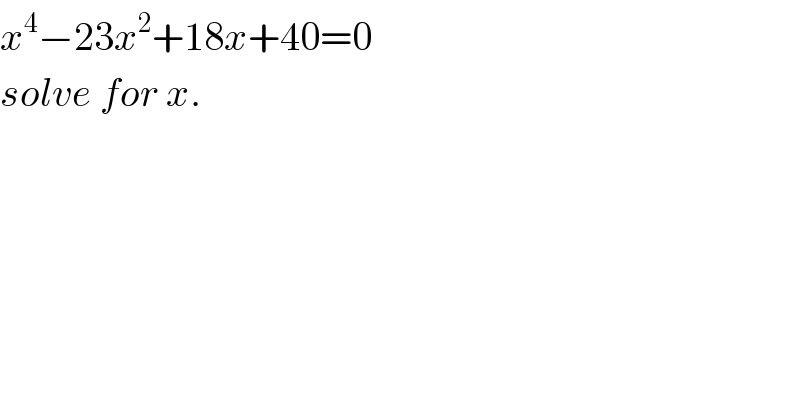
x^4 −23x^2 +18x+40=0 solve for x.
$${x}^{\mathrm{4}} −\mathrm{23}{x}^{\mathrm{2}} +\mathrm{18}{x}+\mathrm{40}=\mathrm{0} \\ $$$${solve}\:{for}\:{x}. \\ $$
Answered by ajfour last updated on 05/Aug/19
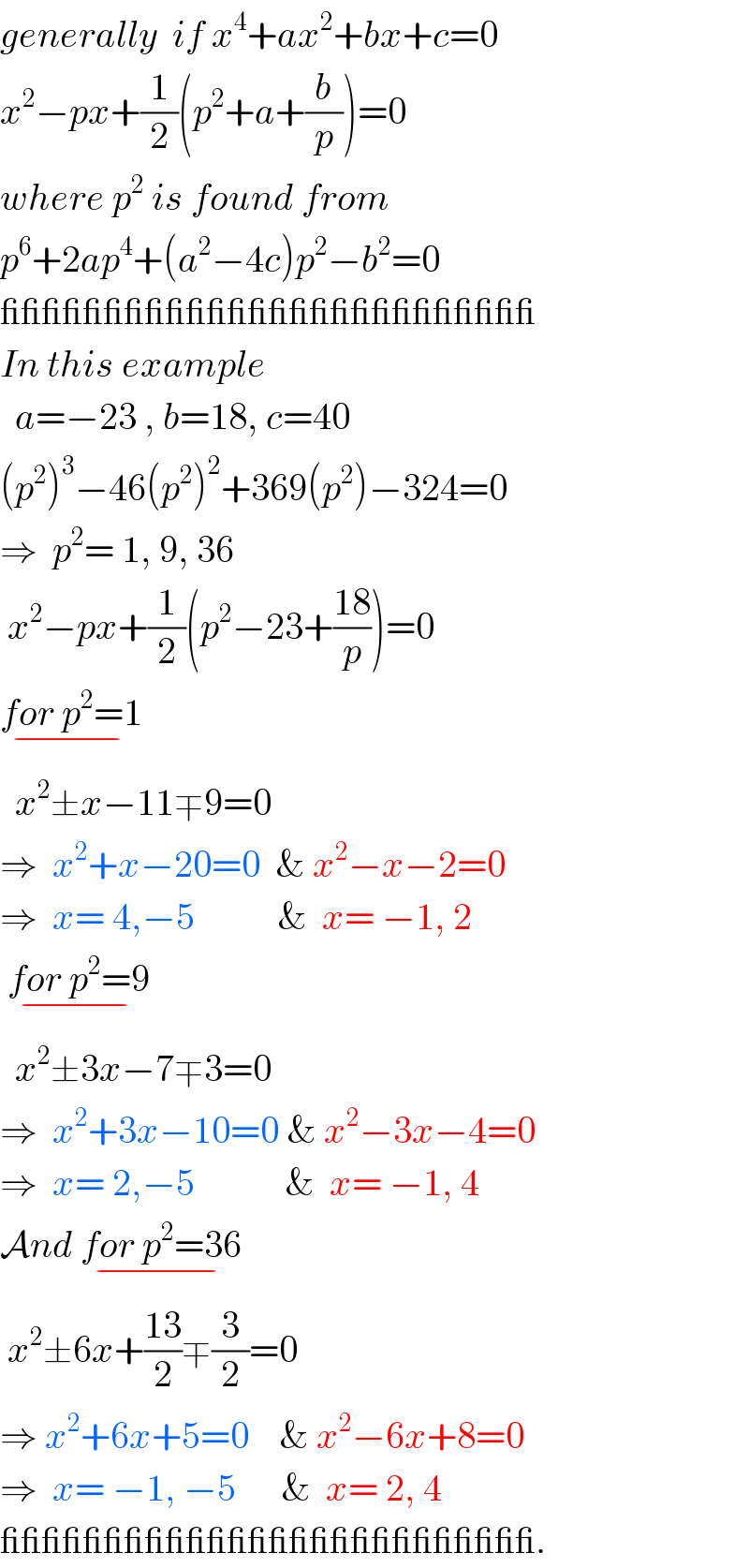
generally if x^4 +ax^2 +bx+c=0 x^2 −px+(1/2)(p^2 +a+(b/p))=0 where p^2 is found from p^6 +2ap^4 +(a^2 −4c)p^2 −b^2 =0 __________________________ In this example a=−23 , b=18, c=40 (p^2 )^3 −46(p^2 )^2 +369(p^2 )−324=0 ⇒ p^2 = 1, 9, 36 x^2 −px+(1/2)(p^2 −23+((18)/p))=0 for p^2 =1_(−) x^2 ±x−11∓9=0 ⇒ x^2 +x−20=0 & x^2 −x−2=0 ⇒ x= 4,−5 & x= −1, 2 for p^2 =9_(−) x^2 ±3x−7∓3=0 ⇒ x^2 +3x−10=0 & x^2 −3x−4=0 ⇒ x= 2,−5 & x= −1, 4 And for p^2 =36_(−) x^2 ±6x+((13)/2)∓(3/2)=0 ⇒ x^2 +6x+5=0 & x^2 −6x+8=0 ⇒ x= −1, −5 & x= 2, 4 __________________________.
$${generally}\:\:{if}\:{x}^{\mathrm{4}} +{ax}^{\mathrm{2}} +{bx}+{c}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} −{px}+\frac{\mathrm{1}}{\mathrm{2}}\left({p}^{\mathrm{2}} +{a}+\frac{{b}}{{p}}\right)=\mathrm{0} \\ $$$${where}\:{p}^{\mathrm{2}} \:{is}\:{found}\:{from} \\ $$$${p}^{\mathrm{6}} +\mathrm{2}{ap}^{\mathrm{4}} +\left({a}^{\mathrm{2}} −\mathrm{4}{c}\right){p}^{\mathrm{2}} −{b}^{\mathrm{2}} =\mathrm{0} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$${In}\:{this}\:{example} \\ $$$$\:\:{a}=−\mathrm{23}\:,\:{b}=\mathrm{18},\:{c}=\mathrm{40} \\ $$$$\left({p}^{\mathrm{2}} \right)^{\mathrm{3}} −\mathrm{46}\left({p}^{\mathrm{2}} \right)^{\mathrm{2}} +\mathrm{369}\left({p}^{\mathrm{2}} \right)−\mathrm{324}=\mathrm{0} \\ $$$$\Rightarrow\:\:{p}^{\mathrm{2}} =\:\mathrm{1},\:\mathrm{9},\:\mathrm{36} \\ $$$$\:{x}^{\mathrm{2}} −{px}+\frac{\mathrm{1}}{\mathrm{2}}\left({p}^{\mathrm{2}} −\mathrm{23}+\frac{\mathrm{18}}{{p}}\right)=\mathrm{0} \\ $$$$\underset{−} {{for}\:{p}^{\mathrm{2}} =\mathrm{1}} \\ $$$$\:\:{x}^{\mathrm{2}} \pm{x}−\mathrm{11}\mp\mathrm{9}=\mathrm{0} \\ $$$$\Rightarrow\:\:{x}^{\mathrm{2}} +{x}−\mathrm{20}=\mathrm{0}\:\:\&\:{x}^{\mathrm{2}} −{x}−\mathrm{2}=\mathrm{0} \\ $$$$\Rightarrow\:\:{x}=\:\mathrm{4},−\mathrm{5}\:\:\:\:\:\:\:\:\:\:\:\&\:\:{x}=\:−\mathrm{1},\:\mathrm{2}\:\:\: \\ $$$$\:\underset{−} {{for}\:{p}^{\mathrm{2}} =\mathrm{9}} \\ $$$$\:\:{x}^{\mathrm{2}} \pm\mathrm{3}{x}−\mathrm{7}\mp\mathrm{3}=\mathrm{0} \\ $$$$\Rightarrow\:\:{x}^{\mathrm{2}} +\mathrm{3}{x}−\mathrm{10}=\mathrm{0}\:\&\:{x}^{\mathrm{2}} −\mathrm{3}{x}−\mathrm{4}=\mathrm{0} \\ $$$$\Rightarrow\:\:{x}=\:\mathrm{2},−\mathrm{5}\:\:\:\:\:\:\:\:\:\:\:\:\&\:\:{x}=\:−\mathrm{1},\:\mathrm{4} \\ $$$$\mathcal{A}{nd}\:\underset{−} {{for}\:{p}^{\mathrm{2}} =\mathrm{36}} \\ $$$$\:{x}^{\mathrm{2}} \pm\mathrm{6}{x}+\frac{\mathrm{13}}{\mathrm{2}}\mp\frac{\mathrm{3}}{\mathrm{2}}=\mathrm{0} \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} +\mathrm{6}{x}+\mathrm{5}=\mathrm{0}\:\:\:\:\&\:{x}^{\mathrm{2}} −\mathrm{6}{x}+\mathrm{8}=\mathrm{0} \\ $$$$\Rightarrow\:\:{x}=\:−\mathrm{1},\:−\mathrm{5}\:\:\:\:\:\:\&\:\:{x}=\:\mathrm{2},\:\mathrm{4}\: \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_. \\ $$
Commented by TawaTawa last updated on 05/Aug/19
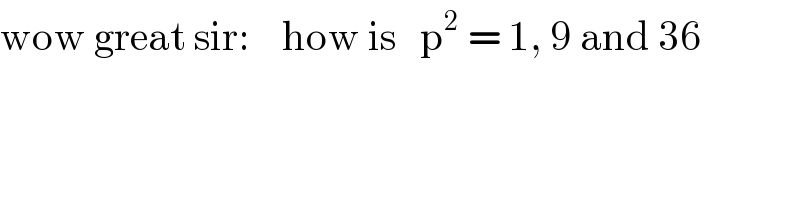
wow great sir: how is p^2 = 1, 9 and 36
$$\mathrm{wow}\:\mathrm{great}\:\mathrm{sir}:\:\:\:\:\mathrm{how}\:\mathrm{is}\:\:\:\mathrm{p}^{\mathrm{2}} \:=\:\mathrm{1},\:\mathrm{9}\:\mathrm{and}\:\mathrm{36} \\ $$

Pin