Menu Close

Prove-that-1-2-3-4-5-6-2005-2006-2007-2008-lt-1-2009-

Tinku Tara 0 Comments
Pin




Question Number 104092 by naka3546 last updated on 19/Jul/20
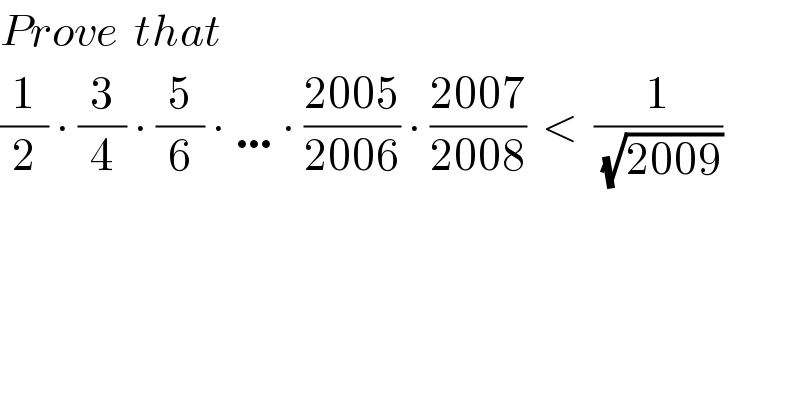
Prove that (1/2) ∙ (3/4) ∙ (5/6) ∙ …∙ ((2005)/(2006)) ∙ ((2007)/(2008)) < (1/( (√(2009))))
$${Prove}\:\:{that}\:\: \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\:\centerdot\:\frac{\mathrm{3}}{\mathrm{4}}\:\centerdot\:\frac{\mathrm{5}}{\mathrm{6}}\:\centerdot\:\ldots\centerdot\:\frac{\mathrm{2005}}{\mathrm{2006}}\:\centerdot\:\frac{\mathrm{2007}}{\mathrm{2008}}\:\:<\:\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2009}}} \\ $$
Commented by JDamian last updated on 19/Jul/20
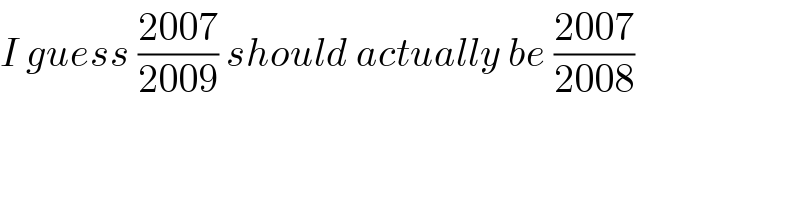
I guess ((2007)/(2009)) should actually be ((2007)/(2008))
$${I}\:{guess}\:\frac{\mathrm{2007}}{\mathrm{2009}}\:{should}\:{actually}\:{be}\:\frac{\mathrm{2007}}{\mathrm{2008}} \\ $$
Answered by 1549442205PVT last updated on 19/Jul/20
It is easy to see that if (a/b)<1 then a<b ⇒ab+a<ab+b⇒a(b+1)<b(a+1)⇒(a/b)<((a+1)/(b+1)). Hence, Putting A=(1/2).(3/4).(5/6)....((2005)/(2006)).((2007)/(2009)).We have: (1/2)<(2/3),(3/4)<(4/5),(5/6)<(6/7)...,((2005)/(2006))<((2006)/(2007)),((2007)/(2009))<((2009)/(2010)) Multiplying 1004 inequlities side by side we getA<(2/3).(4/5).(6/7)....((2006)/(2007)).((2009)/(2010))=B ⇒A^2 <AB=(1/2).(2/3).(3/4).(4/5).(5/6)....((2005)/(2006)).((2006)/(2007)).((2007)/(2009)).((2009)/(2010)) =(1/(2010))⇒A<(1/( (√(2010))))<(1/( (√(2009))))(q.e.d)
$$\mathrm{It}\:\mathrm{is}\:\mathrm{easy}\:\mathrm{to}\:\mathrm{see}\:\mathrm{that}\:\mathrm{if}\:\:\frac{\mathrm{a}}{\mathrm{b}}<\mathrm{1}\:\mathrm{then}\:\mathrm{a}<\mathrm{b} \\ $$$$\Rightarrow\mathrm{ab}+\mathrm{a}<\mathrm{ab}+\mathrm{b}\Rightarrow\mathrm{a}\left(\mathrm{b}+\mathrm{1}\right)<\mathrm{b}\left(\mathrm{a}+\mathrm{1}\right)\Rightarrow\frac{\mathrm{a}}{\mathrm{b}}<\frac{\mathrm{a}+\mathrm{1}}{\mathrm{b}+\mathrm{1}}. \\ $$$$\mathrm{Hence}, \\ $$$$\mathrm{Putting}\:\mathrm{A}=\frac{\mathrm{1}}{\mathrm{2}}.\frac{\mathrm{3}}{\mathrm{4}}.\frac{\mathrm{5}}{\mathrm{6}}….\frac{\mathrm{2005}}{\mathrm{2006}}.\frac{\mathrm{2007}}{\mathrm{2009}}.\mathrm{We}\:\mathrm{have}: \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}<\frac{\mathrm{2}}{\mathrm{3}},\frac{\mathrm{3}}{\mathrm{4}}<\frac{\mathrm{4}}{\mathrm{5}},\frac{\mathrm{5}}{\mathrm{6}}<\frac{\mathrm{6}}{\mathrm{7}}…,\frac{\mathrm{2005}}{\mathrm{2006}}<\frac{\mathrm{2006}}{\mathrm{2007}},\frac{\mathrm{2007}}{\mathrm{2009}}<\frac{\mathrm{2009}}{\mathrm{2010}} \\ $$$$\mathrm{Multiplying}\:\mathrm{1004}\:\mathrm{inequlities}\:\mathrm{side}\:\mathrm{by} \\ $$$$\mathrm{side}\:\mathrm{we}\:\mathrm{getA}<\frac{\mathrm{2}}{\mathrm{3}}.\frac{\mathrm{4}}{\mathrm{5}}.\frac{\mathrm{6}}{\mathrm{7}}….\frac{\mathrm{2006}}{\mathrm{2007}}.\frac{\mathrm{2009}}{\mathrm{2010}}=\mathrm{B} \\ $$$$\Rightarrow\mathrm{A}^{\mathrm{2}} <\mathrm{AB}=\frac{\mathrm{1}}{\mathrm{2}}.\frac{\mathrm{2}}{\mathrm{3}}.\frac{\mathrm{3}}{\mathrm{4}}.\frac{\mathrm{4}}{\mathrm{5}}.\frac{\mathrm{5}}{\mathrm{6}}….\frac{\mathrm{2005}}{\mathrm{2006}}.\frac{\mathrm{2006}}{\mathrm{2007}}.\frac{\mathrm{2007}}{\mathrm{2009}}.\frac{\mathrm{2009}}{\mathrm{2010}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2010}}\Rightarrow\mathrm{A}<\frac{\mathrm{1}}{\:\sqrt{\mathrm{2010}}}<\frac{\mathrm{1}}{\:\sqrt{\mathrm{2009}}}\left(\mathrm{q}.\mathrm{e}.\mathrm{d}\right) \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *