Question Number 38740 by abdo.msup.com last updated on 29/Jun/18

$${find}\:{tbe}\:{polynom}\:{p}\:{withdegre}\:\mathrm{5}\:{wich}\:{verify} \\ $$$${p}\left({x}+\mathrm{1}\right)−{p}\left({x}\right)={x}^{\mathrm{4}} \:\:{and}\:{p}\left(\mathrm{0}\right)=\mathrm{0} \\ $$$${for}\:{that}\:{put}\:{p}\left({x}\right)={ax}^{\mathrm{5}} \:+{bx}^{\mathrm{4}} \:+{cx}^{\mathrm{3}} \:+{dx}^{\mathrm{2}} \\ $$$$+{ex}\:+{f}\:\:{and}\:{find}\:{the}\:{coefficients}. \\ $$$$\left.\mathrm{2}\right)\:{find}\:{interms}\:{of}\:{n}\:{the}\:{value}\:{of} \\ $$$${sum}\:\mathrm{1}\:+\mathrm{2}^{\mathrm{4}} \:+\mathrm{3}^{\mathrm{4}} +….+{n}^{\mathrm{4}} \:. \\ $$
Commented by prakash jain last updated on 29/Jun/18

$$\left({n}+\mathrm{1}\right)^{\mathrm{5}} −{n}^{\mathrm{5}} =\mathrm{5}{n}^{\mathrm{4}} +\mathrm{10}{n}^{\mathrm{3}} +\mathrm{10}{n}^{\mathrm{2}} +\mathrm{5}{n}+\mathrm{1} \\ $$$$\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}\left({i}+\mathrm{1}\right)^{\mathrm{5}} −{i}^{\mathrm{5}} =\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}\mathrm{5}{i}^{\mathrm{4}} +\mathrm{10}{i}^{\mathrm{3}} +\mathrm{10}{i}^{\mathrm{2}} +\mathrm{5}{i}+\mathrm{1} \\ $$$$\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{i}^{\mathrm{4}} =\frac{\mathrm{1}}{\mathrm{5}}\left({n}+\mathrm{1}\right)^{\mathrm{5}} −\frac{{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:−\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{3}}−\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}}−\frac{{n}}{\mathrm{5}} \\ $$
Commented by math khazana by abdo last updated on 29/Jun/18

$${sir}\:{Prakash}\:{are}\:{you}\:{staying}\:{in}\:{india}\:{or}\:{abroad}.. \\ $$
Commented by prakash jain last updated on 30/Jun/18

$$\mathrm{I}\:\mathrm{am}\:\mathrm{in}\:\mathrm{India}. \\ $$
Commented by math khazana by abdo last updated on 30/Jun/18
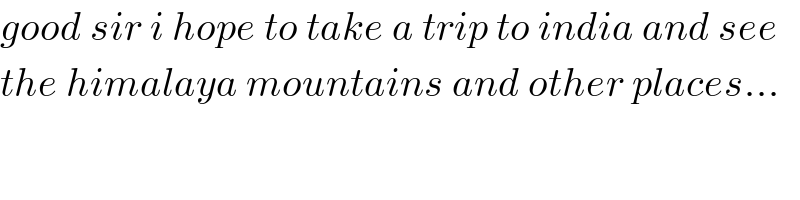
$${good}\:{sir}\:{i}\:{hope}\:{to}\:{take}\:{a}\:{trip}\:{to}\:{india}\:{and}\:{see}\: \\ $$$${the}\:{himalaya}\:{mountains}\:{and}\:{other}\:{places}… \\ $$
Answered by MrW3 last updated on 29/Jun/18
![p(x)=ax^5 +bx^4 +cx^3 +dx^2 +ex+f p(0)=0⇒f=0 p(x+1)=a(x+1)^5 +b(x+1)^4 +c(x+1)^3 +d(x+1)^2 +e(x+1) p(x+1)−p(x)=a[(x+1)^5 −x^5 ]+b[(x+1)^4 −x^4 ]+c[(x+1)^3 −x^3 ]+d[(x+1)^2 −x^2 ]+e=x^4 a[5x^4 +10x^3 +10x^2 +5x+1]+b[4x^3 +6x^2 +4x+1]+c[3x^2 +3x+1]+d[2x+1]+e=x^4 5ax^4 +(10a+4b)x^3 +(10a+6b+3c)x^2 +(5a+4b+3c+2d)x+(a+b+c+d+e)=x^4 ⇒5a=1⇒a=(1/5) ⇒10a+4b=0⇒b=−(5/2)a=−(1/2) ⇒10a+6b+3c=0⇒c=−((10)/3)a−2b=−(1/6)+1=−(5/6) ⇒5a+4b+3c+2d=0⇒d=−(5/2)a−2b−(3/2)c=−(1/2)+1+(5/4)=(7/4) ⇒a+b+c+d+e=0⇒e=−a−b−c−d=−(1/5)+(1/2)+(5/6)−(7/4)=((−12+30+50−85)/(60))=−((17)/(60))](https://www.tinkutara.com/question/Q38774.png)
$${p}\left({x}\right)={ax}^{\mathrm{5}} \:+{bx}^{\mathrm{4}} \:+{cx}^{\mathrm{3}} \:+{dx}^{\mathrm{2}} +{ex}+{f} \\ $$$${p}\left(\mathrm{0}\right)=\mathrm{0}\Rightarrow{f}=\mathrm{0} \\ $$$${p}\left({x}+\mathrm{1}\right)={a}\left({x}+\mathrm{1}\right)^{\mathrm{5}} \:+{b}\left({x}+\mathrm{1}\right)^{\mathrm{4}} \:+{c}\left({x}+\mathrm{1}\right)^{\mathrm{3}} \:+{d}\left({x}+\mathrm{1}\right)^{\mathrm{2}} +{e}\left({x}+\mathrm{1}\right) \\ $$$${p}\left({x}+\mathrm{1}\right)−{p}\left({x}\right)={a}\left[\left({x}+\mathrm{1}\right)^{\mathrm{5}} −{x}^{\mathrm{5}} \right]+{b}\left[\left({x}+\mathrm{1}\right)^{\mathrm{4}} −{x}^{\mathrm{4}} \right]+{c}\left[\left({x}+\mathrm{1}\right)^{\mathrm{3}} −{x}^{\mathrm{3}} \right]+{d}\left[\left({x}+\mathrm{1}\right)^{\mathrm{2}} −{x}^{\mathrm{2}} \right]+{e}={x}^{\mathrm{4}} \\ $$$${a}\left[\mathrm{5}{x}^{\mathrm{4}} +\mathrm{10}{x}^{\mathrm{3}} +\mathrm{10}{x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{1}\right]+{b}\left[\mathrm{4}{x}^{\mathrm{3}} +\mathrm{6}{x}^{\mathrm{2}} +\mathrm{4}{x}+\mathrm{1}\right]+{c}\left[\mathrm{3}{x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{1}\right]+{d}\left[\mathrm{2}{x}+\mathrm{1}\right]+{e}={x}^{\mathrm{4}} \\ $$$$\mathrm{5}{ax}^{\mathrm{4}} +\left(\mathrm{10}{a}+\mathrm{4}{b}\right){x}^{\mathrm{3}} +\left(\mathrm{10}{a}+\mathrm{6}{b}+\mathrm{3}{c}\right){x}^{\mathrm{2}} +\left(\mathrm{5}{a}+\mathrm{4}{b}+\mathrm{3}{c}+\mathrm{2}{d}\right){x}+\left({a}+{b}+{c}+{d}+{e}\right)={x}^{\mathrm{4}} \\ $$$$\Rightarrow\mathrm{5}{a}=\mathrm{1}\Rightarrow{a}=\frac{\mathrm{1}}{\mathrm{5}} \\ $$$$\Rightarrow\mathrm{10}{a}+\mathrm{4}{b}=\mathrm{0}\Rightarrow{b}=−\frac{\mathrm{5}}{\mathrm{2}}{a}=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{10}{a}+\mathrm{6}{b}+\mathrm{3}{c}=\mathrm{0}\Rightarrow{c}=−\frac{\mathrm{10}}{\mathrm{3}}{a}−\mathrm{2}{b}=−\frac{\mathrm{1}}{\mathrm{6}}+\mathrm{1}=−\frac{\mathrm{5}}{\mathrm{6}} \\ $$$$\Rightarrow\mathrm{5}{a}+\mathrm{4}{b}+\mathrm{3}{c}+\mathrm{2}{d}=\mathrm{0}\Rightarrow{d}=−\frac{\mathrm{5}}{\mathrm{2}}{a}−\mathrm{2}{b}−\frac{\mathrm{3}}{\mathrm{2}}{c}=−\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{1}+\frac{\mathrm{5}}{\mathrm{4}}=\frac{\mathrm{7}}{\mathrm{4}} \\ $$$$\Rightarrow{a}+{b}+{c}+{d}+{e}=\mathrm{0}\Rightarrow{e}=−{a}−{b}−{c}−{d}=−\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{5}}{\mathrm{6}}−\frac{\mathrm{7}}{\mathrm{4}}=\frac{−\mathrm{12}+\mathrm{30}+\mathrm{50}−\mathrm{85}}{\mathrm{60}}=−\frac{\mathrm{17}}{\mathrm{60}} \\ $$
Commented by math khazana by abdo last updated on 29/Jun/18

$${thank}\:{you}\:{sir}. \\ $$
