Question Number 3894 by Filup last updated on 24/Dec/15

$$\mathrm{I}\:\mathrm{have}\:{n}\:\mathrm{six}\:\mathrm{sided}\:\mathrm{dice}. \\ $$$$\mathrm{I}\:\mathrm{roll}\:\mathrm{them}\:\mathrm{all}.\:\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{proability} \\ $$$$\mathrm{that}\:{k}\:\mathrm{of}\:\mathrm{them}\:\mathrm{share}\:\mathrm{the}\:\mathrm{same}\:\mathrm{value}? \\ $$
Commented by Filup last updated on 24/Dec/15
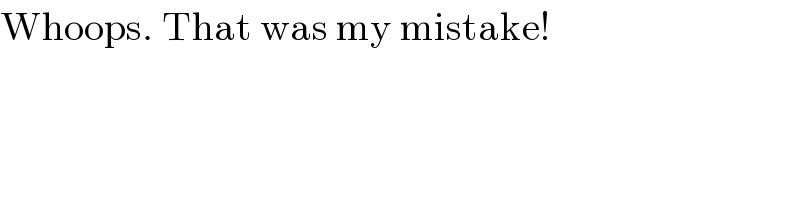
$$\mathrm{Whoops}.\:\mathrm{That}\:\mathrm{was}\:\mathrm{my}\:\mathrm{mistake}! \\ $$
Commented by Filup last updated on 24/Dec/15
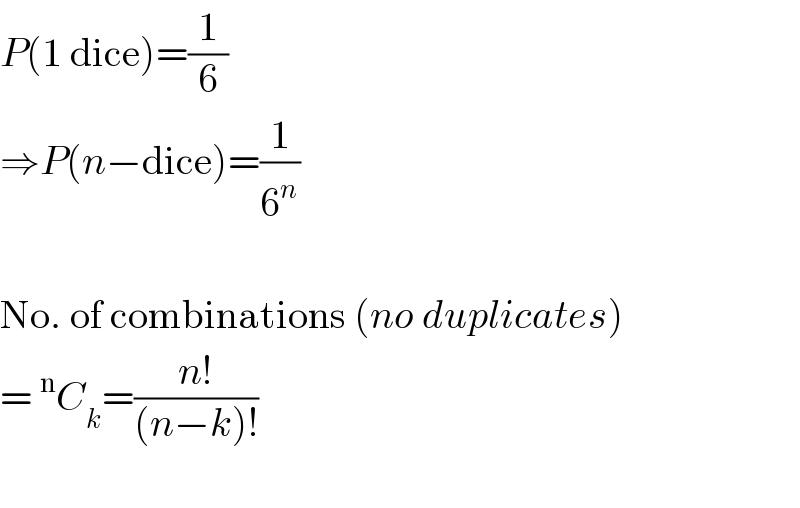
$${P}\left(\mathrm{1}\:\mathrm{dice}\right)=\frac{\mathrm{1}}{\mathrm{6}} \\ $$$$\Rightarrow{P}\left({n}−\mathrm{dice}\right)=\frac{\mathrm{1}}{\mathrm{6}^{{n}} } \\ $$$$ \\ $$$$\mathrm{No}.\:\mathrm{of}\:\mathrm{combinations}\:\left({no}\:{duplicates}\right) \\ $$$$=\:^{\mathrm{n}} {C}_{{k}} =\frac{{n}!}{\left({n}−{k}\right)!} \\ $$$$ \\ $$
Commented by Yozzii last updated on 24/Dec/15
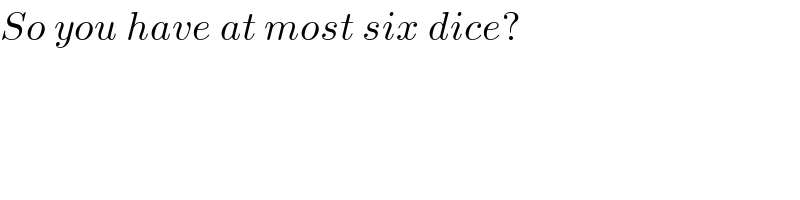
$${So}\:{you}\:{have}\:{at}\:{most}\:{six}\:{dice}?\: \\ $$
Commented by Filup last updated on 24/Dec/15

$$\mathrm{You}\:\mathrm{have}\:{n}\:\mathrm{dice}.\:\mathrm{Each}\:\mathrm{dice}\:\mathrm{has}\:\mathrm{6}\:\mathrm{sides}. \\ $$
Commented by Yozzii last updated on 24/Dec/15
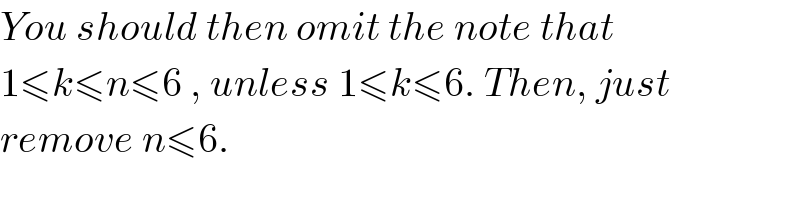
$${You}\:{should}\:{then}\:{omit}\:{the}\:{note}\:{that} \\ $$$$\mathrm{1}\leqslant{k}\leqslant{n}\leqslant\mathrm{6}\:,\:{unless}\:\mathrm{1}\leqslant{k}\leqslant\mathrm{6}.\:{Then},\:{just} \\ $$$${remove}\:{n}\leqslant\mathrm{6}.\: \\ $$
Answered by prakash jain last updated on 24/Dec/15
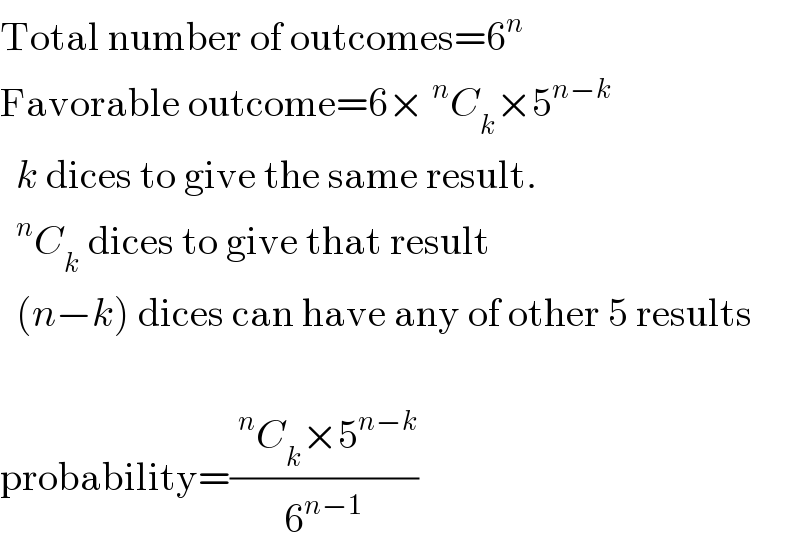
$$\mathrm{Total}\:\mathrm{number}\:\mathrm{of}\:\mathrm{outcomes}=\mathrm{6}^{{n}} \\ $$$$\mathrm{Favorable}\:\mathrm{outcome}=\mathrm{6}×\:^{{n}} {C}_{{k}} ×\mathrm{5}^{{n}−{k}} \\ $$$$\:\:{k}\:\mathrm{dices}\:\mathrm{to}\:\mathrm{give}\:\mathrm{the}\:\mathrm{same}\:\mathrm{result}. \\ $$$$\:\:^{{n}} {C}_{{k}} \:\mathrm{dices}\:\mathrm{to}\:\mathrm{give}\:\mathrm{that}\:\mathrm{result} \\ $$$$\:\:\left({n}−{k}\right)\:\mathrm{dices}\:\mathrm{can}\:\mathrm{have}\:\mathrm{any}\:\mathrm{of}\:\mathrm{other}\:\mathrm{5}\:\mathrm{results} \\ $$$$ \\ $$$$\mathrm{probability}=\frac{\:^{{n}} {C}_{{k}} ×\mathrm{5}^{{n}−{k}} }{\mathrm{6}^{{n}−\mathrm{1}} } \\ $$
Commented by Yozzii last updated on 25/Dec/15
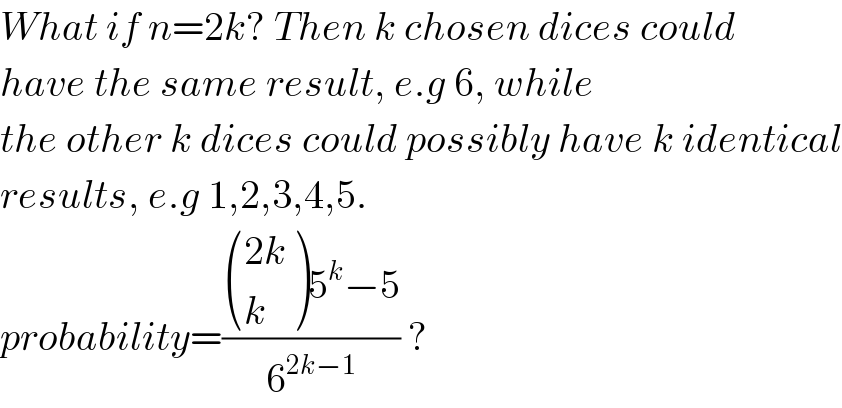
$${What}\:{if}\:{n}=\mathrm{2}{k}?\:{Then}\:{k}\:{chosen}\:{dices}\:{could} \\ $$$${have}\:{the}\:{same}\:{result},\:{e}.{g}\:\mathrm{6},\:{while} \\ $$$${the}\:{other}\:{k}\:{dices}\:{could}\:{possibly}\:{have}\:{k}\:{identical} \\ $$$${results},\:{e}.{g}\:\mathrm{1},\mathrm{2},\mathrm{3},\mathrm{4},\mathrm{5}.\: \\ $$$${probability}=\frac{\begin{pmatrix}{\mathrm{2}{k}}\\{{k}}\end{pmatrix}\mathrm{5}^{{k}} −\mathrm{5}}{\mathrm{6}^{\mathrm{2}{k}−\mathrm{1}} }\:? \\ $$
Commented by prakash jain last updated on 25/Dec/15
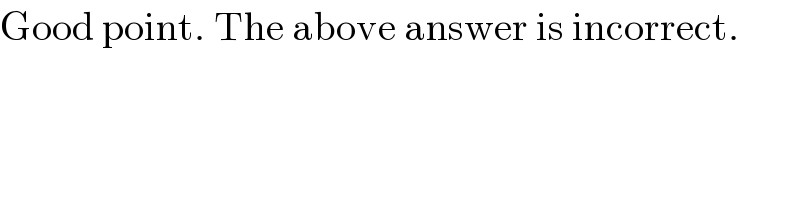
$$\mathrm{Good}\:\mathrm{point}.\:\mathrm{The}\:\mathrm{above}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{incorrect}. \\ $$
Commented by Yozzii last updated on 25/Dec/15
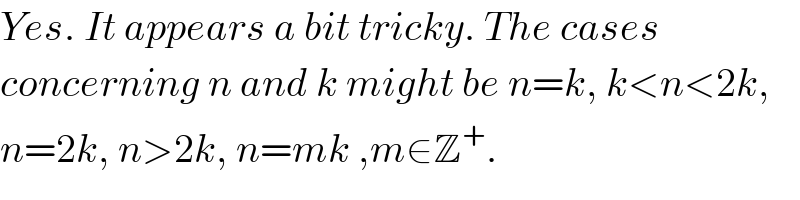
$${Yes}.\:{It}\:{appears}\:{a}\:{bit}\:{tricky}.\:{The}\:{cases} \\ $$$${concerning}\:{n}\:{and}\:{k}\:{might}\:{be}\:{n}={k},\:{k}<{n}<\mathrm{2}{k}, \\ $$$${n}=\mathrm{2}{k},\:{n}>\mathrm{2}{k},\:{n}={mk}\:,{m}\in\mathbb{Z}^{+} .\: \\ $$
Commented by prakash jain last updated on 25/Dec/15

$$\mathrm{I}\:\mathrm{just}\:\mathrm{considerred}\:\mathrm{the}\:\mathrm{case}\:\mathrm{where}\:{n}<\mathrm{2}{k}.\: \\ $$
