Question Number 105383 by bemath last updated on 28/Jul/20
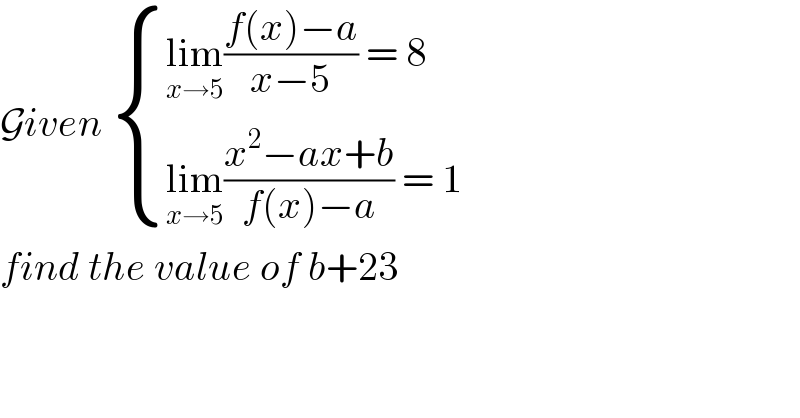
$$\mathcal{G}{iven}\:\begin{cases}{\underset{{x}\rightarrow\mathrm{5}} {\mathrm{lim}}\frac{{f}\left({x}\right)−{a}}{{x}−\mathrm{5}}\:=\:\mathrm{8}}\\{\underset{{x}\rightarrow\mathrm{5}} {\mathrm{lim}}\frac{{x}^{\mathrm{2}} −{ax}+{b}}{{f}\left({x}\right)−{a}}\:=\:\mathrm{1}}\end{cases} \\ $$$${find}\:{the}\:{value}\:{of}\:{b}+\mathrm{23}\: \\ $$
Answered by john santu last updated on 28/Jul/20
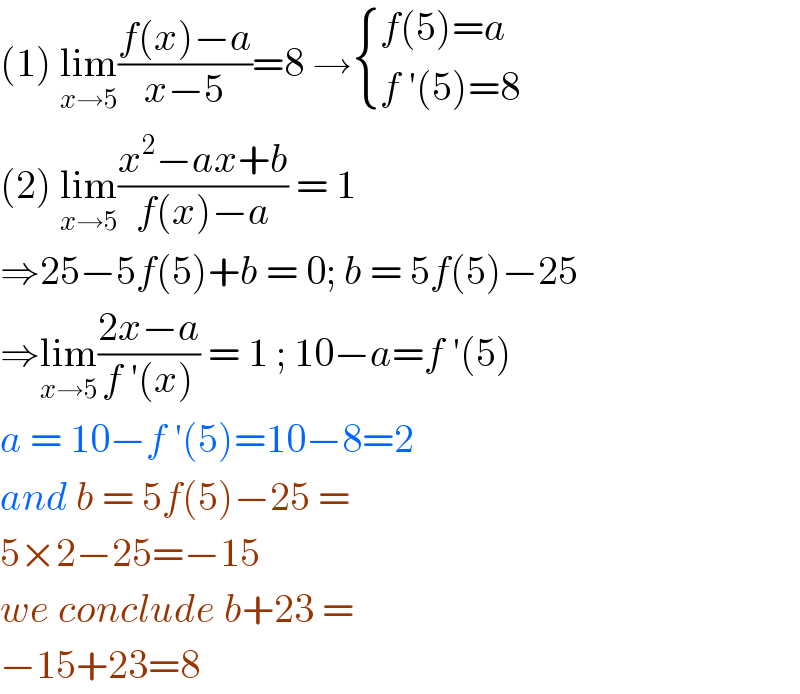
$$\left(\mathrm{1}\right)\:\underset{{x}\rightarrow\mathrm{5}} {\mathrm{lim}}\frac{{f}\left({x}\right)−{a}}{{x}−\mathrm{5}}=\mathrm{8}\:\rightarrow\begin{cases}{{f}\left(\mathrm{5}\right)={a}}\\{{f}\:'\left(\mathrm{5}\right)=\mathrm{8}}\end{cases} \\ $$$$\left(\mathrm{2}\right)\:\underset{{x}\rightarrow\mathrm{5}} {\mathrm{lim}}\frac{{x}^{\mathrm{2}} −{ax}+{b}}{{f}\left({x}\right)−{a}}\:=\:\mathrm{1}\: \\ $$$$\Rightarrow\mathrm{25}−\mathrm{5}{f}\left(\mathrm{5}\right)+{b}\:=\:\mathrm{0};\:{b}\:=\:\mathrm{5}{f}\left(\mathrm{5}\right)−\mathrm{25} \\ $$$$\Rightarrow\underset{{x}\rightarrow\mathrm{5}} {\mathrm{lim}}\frac{\mathrm{2}{x}−{a}}{{f}\:'\left({x}\right)}\:=\:\mathrm{1}\:;\:\mathrm{10}−{a}={f}\:'\left(\mathrm{5}\right) \\ $$$${a}\:=\:\mathrm{10}−{f}\:'\left(\mathrm{5}\right)=\mathrm{10}−\mathrm{8}=\mathrm{2} \\ $$$${and}\:{b}\:=\:\mathrm{5}{f}\left(\mathrm{5}\right)−\mathrm{25}\:= \\ $$$$\mathrm{5}×\mathrm{2}−\mathrm{25}=−\mathrm{15} \\ $$$${we}\:{conclude}\:{b}+\mathrm{23}\:= \\ $$$$−\mathrm{15}+\mathrm{23}=\mathrm{8}\: \\ $$
Commented by bemath last updated on 28/Jul/20

$${jooss} \\ $$
