Menu Close

Question-39993

Tinku Tara 0 Comments
Pin




Question Number 39993 by ajfour last updated on 14/Jul/18
Answered by tanmay.chaudhury50@gmail.com last updated on 14/Jul/18
let ∠AOT=θ OT∥ toBC so ((AO)/(AC))=((AT)/(AB))=((OT)/(BC)) point T(cosθ,sinθ) andpoint C(−1,0) eqn of OT is y=xtanθ eqn ofCB is y=(x+1)tanθ eqn of circle is x^2 +y^2 =1 solve y=(x+1)tanθ and x^2 +y^2 =1 x^2 +x^2 tan^2 θ+2xtan^2 θ+tan^2 θ−1=0 x^2 (sec^2 θ)+x(2tan^2 θ)+tan^2 θ−1=0 x^2 +2xsin^2 θ+sin^2 θ−cos^2 θ=0 x^2 +x(1−cos2θ)−(cos2θ)=0 x=((−(1−cos2θ)±(√(1−2cos2θ+cos^2 2θ+4cos2θ)))/2) x=((−(1−cos2θ)±(1+cos2θ))/2) x=((−1+cos2θ+1+cos2θ)/2),((−1+cos2θ−1−cos2θ)/2) x=cos2θ,−1 when x=cos2θ y=sin2θ when x=−1 so y=0 so point C(−1,0) point P(cos2θ,sin2θ) CP=(√((cos2θ +1)^2 +(sin2θ)^2 )) =(√(co_ s^2 2θ+2cos2θ+1+sin^2 2θ)) =(√(2(1+cos2θ))) =(√(4cos^2 θ))=2cosθ where θ=∠AOT wait...
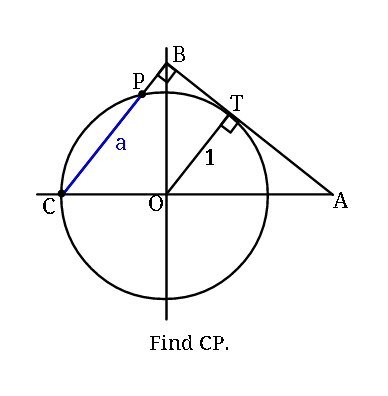
$${let}\:\angle{AOT}=\theta \\ $$$${OT}\parallel\:{toBC}\:{so}\:\frac{{AO}}{{AC}}=\frac{{AT}}{{AB}}=\frac{{OT}}{{BC}} \\ $$$${point}\:{T}\left({cos}\theta,{sin}\theta\right)\:\:{andpoint}\:{C}\left(−\mathrm{1},\mathrm{0}\right) \\ $$$${eqn}\:\:\:{of}\:{OT}\:{is}\:{y}={xtan}\theta \\ $$$${eqn}\:{ofCB}\:{is}\:{y}=\left({x}+\mathrm{1}\right){tan}\theta \\ $$$${eqn}\:{of}\:{circle}\:{is}\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{1} \\ $$$${solve}\:{y}=\left({x}+\mathrm{1}\right){tan}\theta\:{and}\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{1} \\ $$$${x}^{\mathrm{2}} +{x}^{\mathrm{2}} {tan}^{\mathrm{2}} \theta+\mathrm{2}{xtan}^{\mathrm{2}} \theta+{tan}^{\mathrm{2}} \theta−\mathrm{1}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} \left({sec}^{\mathrm{2}} \theta\right)+{x}\left(\mathrm{2}{tan}^{\mathrm{2}} \theta\right)+{tan}^{\mathrm{2}} \theta−\mathrm{1}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} +\mathrm{2}{xsin}^{\mathrm{2}} \theta+{sin}^{\mathrm{2}} \theta−{cos}^{\mathrm{2}} \theta=\mathrm{0} \\ $$$${x}^{\mathrm{2}} +{x}\left(\mathrm{1}−{cos}\mathrm{2}\theta\right)−\left({cos}\mathrm{2}\theta\right)=\mathrm{0} \\ $$$${x}=\frac{−\left(\mathrm{1}−{cos}\mathrm{2}\theta\right)\pm\sqrt{\mathrm{1}−\mathrm{2}{cos}\mathrm{2}\theta+{cos}^{\mathrm{2}} \mathrm{2}\theta+\mathrm{4}{cos}\mathrm{2}\theta}}{\mathrm{2}} \\ $$$${x}=\frac{−\left(\mathrm{1}−{cos}\mathrm{2}\theta\right)\pm\left(\mathrm{1}+{cos}\mathrm{2}\theta\right)}{\mathrm{2}} \\ $$$${x}=\frac{−\mathrm{1}+{cos}\mathrm{2}\theta+\mathrm{1}+{cos}\mathrm{2}\theta}{\mathrm{2}},\frac{−\mathrm{1}+{cos}\mathrm{2}\theta−\mathrm{1}−{cos}\mathrm{2}\theta}{\mathrm{2}} \\ $$$${x}={cos}\mathrm{2}\theta,−\mathrm{1}\: \\ $$$${when}\:{x}={cos}\mathrm{2}\theta\:\:{y}={sin}\mathrm{2}\theta \\ $$$${when}\:{x}=−\mathrm{1}\:\:{so}\:{y}=\mathrm{0} \\ $$$${so}\:{point}\:{C}\left(−\mathrm{1},\mathrm{0}\right)\:{point}\:{P}\left({cos}\mathrm{2}\theta,{sin}\mathrm{2}\theta\right) \\ $$$${CP}=\sqrt{\left({cos}\mathrm{2}\theta\:+\mathrm{1}\right)^{\mathrm{2}} +\left({sin}\mathrm{2}\theta\right)^{\mathrm{2}} } \\ $$$$\:\:=\sqrt{{co}_{} {s}^{\mathrm{2}} \mathrm{2}\theta+\mathrm{2}{cos}\mathrm{2}\theta+\mathrm{1}+{sin}^{\mathrm{2}} \mathrm{2}\theta} \\ $$$$=\sqrt{\mathrm{2}\left(\mathrm{1}+{cos}\mathrm{2}\theta\right)} \\ $$$$=\sqrt{\mathrm{4}{cos}^{\mathrm{2}} \theta}=\mathrm{2}{cos}\theta\:\:{where}\:\theta=\angle{AOT} \\ $$$${wait}… \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Answered by ajfour last updated on 15/Jul/18
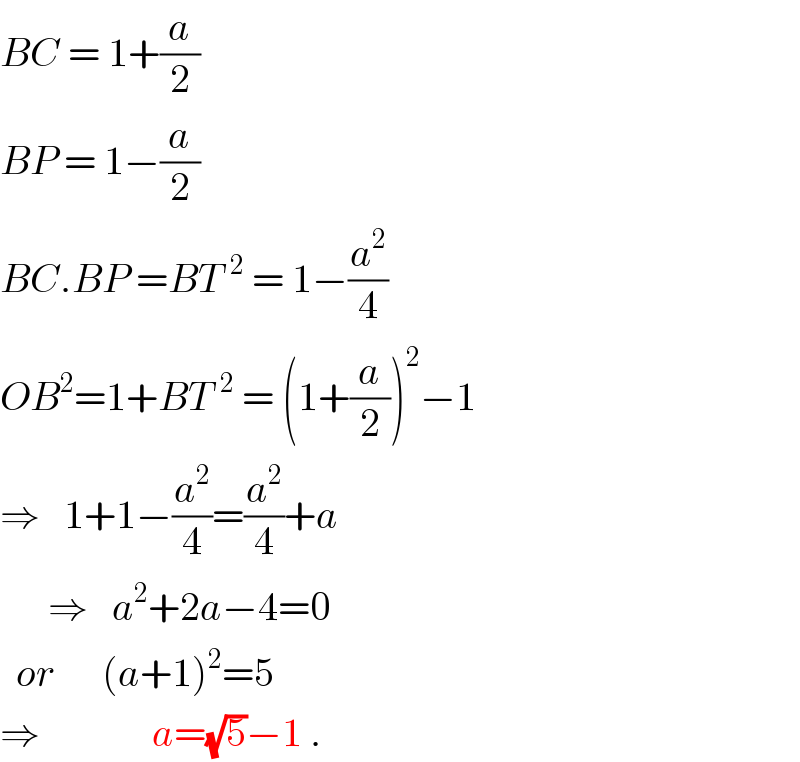
BC = 1+(a/2) BP = 1−(a/2) BC.BP =BT^( 2) = 1−(a^2 /4) OB^2 =1+BT^( 2) = (1+(a/2))^2 −1 ⇒ 1+1−(a^2 /4)=(a^2 /4)+a ⇒ a^2 +2a−4=0 or (a+1)^2 =5 ⇒ a=(√5)−1 .
$${BC}\:=\:\mathrm{1}+\frac{{a}}{\mathrm{2}} \\ $$$${BP}\:=\:\mathrm{1}−\frac{{a}}{\mathrm{2}} \\ $$$${BC}.{BP}\:={BT}^{\:\:\mathrm{2}} \:=\:\mathrm{1}−\frac{{a}^{\mathrm{2}} }{\mathrm{4}} \\ $$$${OB}^{\mathrm{2}} =\mathrm{1}+{BT}^{\:\:\mathrm{2}} \:=\:\left(\mathrm{1}+\frac{{a}}{\mathrm{2}}\right)^{\mathrm{2}} −\mathrm{1} \\ $$$$\Rightarrow\:\:\:\mathrm{1}+\mathrm{1}−\frac{{a}^{\mathrm{2}} }{\mathrm{4}}=\frac{{a}^{\mathrm{2}} }{\mathrm{4}}+{a} \\ $$$$\:\:\:\:\:\:\Rightarrow\:\:\:{a}^{\mathrm{2}} +\mathrm{2}{a}−\mathrm{4}=\mathrm{0} \\ $$$$\:\:{or}\:\:\:\:\:\:\left({a}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{5} \\ $$$$\Rightarrow\:\:\:\:\:\:\:\:\:\:\:\:\:\:{a}=\sqrt{\mathrm{5}}−\mathrm{1}\:. \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 15/Jul/18
hw BC and BP found...pls explain..
$${hw}\:{BC}\:{and}\:{BP}\:{found}…{pls}\:{explain}.. \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 15/Jul/18
yes i got it..perpedicuar from centre bisect the chord...and perpendicular from centre make a rectangle...opposite side are same equals to 1
$${yes}\:{i}\:{got}\:{it}..{perpedicuar}\:{from}\:{centre}\:{bisect}\:{the} \\ $$$${chord}…{and}\:{perpendicular}\:{from}\:{centre} \\ $$$${make}\:{a}\:{rectangle}…{opposite}\:{side}\:{are}\:{same}\:{equals}\: \\ $$$${to}\:\mathrm{1} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *