Menu Close

Solve-dy-dx-x-y-x-y-

Tinku Tara 0 Comments
Pin




Question Number 40380 by rahul 19 last updated on 21/Jul/18
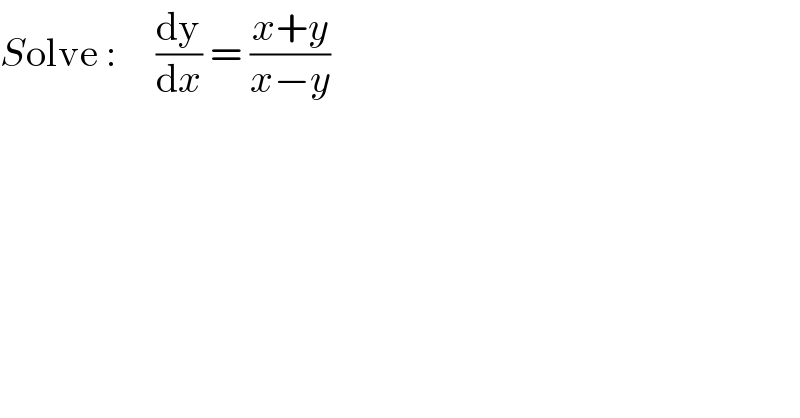
Solve : (dy/dx) = ((x+y)/(x−y))
$${S}\mathrm{olve}\::\:\:\:\:\:\frac{\mathrm{dy}}{\mathrm{d}{x}}\:=\:\frac{{x}+{y}}{{x}−{y}} \\ $$
Commented by rahul 19 last updated on 21/Jul/18
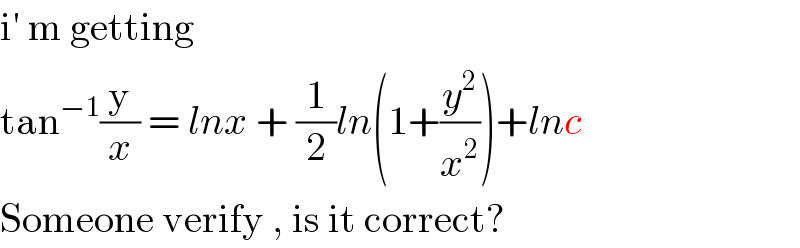
i′ m getting tan^(−1) (y/x) = lnx + (1/2)ln(1+(y^2 /x^2 ))+lnc Someone verify , is it correct?
$$\mathrm{i}'\:\mathrm{m}\:\mathrm{getting}\: \\ $$$$\mathrm{tan}^{−\mathrm{1}} \frac{\mathrm{y}}{{x}}\:=\:{lnx}\:+\:\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{1}+\frac{{y}^{\mathrm{2}} }{{x}^{\mathrm{2}} }\right)+{lnc} \\ $$$$\mathrm{Someone}\:\mathrm{verify}\:,\:\mathrm{is}\:\mathrm{it}\:\mathrm{correct}? \\ $$
Answered by ajfour last updated on 21/Jul/18
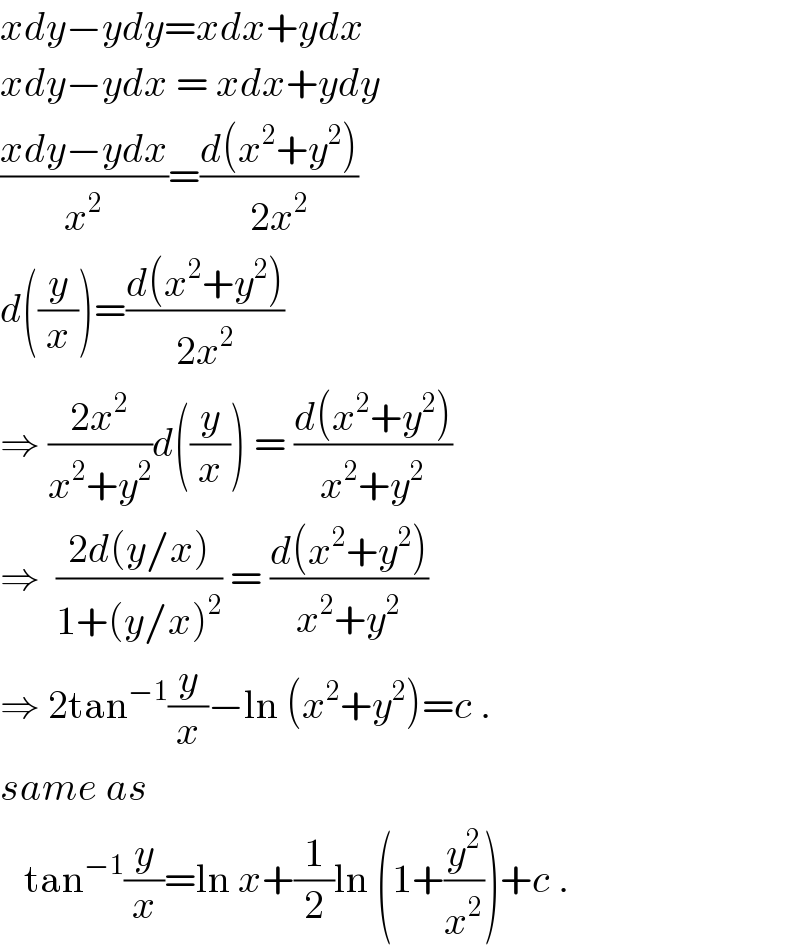
xdy−ydy=xdx+ydx xdy−ydx = xdx+ydy ((xdy−ydx)/x^2 )=((d(x^2 +y^2 ))/(2x^2 )) d((y/x))=((d(x^2 +y^2 ))/(2x^2 )) ⇒ ((2x^2 )/(x^2 +y^2 ))d((y/x)) = ((d(x^2 +y^2 ))/(x^2 +y^2 )) ⇒ ((2d(y/x))/(1+(y/x)^2 )) = ((d(x^2 +y^2 ))/(x^2 +y^2 )) ⇒ 2tan^(−1) (y/x)−ln (x^2 +y^2 )=c . same as tan^(−1) (y/x)=ln x+(1/2)ln (1+(y^2 /x^2 ))+c .
$${xdy}−{ydy}={xdx}+{ydx} \\ $$$${xdy}−{ydx}\:=\:{xdx}+{ydy} \\ $$$$\frac{{xdy}−{ydx}}{{x}^{\mathrm{2}} }=\frac{{d}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)}{\mathrm{2}{x}^{\mathrm{2}} } \\ $$$${d}\left(\frac{{y}}{{x}}\right)=\frac{{d}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)}{\mathrm{2}{x}^{\mathrm{2}} } \\ $$$$\Rightarrow\:\frac{\mathrm{2}{x}^{\mathrm{2}} }{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }{d}\left(\frac{{y}}{{x}}\right)\:=\:\frac{{d}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} } \\ $$$$\Rightarrow\:\:\frac{\mathrm{2}{d}\left({y}/{x}\right)}{\mathrm{1}+\left({y}/{x}\right)^{\mathrm{2}} }\:=\:\frac{{d}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} } \\ $$$$\Rightarrow\:\mathrm{2tan}^{−\mathrm{1}} \frac{{y}}{{x}}−\mathrm{ln}\:\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)={c}\:. \\ $$$${same}\:{as} \\ $$$$\:\:\:\mathrm{tan}^{−\mathrm{1}} \frac{{y}}{{x}}=\mathrm{ln}\:{x}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\mathrm{1}+\frac{{y}^{\mathrm{2}} }{{x}^{\mathrm{2}} }\right)+{c}\:. \\ $$
Commented by rahul 19 last updated on 21/Jul/18
thank you sir ��
Commented by mondodotto@gmail.com last updated on 21/Jul/18
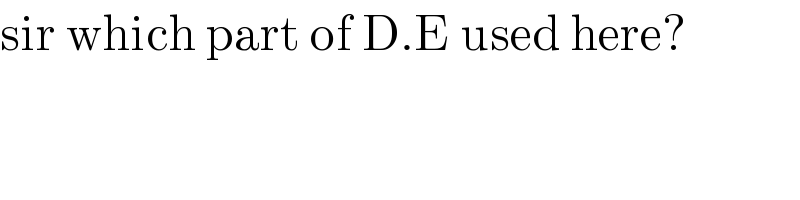
sir which part of D.E used here?
$$\mathrm{sir}\:\mathrm{which}\:\mathrm{part}\:\mathrm{of}\:\mathrm{D}.\mathrm{E}\:\mathrm{used}\:\mathrm{here}? \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 21/Jul/18
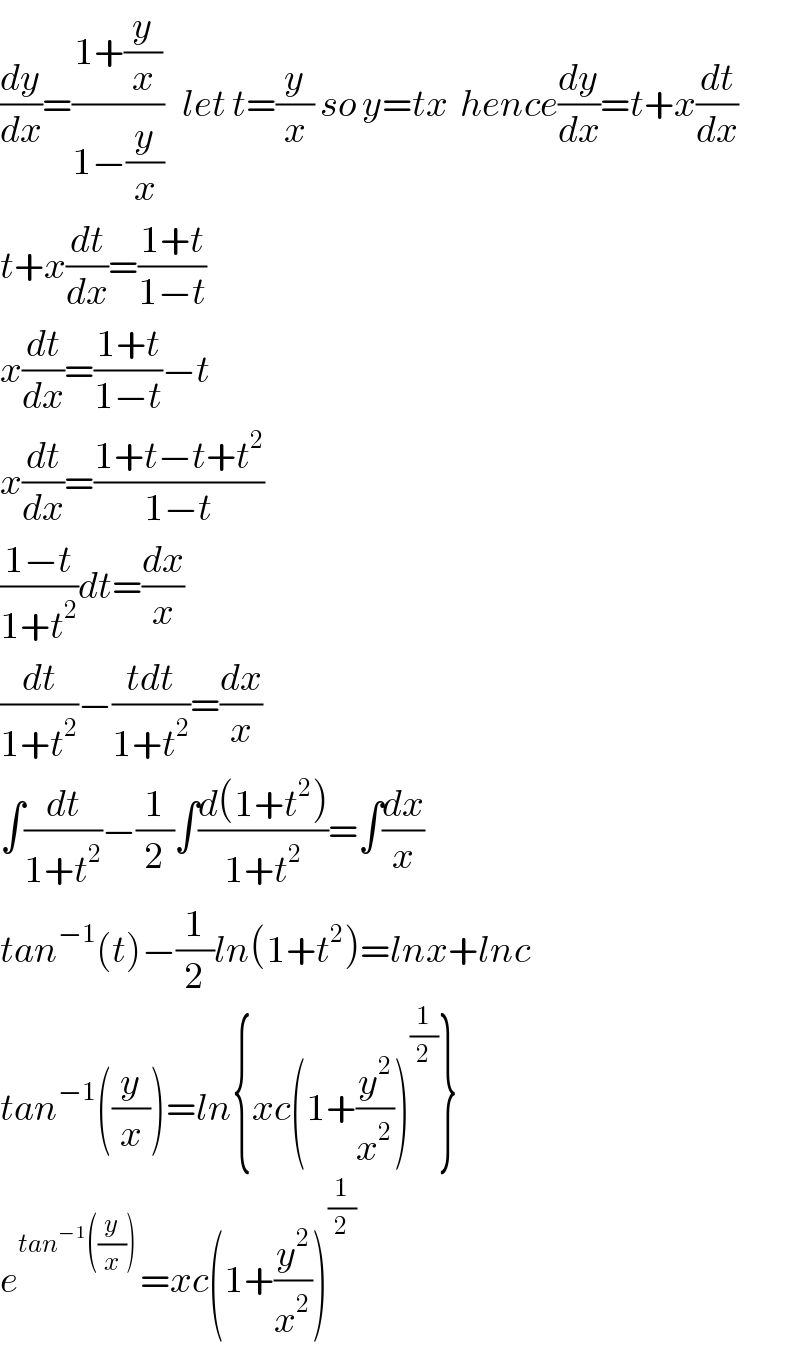
(dy/dx)=((1+(y/x))/(1−(y/x))) let t=(y/x) so y=tx hence(dy/dx)=t+x(dt/dx) t+x(dt/dx)=((1+t)/(1−t)) x(dt/dx)=((1+t)/(1−t))−t x(dt/dx)=((1+t−t+t^2 )/(1−t)) ((1−t)/(1+t^2 ))dt=(dx/x) (dt/(1+t^2 ))−((tdt)/(1+t^2 ))=(dx/x) ∫(dt/(1+t^2 ))−(1/2)∫((d(1+t^2 ))/(1+t^2 ))=∫(dx/x) tan^(−1) (t)−(1/2)ln(1+t^2 )=lnx+lnc tan^(−1) ((y/x))=ln{xc(1+(y^2 /x^2 ))^(1/2) } e^(tan^(−1) ((y/x)) ) =xc(1+(y^2 /x^2 ))^(1/2)
$$\frac{{dy}}{{dx}}=\frac{\mathrm{1}+\frac{{y}}{{x}}}{\mathrm{1}−\frac{{y}}{{x}}}\:\:\:{let}\:{t}=\frac{{y}}{{x}}\:{so}\:{y}={tx}\:\:{hence}\frac{{dy}}{{dx}}={t}+{x}\frac{{dt}}{{dx}} \\ $$$${t}+{x}\frac{{dt}}{{dx}}=\frac{\mathrm{1}+{t}}{\mathrm{1}−{t}} \\ $$$${x}\frac{{dt}}{{dx}}=\frac{\mathrm{1}+{t}}{\mathrm{1}−{t}}−{t} \\ $$$${x}\frac{{dt}}{{dx}}=\frac{\mathrm{1}+{t}−{t}+{t}^{\mathrm{2}} }{\mathrm{1}−{t}} \\ $$$$\frac{\mathrm{1}−{t}}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}=\frac{{dx}}{{x}} \\ $$$$\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }−\frac{{tdt}}{\mathrm{1}+{t}^{\mathrm{2}} }=\frac{{dx}}{{x}} \\ $$$$\int\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{d}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }=\int\frac{{dx}}{{x}} \\ $$$${tan}^{−\mathrm{1}} \left({t}\right)−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)={lnx}+{lnc} \\ $$$${tan}^{−\mathrm{1}} \left(\frac{{y}}{{x}}\right)={ln}\left\{{xc}\left(\mathrm{1}+\frac{{y}^{\mathrm{2}} }{{x}^{\mathrm{2}} }\right)^{\frac{\mathrm{1}}{\mathrm{2}}} \right\} \\ $$$${e}^{{tan}^{−\mathrm{1}} \left(\frac{{y}}{{x}}\right)\:} ={xc}\left(\mathrm{1}+\frac{{y}^{\mathrm{2}} }{{x}^{\mathrm{2}} }\right)^{\frac{\mathrm{1}}{\mathrm{2}}} \\ $$
Commented by rahul 19 last updated on 21/Jul/18
thank you sir ��

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *