Question Number 40711 by Necxx last updated on 26/Jul/18

$${Two}\:{point}\:{charges},{q}_{\mathrm{1}} =\mathrm{0}.\mathrm{4}\mu{C}\:{and} \\ $$$${q}_{\mathrm{2}} =−\mathrm{0}.\mathrm{3}\mu{C}\:{are}\:{placed}\:{at}\:\mathrm{10}{cm} \\ $$$${apart}.{Calculate}\: \\ $$$$\left({a}\right){the}\:{potential}\:{at}\:{point}\:{A}\:{which}\:{is} \\ $$$${midway}\:{between}\:{them},{and} \\ $$$$\left({b}\right){point}\:{B}\:{which}\:{is}\:\mathrm{6}{cm}\:{from}\:{q}_{\mathrm{1}} \\ $$$${and}\:\mathrm{8}{cm}\:{from}\:{q}_{\mathrm{2}} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 26/Jul/18
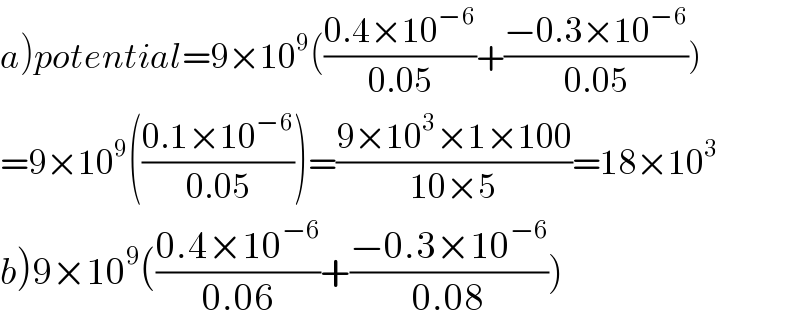
$$\left.{a}\right){potential}=\mathrm{9}×\mathrm{10}^{\mathrm{9}} \left(\frac{\mathrm{0}.\mathrm{4}×\mathrm{10}^{−\mathrm{6}} }{\mathrm{0}.\mathrm{05}}+\frac{−\mathrm{0}.\mathrm{3}×\mathrm{10}^{−\mathrm{6}} }{\mathrm{0}.\mathrm{05}}\right) \\ $$$$=\mathrm{9}×\mathrm{10}^{\mathrm{9}} \left(\frac{\mathrm{0}.\mathrm{1}×\mathrm{10}^{−\mathrm{6}} }{\mathrm{0}.\mathrm{05}}\right)=\frac{\mathrm{9}×\mathrm{10}^{\mathrm{3}} ×\mathrm{1}×\mathrm{100}}{\mathrm{10}×\mathrm{5}}=\mathrm{18}×\mathrm{10}^{\mathrm{3}} \\ $$$$\left.{b}\right)\mathrm{9}×\mathrm{10}^{\mathrm{9}} \left(\frac{\mathrm{0}.\mathrm{4}×\mathrm{10}^{−\mathrm{6}} }{\mathrm{0}.\mathrm{06}}+\frac{−\mathrm{0}.\mathrm{3}×\mathrm{10}^{−\mathrm{6}} }{\mathrm{0}.\mathrm{08}}\right) \\ $$
