Menu Close

Question-41038

Tinku Tara 0 Comments
Pin




Question Number 41038 by Tawa1 last updated on 31/Jul/18
Commented by tanmay.chaudhury50@gmail.com last updated on 31/Jul/18
about question number 25) there is eight corner...at each corner three face merge...number at any corner share by three face..target to find sum of four number such that each face give same value...the sumtotal can not devide 45... attaching a paper cut...when join make a cube each corner mark by alphbet...every feace with proper mark attached... trying to reach the goal...
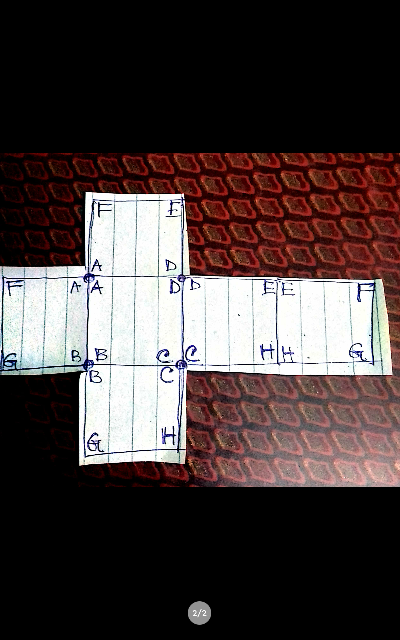
$$\left.{about}\:{question}\:{number}\:\mathrm{25}\right) \\ $$$${there}\:{is}\:{eight}\:{corner}…{at}\:{each}\:{corner}\:{three}\:{face} \\ $$$${merge}…{number}\:{at}\:{any}\:{corner}\:{share}\:{by}\:{three} \\ $$$${face}..{target}\:{to}\:{find}\:{sum}\:{of}\:{four}\:{number}\:{such} \\ $$$${that}\:{each}\:{face}\:{give}\:{same}\:{value}…{the}\:{sumtotal} \\ $$$${can}\:{not}\:{devide}\:\mathrm{45}… \\ $$$${attaching}\:{a}\:{paper}\:{cut}…{when}\:{join}\:{make}\:{a}\:{cube} \\ $$$${each}\:{corner}\:{mark}\:{by}\:{alphbet}…{every}\:{feace}\:{with} \\ $$$${proper}\:{mark}\:{attached}… \\ $$$${trying}\:{to}\:{reach}\:{the}\:{goal}… \\ $$$$ \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 31/Jul/18
Commented by Tawa1 last updated on 31/Jul/18
Please what will be the final answer. God bless you. thanks for your help
$$\mathrm{Please}\:\mathrm{what}\:\mathrm{will}\:\mathrm{be}\:\mathrm{the}\:\mathrm{final}\:\mathrm{answer}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}.\:\mathrm{thanks}\:\mathrm{for}\:\mathrm{your}\:\mathrm{help} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 31/Jul/18
6+5+3+1=15 this cobination not allow 8+4+2+1 not allowed 7+5+2+1 not allowed 9+3+2+1not allowed 6+5+3+1 not allowrd 7+4+3+1 not allowed 7+5+2+1 not allowed A= B= C= D= face value= A= D= E= F= A= B= F= G= B= C= G= H= A= B= C= D= E= F= G= H= observing the combination of number whose sumtotal is 15...it is observed that every time one number is appearing and that is 1 it seems that the required number is 1 ....leg me try more to find the face value and combination...
$$\mathrm{6}+\mathrm{5}+\mathrm{3}+\mathrm{1}=\mathrm{15}\:{this}\:{cobination}\:{not}\:{allow} \\ $$$$\mathrm{8}+\mathrm{4}+\mathrm{2}+\mathrm{1}\:\:{not}\:{allowed} \\ $$$$\mathrm{7}+\mathrm{5}+\mathrm{2}+\mathrm{1}\:{not}\:{allowed} \\ $$$$\mathrm{9}+\mathrm{3}+\mathrm{2}+\mathrm{1}{not}\:{allowed} \\ $$$$\mathrm{6}+\mathrm{5}+\mathrm{3}+\mathrm{1}\:{not}\:{allowrd} \\ $$$$\mathrm{7}+\mathrm{4}+\mathrm{3}+\mathrm{1}\:{not}\:{allowed} \\ $$$$\mathrm{7}+\mathrm{5}+\mathrm{2}+\mathrm{1}\:{not}\:{allowed} \\ $$$$ \\ $$$${A}=\:\:\:{B}=\:\:\:\:{C}=\:\:\:{D}=\:\:{face}\:{value}= \\ $$$${A}=\:\:\:\:{D}=\:\:\:{E}=\:\:{F}= \\ $$$${A}=\:\:\:{B}=\:\:\:{F}=\:\:\:{G}= \\ $$$${B}=\:{C}=\:\:{G}=\:\:{H}= \\ $$$${A}= \\ $$$${B}= \\ $$$${C}= \\ $$$${D}= \\ $$$${E}= \\ $$$${F}= \\ $$$${G}= \\ $$$${H}= \\ $$$${observing}\:{the}\:{combination}\:{of}\:{number}\:{whose} \\ $$$${sumtotal}\:{is}\:\mathrm{15}…{it}\:{is}\:{observed}\:{that}\:{every}\:{time} \\ $$$${one}\:{number}\:{is}\:{appearing}\:{and}\:{that}\:{is}\:\mathrm{1}\: \\ $$$${it}\:{seems}\:{that}\:{the}\:{required}\:{number}\:{is}\:\mathrm{1} \\ $$$$….{leg}\:{me}\:{try}\:{more}\:{to}\:{find}\:{the}\:{face}\:{value}\:{and} \\ $$$${combination}… \\ $$$$ \\ $$
Commented by Tawa1 last updated on 31/Jul/18
Waiting sir. Thanks for your time. God bless you
$$\mathrm{Waiting}\:\mathrm{sir}.\:\mathrm{Thanks}\:\mathrm{for}\:\mathrm{your}\:\mathrm{time}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you} \\ $$
Answered by MJS last updated on 01/Aug/18
qu. 25 sum on 1 face=s sum on 6 faces=6s used numbers: a b c d e f g h each number counts on 3 faces 3(a+b+c+d+e+f+g+h)=6s a+b+c+d+e+f+g+h=2s ⇒ sum of used numbers is even 1+2+3+4+5+6+7+8+9=45 ⇒ left−off number must be uneven 45−1=44 ⇒ s=22 possible faces 2389 2479 2569 2578 3469 3478 3568 4567 possible combinations of opposite faces 2389 4567 2479 3568 2569 3478 2578 3469 possible cubes determinant (((2839)),((5746))) determinant (((2839)),((7564))) 45−3=42 ⇒ s=21 possible faces 1479 1569 1578 2469 2478 2568 possible combinations of opposite faces 1479 2568 1569 2478 1578 2469 possible cube determinant (((1749)),((5826))) 45−5=40 ⇒ s=20 possible faces 1289 1379 1469 1478 2369 2378 2468 3467 possible combinations of opposite faces 1289 3467 1379 2468 1469 2378 1478 2369 possible cubes determinant (((1829)),((4736))) determinant (((1829)),((7463))) 45−7=38 ⇒ s=19 possible faces 1369 1459 1468 2359 2368 2458 possible combinations of opposite faces 1369 2458 1459 2368 1468 2359 possible cube determinant (((1639)),((4825))) 45−9=36 ⇒ s=18 possible faces 1278 1368 1458 1467 2358 2367 2457 3456 possible combinations of opposite faces 1278 3456 1368 2457 1458 2367 1467 2358 possible cubes determinant (((1728)),((4635))) determinant (((1728)),((6453))) ⇒ leave off any uneven number and you can form a cube with a face−sum not dividing 45 the question is over−defined
$${qu}.\:\mathrm{25} \\ $$$$\mathrm{sum}\:\mathrm{on}\:\mathrm{1}\:\mathrm{face}={s} \\ $$$$\mathrm{sum}\:\mathrm{on}\:\mathrm{6}\:\mathrm{faces}=\mathrm{6}{s} \\ $$$$\mathrm{used}\:\mathrm{numbers}:\:{a}\:{b}\:{c}\:{d}\:{e}\:{f}\:{g}\:{h} \\ $$$$\mathrm{each}\:\mathrm{number}\:\mathrm{counts}\:\mathrm{on}\:\mathrm{3}\:\mathrm{faces} \\ $$$$\mathrm{3}\left({a}+{b}+{c}+{d}+{e}+{f}+{g}+{h}\right)=\mathrm{6}{s} \\ $$$${a}+{b}+{c}+{d}+{e}+{f}+{g}+{h}=\mathrm{2}{s} \\ $$$$\Rightarrow\:\mathrm{sum}\:\mathrm{of}\:\mathrm{used}\:\mathrm{numbers}\:\mathrm{is}\:\mathrm{even} \\ $$$$\mathrm{1}+\mathrm{2}+\mathrm{3}+\mathrm{4}+\mathrm{5}+\mathrm{6}+\mathrm{7}+\mathrm{8}+\mathrm{9}=\mathrm{45} \\ $$$$\Rightarrow\:\mathrm{left}−\mathrm{off}\:\mathrm{number}\:\mathrm{must}\:\mathrm{be}\:\mathrm{uneven} \\ $$$$ \\ $$$$\mathrm{45}−\mathrm{1}=\mathrm{44}\:\Rightarrow\:{s}=\mathrm{22} \\ $$$$\mathrm{possible}\:\mathrm{faces} \\ $$$$\mathrm{2389}\:\:\mathrm{2479}\:\:\mathrm{2569}\:\:\mathrm{2578}\:\:\mathrm{3469}\:\:\mathrm{3478}\:\:\mathrm{3568}\:\:\mathrm{4567} \\ $$$$\mathrm{possible}\:\mathrm{combinations}\:\mathrm{of}\:\mathrm{opposite}\:\mathrm{faces} \\ $$$$\mathrm{2389}\:\:\mathrm{4567} \\ $$$$\mathrm{2479}\:\:\mathrm{3568} \\ $$$$\mathrm{2569}\:\:\mathrm{3478} \\ $$$$\mathrm{2578}\:\:\mathrm{3469} \\ $$$$\mathrm{possible}\:\mathrm{cubes}\:\begin{vmatrix}{\mathrm{2839}}\\{\mathrm{5746}}\end{vmatrix}\:\begin{vmatrix}{\mathrm{2839}}\\{\mathrm{7564}}\end{vmatrix} \\ $$$$ \\ $$$$\mathrm{45}−\mathrm{3}=\mathrm{42}\:\Rightarrow\:{s}=\mathrm{21} \\ $$$$\mathrm{possible}\:\mathrm{faces} \\ $$$$\mathrm{1479}\:\:\mathrm{1569}\:\:\mathrm{1578}\:\:\mathrm{2469}\:\:\mathrm{2478}\:\:\mathrm{2568} \\ $$$$\mathrm{possible}\:\mathrm{combinations}\:\mathrm{of}\:\mathrm{opposite}\:\mathrm{faces} \\ $$$$\mathrm{1479}\:\:\mathrm{2568} \\ $$$$\mathrm{1569}\:\:\mathrm{2478} \\ $$$$\mathrm{1578}\:\:\mathrm{2469} \\ $$$$\mathrm{possible}\:\mathrm{cube}\:\begin{vmatrix}{\mathrm{1749}}\\{\mathrm{5826}}\end{vmatrix}\: \\ $$$$ \\ $$$$\mathrm{45}−\mathrm{5}=\mathrm{40}\:\Rightarrow\:{s}=\mathrm{20} \\ $$$$\mathrm{possible}\:\mathrm{faces} \\ $$$$\mathrm{1289}\:\:\mathrm{1379}\:\:\mathrm{1469}\:\:\mathrm{1478}\:\:\mathrm{2369}\:\:\mathrm{2378}\:\:\mathrm{2468}\:\:\mathrm{3467} \\ $$$$\mathrm{possible}\:\mathrm{combinations}\:\mathrm{of}\:\mathrm{opposite}\:\mathrm{faces} \\ $$$$\mathrm{1289}\:\:\mathrm{3467} \\ $$$$\mathrm{1379}\:\:\mathrm{2468} \\ $$$$\mathrm{1469}\:\:\mathrm{2378} \\ $$$$\mathrm{1478}\:\:\mathrm{2369} \\ $$$$\mathrm{possible}\:\mathrm{cubes}\:\begin{vmatrix}{\mathrm{1829}}\\{\mathrm{4736}}\end{vmatrix}\:\begin{vmatrix}{\mathrm{1829}}\\{\mathrm{7463}}\end{vmatrix} \\ $$$$ \\ $$$$\mathrm{45}−\mathrm{7}=\mathrm{38}\:\Rightarrow\:{s}=\mathrm{19} \\ $$$$\mathrm{possible}\:\mathrm{faces} \\ $$$$\mathrm{1369}\:\:\mathrm{1459}\:\:\mathrm{1468}\:\:\mathrm{2359}\:\:\mathrm{2368}\:\:\mathrm{2458} \\ $$$$\mathrm{possible}\:\mathrm{combinations}\:\mathrm{of}\:\mathrm{opposite}\:\mathrm{faces} \\ $$$$\mathrm{1369}\:\:\mathrm{2458} \\ $$$$\mathrm{1459}\:\:\mathrm{2368} \\ $$$$\mathrm{1468}\:\:\mathrm{2359} \\ $$$$\mathrm{possible}\:\mathrm{cube}\:\begin{vmatrix}{\mathrm{1639}}\\{\mathrm{4825}}\end{vmatrix} \\ $$$$ \\ $$$$\mathrm{45}−\mathrm{9}=\mathrm{36}\:\Rightarrow\:{s}=\mathrm{18} \\ $$$$\mathrm{possible}\:\mathrm{faces} \\ $$$$\mathrm{1278}\:\:\mathrm{1368}\:\:\mathrm{1458}\:\:\mathrm{1467}\:\:\mathrm{2358}\:\:\mathrm{2367}\:\:\mathrm{2457}\:\:\mathrm{3456} \\ $$$$\mathrm{possible}\:\mathrm{combinations}\:\mathrm{of}\:\mathrm{opposite}\:\mathrm{faces} \\ $$$$\mathrm{1278}\:\:\mathrm{3456} \\ $$$$\mathrm{1368}\:\:\mathrm{2457} \\ $$$$\mathrm{1458}\:\:\mathrm{2367} \\ $$$$\mathrm{1467}\:\:\mathrm{2358} \\ $$$$\mathrm{possible}\:\mathrm{cubes}\:\begin{vmatrix}{\mathrm{1728}}\\{\mathrm{4635}}\end{vmatrix}\:\begin{vmatrix}{\mathrm{1728}}\\{\mathrm{6453}}\end{vmatrix} \\ $$$$ \\ $$$$\Rightarrow\:\mathrm{leave}\:\mathrm{off}\:\mathrm{any}\:\mathrm{uneven}\:\mathrm{number}\:\mathrm{and}\:\mathrm{you}\:\mathrm{can} \\ $$$$\mathrm{form}\:\mathrm{a}\:\mathrm{cube}\:\mathrm{with}\:\mathrm{a}\:\mathrm{face}−\mathrm{sum}\:\mathrm{not}\:\mathrm{dividing}\:\mathrm{45} \\ $$$$\mathrm{the}\:\mathrm{question}\:\mathrm{is}\:\mathrm{over}−\mathrm{defined} \\ $$
Commented by Tawa1 last updated on 01/Aug/18
God bless you sir
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *