Menu Close

Question-41233

Tinku Tara 0 Comments
Pin




Question Number 41233 by ajfour last updated on 03/Aug/18
Commented by ajfour last updated on 03/Aug/18
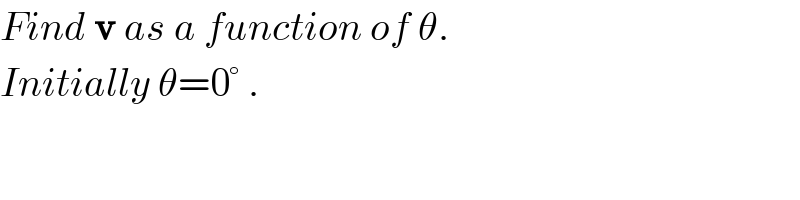
Find v as a function of θ. Initially θ=0° .
$${Find}\:\boldsymbol{\mathrm{v}}\:{as}\:{a}\:{function}\:{of}\:\theta. \\ $$$${Initially}\:\theta=\mathrm{0}°\:. \\ $$
Answered by MrW3 last updated on 04/Aug/18
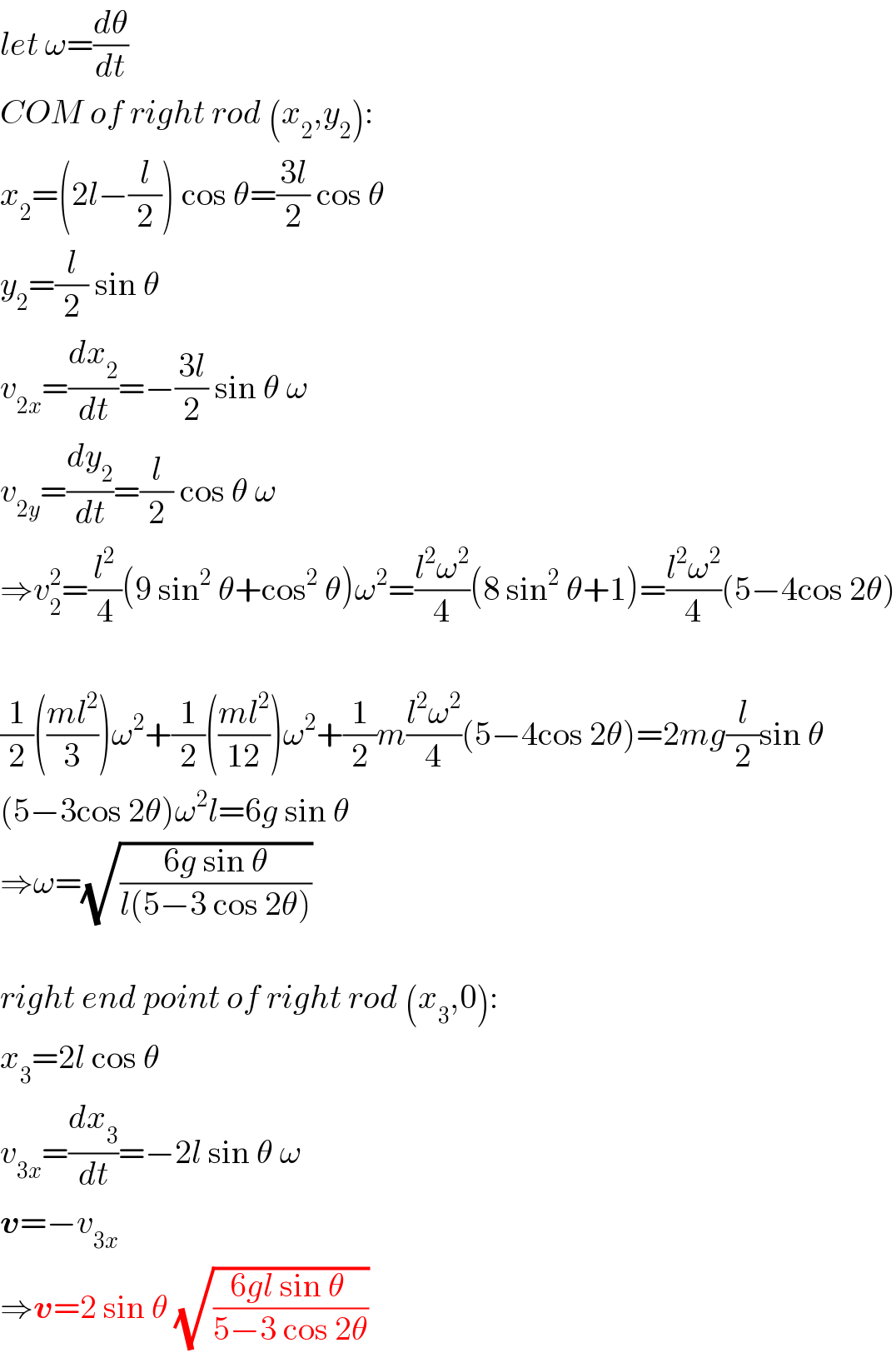
let ω=(dθ/dt) COM of right rod (x_2 ,y_2 ): x_2 =(2l−(l/2)) cos θ=((3l)/2) cos θ y_2 =(l/2) sin θ v_(2x) =(dx_2 /dt)=−((3l)/2) sin θ ω v_(2y) =(dy_2 /dt)=(l/2) cos θ ω ⇒v_2 ^2 =(l^2 /4)(9 sin^2 θ+cos^2 θ)ω^2 =((l^2 ω^2 )/4)(8 sin^2 θ+1)=((l^2 ω^2 )/4)(5−4cos 2θ) (1/2)(((ml^2 )/3))ω^2 +(1/2)(((ml^2 )/(12)))ω^2 +(1/2)m((l^2 ω^2 )/4)(5−4cos 2θ)=2mg(l/2)sin θ (5−3cos 2θ)ω^2 l=6g sin θ ⇒ω=(√((6g sin θ)/(l(5−3 cos 2θ)))) right end point of right rod (x_3 ,0): x_3 =2l cos θ v_(3x) =(dx_3 /dt)=−2l sin θ ω v=−v_(3x) ⇒v=2 sin θ (√((6gl sin θ )/(5−3 cos 2θ)))
$${let}\:\omega=\frac{{d}\theta}{{dt}} \\ $$$${COM}\:{of}\:{right}\:{rod}\:\left({x}_{\mathrm{2}} ,{y}_{\mathrm{2}} \right): \\ $$$${x}_{\mathrm{2}} =\left(\mathrm{2}{l}−\frac{{l}}{\mathrm{2}}\right)\:\mathrm{cos}\:\theta=\frac{\mathrm{3}{l}}{\mathrm{2}}\:\mathrm{cos}\:\theta \\ $$$${y}_{\mathrm{2}} =\frac{{l}}{\mathrm{2}}\:\mathrm{sin}\:\theta \\ $$$${v}_{\mathrm{2}{x}} =\frac{{dx}_{\mathrm{2}} }{{dt}}=−\frac{\mathrm{3}{l}}{\mathrm{2}}\:\mathrm{sin}\:\theta\:\omega \\ $$$${v}_{\mathrm{2}{y}} =\frac{{dy}_{\mathrm{2}} }{{dt}}=\frac{{l}}{\mathrm{2}}\:\mathrm{cos}\:\theta\:\omega \\ $$$$\Rightarrow{v}_{\mathrm{2}} ^{\mathrm{2}} =\frac{{l}^{\mathrm{2}} }{\mathrm{4}}\left(\mathrm{9}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{cos}^{\mathrm{2}} \:\theta\right)\omega^{\mathrm{2}} =\frac{{l}^{\mathrm{2}} \omega^{\mathrm{2}} }{\mathrm{4}}\left(\mathrm{8}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right)=\frac{{l}^{\mathrm{2}} \omega^{\mathrm{2}} }{\mathrm{4}}\left(\mathrm{5}−\mathrm{4cos}\:\mathrm{2}\theta\right) \\ $$$$ \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{ml}^{\mathrm{2}} }{\mathrm{3}}\right)\omega^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{ml}^{\mathrm{2}} }{\mathrm{12}}\right)\omega^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}{m}\frac{{l}^{\mathrm{2}} \omega^{\mathrm{2}} }{\mathrm{4}}\left(\mathrm{5}−\mathrm{4cos}\:\mathrm{2}\theta\right)=\mathrm{2}{mg}\frac{{l}}{\mathrm{2}}\mathrm{sin}\:\theta \\ $$$$\left(\mathrm{5}−\mathrm{3cos}\:\mathrm{2}\theta\right)\omega^{\mathrm{2}} {l}=\mathrm{6}{g}\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow\omega=\sqrt{\frac{\mathrm{6}{g}\:\mathrm{sin}\:\theta}{{l}\left(\mathrm{5}−\mathrm{3}\:\mathrm{cos}\:\mathrm{2}\theta\right)}} \\ $$$$ \\ $$$${right}\:{end}\:{point}\:{of}\:{right}\:{rod}\:\left({x}_{\mathrm{3}} ,\mathrm{0}\right): \\ $$$${x}_{\mathrm{3}} =\mathrm{2}{l}\:\mathrm{cos}\:\theta \\ $$$${v}_{\mathrm{3}{x}} =\frac{{dx}_{\mathrm{3}} }{{dt}}=−\mathrm{2}{l}\:\mathrm{sin}\:\theta\:\omega \\ $$$$\boldsymbol{{v}}=−{v}_{\mathrm{3}{x}} \\ $$$$\Rightarrow\boldsymbol{{v}}=\mathrm{2}\:\mathrm{sin}\:\theta\:\sqrt{\frac{\mathrm{6}{gl}\:\mathrm{sin}\:\theta\:}{\mathrm{5}−\mathrm{3}\:\mathrm{cos}\:\mathrm{2}\theta}} \\ $$
Commented by ajfour last updated on 04/Aug/18
Excellent Sir, thanks a lot.
$${Excellent}\:{Sir},\:{thanks}\:{a}\:{lot}. \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 04/Aug/18
excellent...
$${excellent}… \\ $$
Commented by MrW3 last updated on 04/Aug/18
thank you both!
$${thank}\:{you}\:{both}! \\ $$
Answered by MrW3 last updated on 05/Aug/18
Commented by MrW3 last updated on 06/Aug/18
An other way: BC=2l sin θ CM^2 =BC^2 +BM^2 −2 BC×BM cos (90°−θ) =4l^2 sin^2 θ+(l^2 /4)−2×2l sin θ×(l/2)×sin θ =(l^2 /4)(8 sin^2 θ+1) I_c =((ml^2 )/(12))+m×(l^2 /4)(8 sin^2 θ+1) =((ml^2 )/3)(1+6sin^2 θ) (1/2)(((ml^2 )/3))ω^2 +(1/2)×((ml^2 )/3)(1+6 sin^2 θ)ω^2 =2mg×(l/2)sin θ (1+3 sin^2 θ)ω^2 l=3g sin θ ⇒ω=(√((3g sin θ)/((1+3 sin^2 θ)l))) ⇒v=BC×ω=2 sin θ (√((3gl sin θ)/(1+3 sin^2 θ))) =2 sin θ (√((6gl sin θ)/(5−3cos 2θ)))
$$\mathrm{A}{n}\:{other}\:{way}: \\ $$$$ \\ $$$${BC}=\mathrm{2}{l}\:\mathrm{sin}\:\theta \\ $$$${CM}^{\mathrm{2}} ={BC}^{\mathrm{2}} +{BM}^{\mathrm{2}} −\mathrm{2}\:{BC}×{BM}\:\mathrm{cos}\:\left(\mathrm{90}°−\theta\right) \\ $$$$=\mathrm{4}{l}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta+\frac{{l}^{\mathrm{2}} }{\mathrm{4}}−\mathrm{2}×\mathrm{2}{l}\:\mathrm{sin}\:\theta×\frac{{l}}{\mathrm{2}}×\mathrm{sin}\:\theta \\ $$$$=\frac{{l}^{\mathrm{2}} }{\mathrm{4}}\left(\mathrm{8}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right) \\ $$$${I}_{{c}} =\frac{{ml}^{\mathrm{2}} }{\mathrm{12}}+{m}×\frac{{l}^{\mathrm{2}} }{\mathrm{4}}\left(\mathrm{8}\:\mathrm{sin}^{\mathrm{2}} \:\theta+\mathrm{1}\right) \\ $$$$=\frac{{ml}^{\mathrm{2}} }{\mathrm{3}}\left(\mathrm{1}+\mathrm{6sin}^{\mathrm{2}} \:\theta\right) \\ $$$$ \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{ml}^{\mathrm{2}} }{\mathrm{3}}\right)\omega^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}×\frac{{ml}^{\mathrm{2}} }{\mathrm{3}}\left(\mathrm{1}+\mathrm{6}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right)\omega^{\mathrm{2}} =\mathrm{2}{mg}×\frac{{l}}{\mathrm{2}}\mathrm{sin}\:\theta \\ $$$$\left(\mathrm{1}+\mathrm{3}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right)\omega^{\mathrm{2}} {l}=\mathrm{3}{g}\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow\omega=\sqrt{\frac{\mathrm{3}{g}\:\mathrm{sin}\:\theta}{\left(\mathrm{1}+\mathrm{3}\:\mathrm{sin}^{\mathrm{2}} \:\theta\right){l}}} \\ $$$$ \\ $$$$\Rightarrow\boldsymbol{{v}}={BC}×\omega=\mathrm{2}\:\mathrm{sin}\:\theta\:\sqrt{\frac{\mathrm{3}{gl}\:\mathrm{sin}\:\theta}{\mathrm{1}+\mathrm{3}\:\mathrm{sin}^{\mathrm{2}} \:\theta}} \\ $$$$=\mathrm{2}\:\mathrm{sin}\:\theta\:\sqrt{\frac{\mathrm{6}{gl}\:\mathrm{sin}\:\theta}{\mathrm{5}−\mathrm{3cos}\:\mathrm{2}\theta}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *