Question Number 41519 by maxmathsup by imad last updated on 08/Aug/18
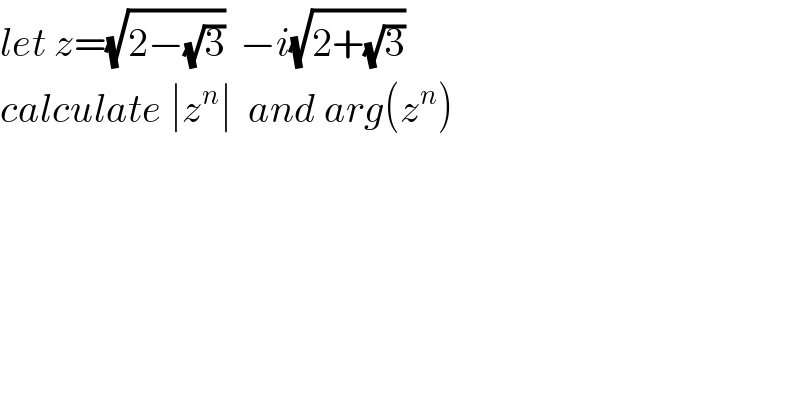
$${let}\:{z}=\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}\:\:−{i}\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}} \\ $$$${calculate}\:\mid{z}^{{n}} \mid\:\:{and}\:{arg}\left({z}^{{n}} \right) \\ $$
Answered by alex041103 last updated on 09/Aug/18
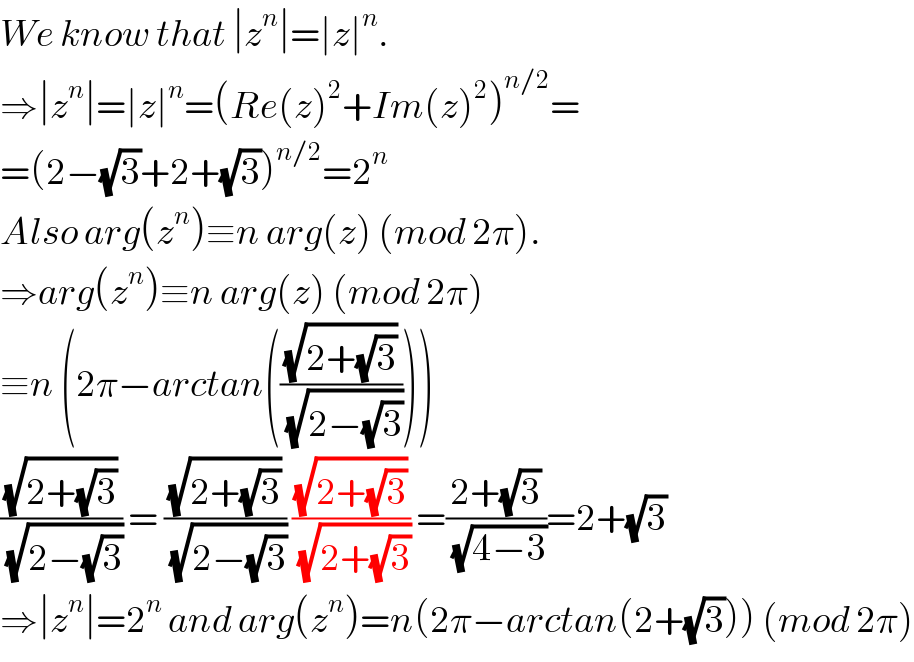
$${We}\:{know}\:{that}\:\mid{z}^{{n}} \mid=\mid{z}\mid^{{n}} . \\ $$$$\Rightarrow\mid{z}^{{n}} \mid=\mid{z}\mid^{{n}} =\left({Re}\left({z}\right)^{\mathrm{2}} +{Im}\left({z}\right)^{\mathrm{2}} \right)^{{n}/\mathrm{2}} = \\ $$$$=\left(\mathrm{2}−\sqrt{\mathrm{3}}+\mathrm{2}+\sqrt{\mathrm{3}}\right)^{{n}/\mathrm{2}} =\mathrm{2}^{{n}} \\ $$$${Also}\:{arg}\left({z}^{{n}} \right)\equiv{n}\:{arg}\left({z}\right)\:\left({mod}\:\mathrm{2}\pi\right). \\ $$$$\Rightarrow{arg}\left({z}^{{n}} \right)\equiv{n}\:{arg}\left({z}\right)\:\left({mod}\:\mathrm{2}\pi\right) \\ $$$$\equiv{n}\:\left(\mathrm{2}\pi−{arctan}\left(\frac{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}{\:\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}}\right)\right) \\ $$$$\frac{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}{\:\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}}\:=\:\frac{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}{\:\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}}\:\frac{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}{\:\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}\:=\frac{\mathrm{2}+\sqrt{\mathrm{3}}}{\:\sqrt{\mathrm{4}−\mathrm{3}}}=\mathrm{2}+\sqrt{\mathrm{3}} \\ $$$$\Rightarrow\mid{z}^{{n}} \mid=\mathrm{2}^{{n}} \:{and}\:{arg}\left({z}^{{n}} \right)={n}\left(\mathrm{2}\pi−{arctan}\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)\right)\:\left({mod}\:\mathrm{2}\pi\right) \\ $$
Answered by maxmathsup by imad last updated on 09/Aug/18
![we have cos^2 ((π/(12)))=((1+cos((π/6)))/2) =((1+((√3)/2))/2) =((2+(√3))/4) ⇒cos((π/(12)))=((√(2+(√3)))/2) also we have sin^2 ((π/(12)))=((1−cos((π/6)))/2) .((1−((√3)/2))/2) =((2−(√3))/4) ⇒sin((π/(12)))=((√(2−(√3)))/2) ⇒ z =2sin((π/(12)))−2i cos((π/(12))) =2 cos((π/2)−(π/(12)))−2i sin((π/2)−(π/(12))) =2 cos(((5π)/(12)))−2isin(((5π)/(12))) = 2 e^(−i((5π)/(12))) ⇒ z^n =2^n e^(−n((i5π)/(12))) ⇒ ∣z^n ∣ =2^n and arg(z^n )≡−((5nπ)/(12))[2π] .](https://www.tinkutara.com/question/Q41580.png)
$${we}\:{have}\:{cos}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{12}}\right)=\frac{\mathrm{1}+{cos}\left(\frac{\pi}{\mathrm{6}}\right)}{\mathrm{2}}\:=\frac{\mathrm{1}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}{\mathrm{2}}\:=\frac{\mathrm{2}+\sqrt{\mathrm{3}}}{\mathrm{4}}\:\Rightarrow{cos}\left(\frac{\pi}{\mathrm{12}}\right)=\frac{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}{\mathrm{2}}\:{also} \\ $$$${we}\:{have}\:{sin}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{12}}\right)=\frac{\mathrm{1}−{cos}\left(\frac{\pi}{\mathrm{6}}\right)}{\mathrm{2}}\:.\frac{\mathrm{1}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}{\mathrm{2}}\:=\frac{\mathrm{2}−\sqrt{\mathrm{3}}}{\mathrm{4}}\:\Rightarrow{sin}\left(\frac{\pi}{\mathrm{12}}\right)=\frac{\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}}{\mathrm{2}}\:\Rightarrow \\ $$$${z}\:=\mathrm{2}{sin}\left(\frac{\pi}{\mathrm{12}}\right)−\mathrm{2}{i}\:{cos}\left(\frac{\pi}{\mathrm{12}}\right)\:=\mathrm{2}\:{cos}\left(\frac{\pi}{\mathrm{2}}−\frac{\pi}{\mathrm{12}}\right)−\mathrm{2}{i}\:{sin}\left(\frac{\pi}{\mathrm{2}}−\frac{\pi}{\mathrm{12}}\right) \\ $$$$=\mathrm{2}\:{cos}\left(\frac{\mathrm{5}\pi}{\mathrm{12}}\right)−\mathrm{2}{isin}\left(\frac{\mathrm{5}\pi}{\mathrm{12}}\right)\:=\:\mathrm{2}\:{e}^{−{i}\frac{\mathrm{5}\pi}{\mathrm{12}}} \:\:\Rightarrow\:{z}^{{n}} \:=\mathrm{2}^{{n}} \:{e}^{−{n}\frac{{i}\mathrm{5}\pi}{\mathrm{12}}} \:\Rightarrow \\ $$$$\mid{z}^{{n}} \mid\:=\mathrm{2}^{{n}} \:\:{and}\:{arg}\left({z}^{{n}} \right)\equiv−\frac{\mathrm{5}{n}\pi}{\mathrm{12}}\left[\mathrm{2}\pi\right]\:. \\ $$
