Question Number 172784 by dragan91 last updated on 01/Jul/22

Answered by Eulerian last updated on 01/Jul/22
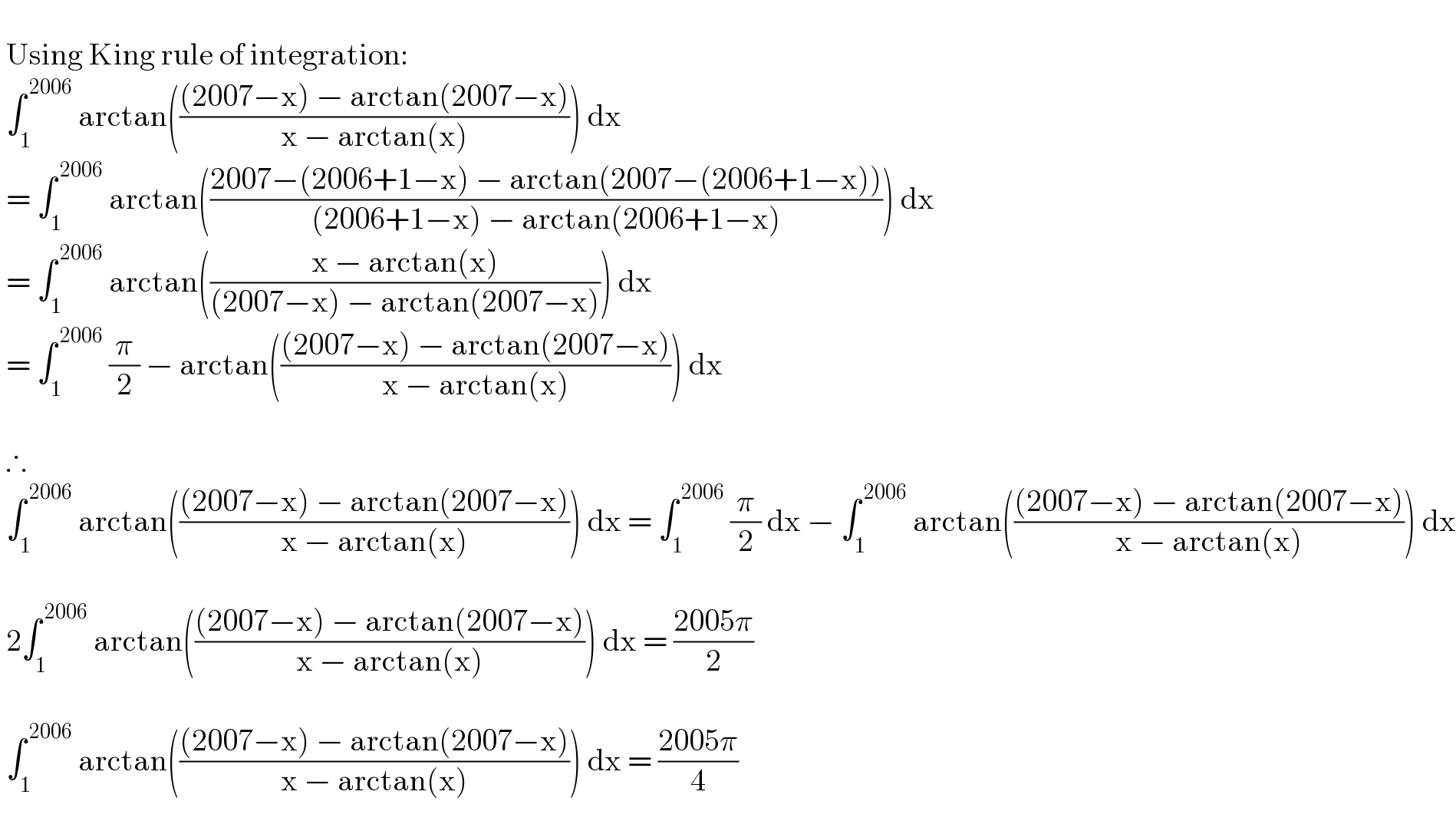
$$\: \\ $$$$\:\mathrm{Using}\:\mathrm{King}\:\mathrm{rule}\:\mathrm{of}\:\mathrm{integration}: \\ $$$$\:\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\mathrm{arctan}\left(\frac{\left(\mathrm{2007}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2007}−\mathrm{x}\right)}{\mathrm{x}\:−\:\mathrm{arctan}\left(\mathrm{x}\right)}\right)\:\mathrm{dx} \\ $$$$\:=\:\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\mathrm{arctan}\left(\frac{\mathrm{2007}−\left(\mathrm{2006}+\mathrm{1}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2007}−\left(\mathrm{2006}+\mathrm{1}−\mathrm{x}\right)\right)}{\left(\mathrm{2006}+\mathrm{1}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2006}+\mathrm{1}−\mathrm{x}\right)}\right)\:\mathrm{dx} \\ $$$$\:=\:\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\mathrm{arctan}\left(\frac{\mathrm{x}\:−\:\mathrm{arctan}\left(\mathrm{x}\right)}{\left(\mathrm{2007}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2007}−\mathrm{x}\right)}\right)\:\mathrm{dx} \\ $$$$\:=\:\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\frac{\pi}{\mathrm{2}}\:−\:\mathrm{arctan}\left(\frac{\left(\mathrm{2007}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2007}−\mathrm{x}\right)}{\mathrm{x}\:−\:\mathrm{arctan}\left(\mathrm{x}\right)}\right)\:\mathrm{dx} \\ $$$$\: \\ $$$$\:\therefore \\ $$$$\:\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\mathrm{arctan}\left(\frac{\left(\mathrm{2007}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2007}−\mathrm{x}\right)}{\mathrm{x}\:−\:\mathrm{arctan}\left(\mathrm{x}\right)}\right)\:\mathrm{dx}\:=\:\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\frac{\pi}{\mathrm{2}}\:\mathrm{dx}\:−\:\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\mathrm{arctan}\left(\frac{\left(\mathrm{2007}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2007}−\mathrm{x}\right)}{\mathrm{x}\:−\:\mathrm{arctan}\left(\mathrm{x}\right)}\right)\:\mathrm{dx} \\ $$$$\: \\ $$$$\:\mathrm{2}\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\mathrm{arctan}\left(\frac{\left(\mathrm{2007}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2007}−\mathrm{x}\right)}{\mathrm{x}\:−\:\mathrm{arctan}\left(\mathrm{x}\right)}\right)\:\mathrm{dx}\:=\:\frac{\mathrm{2005}\pi}{\mathrm{2}} \\ $$$$\: \\ $$$$\:\int_{\mathrm{1}} ^{\:\mathrm{2006}} \:\mathrm{arctan}\left(\frac{\left(\mathrm{2007}−\mathrm{x}\right)\:−\:\mathrm{arctan}\left(\mathrm{2007}−\mathrm{x}\right)}{\mathrm{x}\:−\:\mathrm{arctan}\left(\mathrm{x}\right)}\right)\:\mathrm{dx}\:=\:\frac{\mathrm{2005}\pi}{\mathrm{4}} \\ $$
