Question-109508

Question Number 109508 by Study last updated on 24/Aug/20

Commented by som(math1967) last updated on 24/Aug/20

(1/4)π×2^2 −{(1/2)π×(((√2)/2))^2 +(1/2)×1×1} (π−(π/4)−(1/2))cm^2

$$\:\frac{\mathrm{1}}{\mathrm{4}}\pi×\mathrm{2}^{\mathrm{2}} −\left\{\frac{\mathrm{1}}{\mathrm{2}}\pi×\left(\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}×\mathrm{1}×\mathrm{1}\right\} \\ $$$$\left(\pi−\frac{\pi}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}}\right)\mathrm{cm}^{\mathrm{2}} \\ $$

Answered by Her_Majesty last updated on 24/Aug/20

$$\boldsymbol{{yes}}\:\boldsymbol{{I}}\:\boldsymbol{{can}} \\ $$

Answered by Rasheed.Sindhi last updated on 24/Aug/20

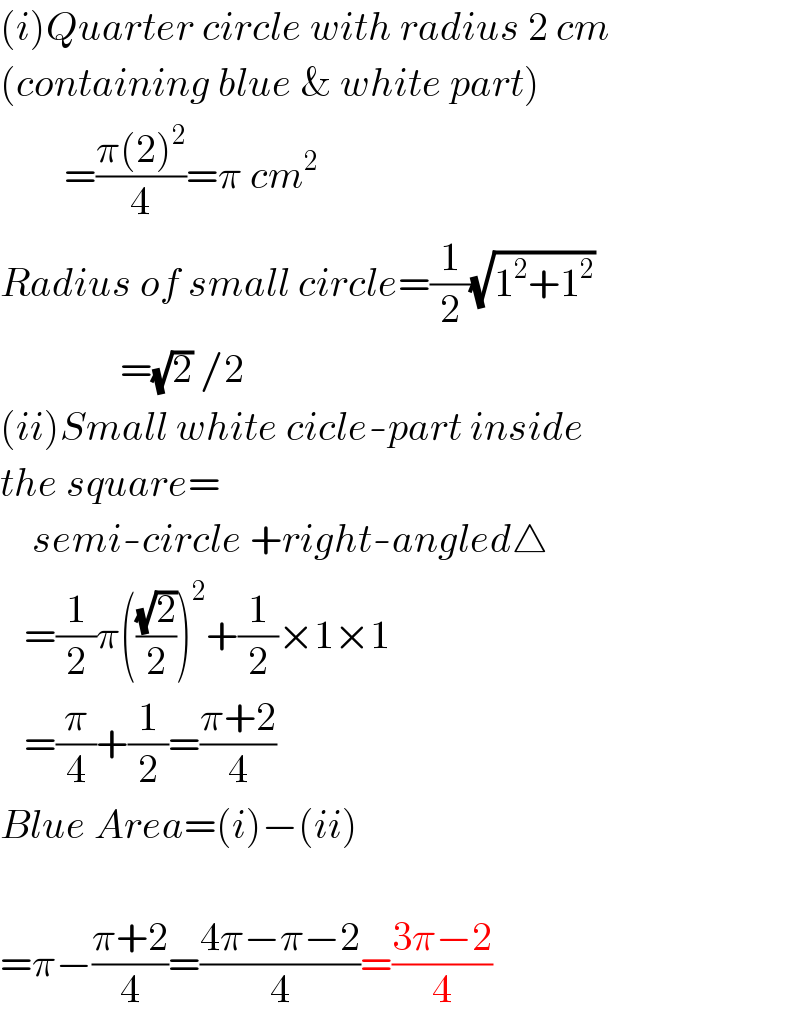
(i)Quarter circle with radius 2 cm (containing blue & white part) =((π(2)^2 )/4)=π cm^2 Radius of small circle=(1/2)(√(1^2 +1^2 )) =(√2) /2 (ii)Small white cicle-part inside the square= semi-circle +right-angled△ =(1/2)π(((√2)/2))^2 +(1/2)×1×1 =(π/4)+(1/2)=((π+2)/4) Blue Area=(i)−(ii) =π−((π+2)/4)=((4π−π−2)/4)=((3π−2)/4)

$$\left({i}\right){Quarter}\:{circle}\:{with}\:{radius}\:\mathrm{2}\:{cm} \\ $$$$\left({containing}\:{blue}\:\&\:{white}\:{part}\right) \\ $$$$\:\:\:\:\:\:\:\:=\frac{\pi\left(\mathrm{2}\right)^{\mathrm{2}} }{\mathrm{4}}=\pi\:{cm}^{\mathrm{2}} \\ $$$${Radius}\:{of}\:{small}\:{circle}=\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\mathrm{1}^{\mathrm{2}} +\mathrm{1}^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\sqrt{\mathrm{2}}\:/\mathrm{2} \\ $$$$\left({ii}\right){Small}\:{white}\:{cicle}-{part}\:{inside} \\ $$$${the}\:{square}= \\ $$$$\:\:\:\:{semi}-{circle}\:+{right}-{angled}\bigtriangleup \\ $$$$\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\pi\left(\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}×\mathrm{1}×\mathrm{1} \\ $$$$\:\:\:=\frac{\pi}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}=\frac{\pi+\mathrm{2}}{\mathrm{4}} \\ $$$${Blue}\:{Area}=\left({i}\right)−\left({ii}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\: \\ $$$$=\pi−\frac{\pi+\mathrm{2}}{\mathrm{4}}=\frac{\mathrm{4}\pi−\pi−\mathrm{2}}{\mathrm{4}}=\frac{\mathrm{3}\pi−\mathrm{2}}{\mathrm{4}} \\ $$

Commented by Rasheed.Sindhi last updated on 24/Aug/20