Question Number 175333 by mnjuly1970 last updated on 27/Aug/22
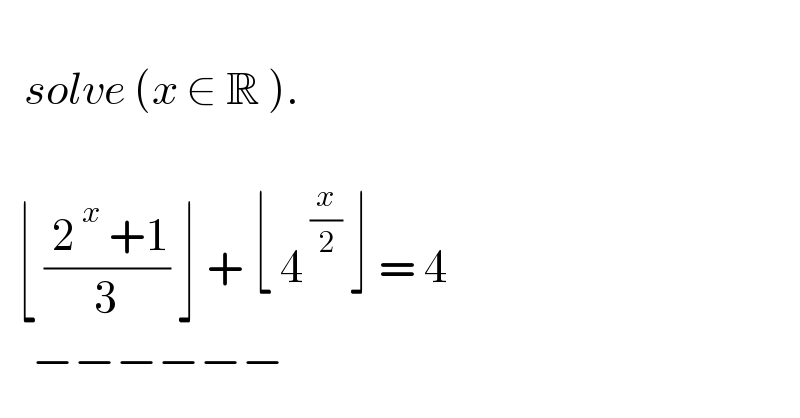
$$ \\ $$$$\:\:\:{solve}\:\left({x}\:\in\:\mathbb{R}\:\right). \\ $$$$ \\ $$$$\:\:\lfloor\:\frac{\:\mathrm{2}^{\:{x}} \:+\mathrm{1}}{\mathrm{3}}\:\rfloor\:+\:\lfloor\:\mathrm{4}^{\:\frac{{x}}{\mathrm{2}}} \:\rfloor\:=\:\mathrm{4} \\ $$$$\:\:\:\:−−−−−−\: \\ $$
Answered by mr W last updated on 27/Aug/22
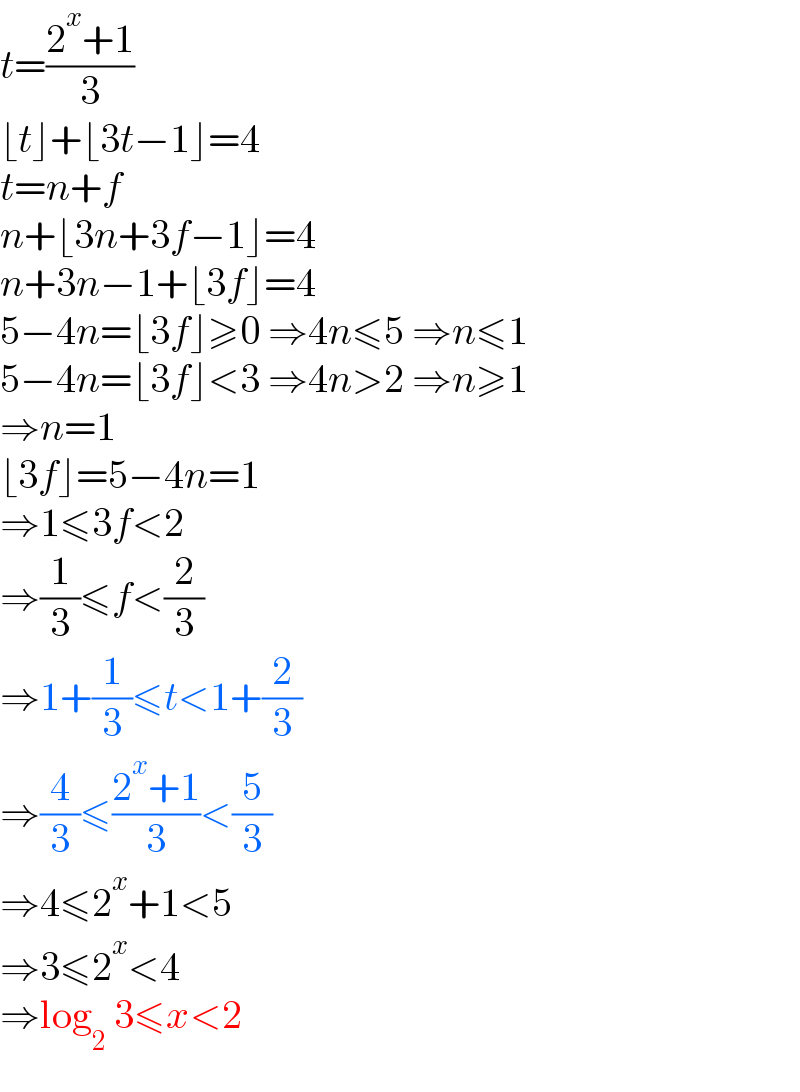
$${t}=\frac{\mathrm{2}^{{x}} +\mathrm{1}}{\mathrm{3}} \\ $$$$\lfloor{t}\rfloor+\lfloor\mathrm{3}{t}−\mathrm{1}\rfloor=\mathrm{4} \\ $$$${t}={n}+{f} \\ $$$${n}+\lfloor\mathrm{3}{n}+\mathrm{3}{f}−\mathrm{1}\rfloor=\mathrm{4} \\ $$$${n}+\mathrm{3}{n}−\mathrm{1}+\lfloor\mathrm{3}{f}\rfloor=\mathrm{4} \\ $$$$\mathrm{5}−\mathrm{4}{n}=\lfloor\mathrm{3}{f}\rfloor\geqslant\mathrm{0}\:\Rightarrow\mathrm{4}{n}\leqslant\mathrm{5}\:\Rightarrow{n}\leqslant\mathrm{1} \\ $$$$\mathrm{5}−\mathrm{4}{n}=\lfloor\mathrm{3}{f}\rfloor<\mathrm{3}\:\Rightarrow\mathrm{4}{n}>\mathrm{2}\:\Rightarrow{n}\geqslant\mathrm{1} \\ $$$$\Rightarrow{n}=\mathrm{1} \\ $$$$\lfloor\mathrm{3}{f}\rfloor=\mathrm{5}−\mathrm{4}{n}=\mathrm{1} \\ $$$$\Rightarrow\mathrm{1}\leqslant\mathrm{3}{f}<\mathrm{2} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{3}}\leqslant{f}<\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\Rightarrow\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}\leqslant{t}<\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\Rightarrow\frac{\mathrm{4}}{\mathrm{3}}\leqslant\frac{\mathrm{2}^{{x}} +\mathrm{1}}{\mathrm{3}}<\frac{\mathrm{5}}{\mathrm{3}} \\ $$$$\Rightarrow\mathrm{4}\leqslant\mathrm{2}^{{x}} +\mathrm{1}<\mathrm{5} \\ $$$$\Rightarrow\mathrm{3}\leqslant\mathrm{2}^{{x}} <\mathrm{4} \\ $$$$\Rightarrow\mathrm{log}_{\mathrm{2}} \:\mathrm{3}\leqslant{x}<\mathrm{2} \\ $$
Commented by mnjuly1970 last updated on 27/Aug/22

$${thankz}\:{alot}\:{sir}\:{W} \\ $$
Commented by Tawa11 last updated on 28/Aug/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
