Question Number 109839 by john santu last updated on 25/Aug/20
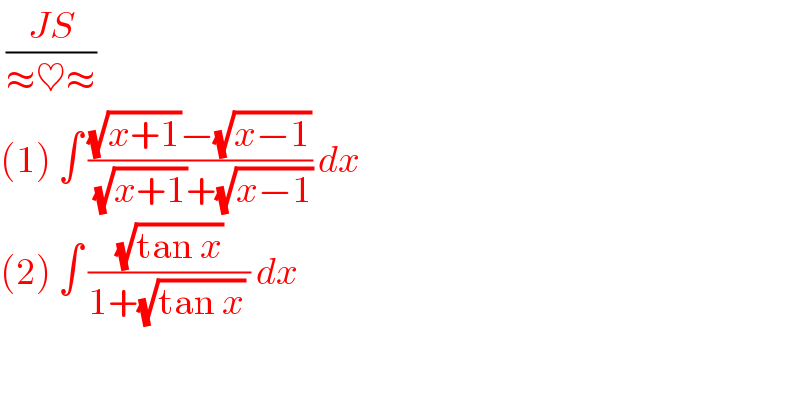
$$\:\frac{{JS}}{\approx\heartsuit\approx} \\ $$$$\left(\mathrm{1}\right)\:\int\:\frac{\sqrt{{x}+\mathrm{1}}−\sqrt{{x}−\mathrm{1}}}{\:\sqrt{{x}+\mathrm{1}}+\sqrt{{x}−\mathrm{1}}}\:{dx} \\ $$$$\left(\mathrm{2}\right)\:\int\:\frac{\sqrt{\mathrm{tan}\:{x}}}{\mathrm{1}+\sqrt{\mathrm{tan}\:{x}}\:}\:{dx}\: \\ $$
Commented by Her_Majesty last updated on 25/Aug/20
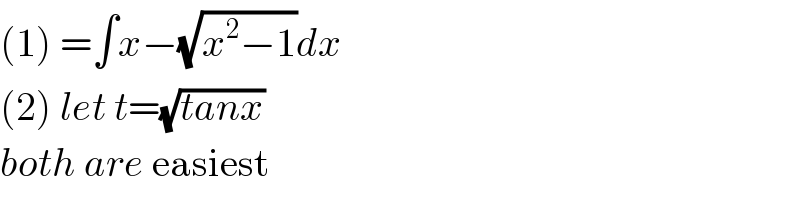
$$\left(\mathrm{1}\right)\:=\int{x}−\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}{dx} \\ $$$$\left(\mathrm{2}\right)\:{let}\:{t}=\sqrt{{tanx}} \\ $$$${both}\:{are}\:\mathrm{easiest} \\ $$
Answered by Dwaipayan Shikari last updated on 25/Aug/20
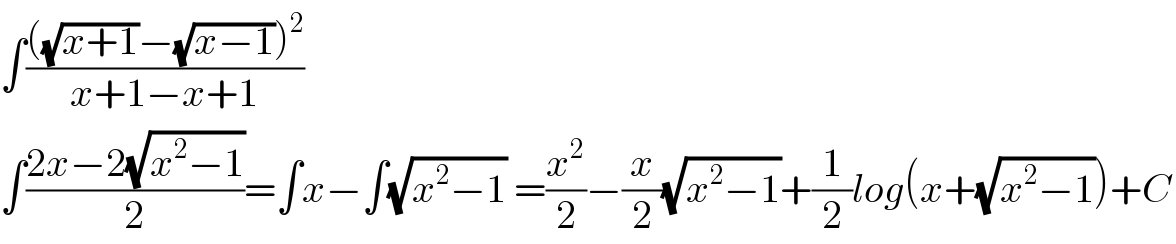
$$\int\frac{\left(\sqrt{{x}+\mathrm{1}}−\sqrt{{x}−\mathrm{1}}\right)^{\mathrm{2}} }{{x}+\mathrm{1}−{x}+\mathrm{1}} \\ $$$$\int\frac{\mathrm{2}{x}−\mathrm{2}\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}}{\mathrm{2}}=\int{x}−\int\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}\:=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}−\frac{{x}}{\mathrm{2}}\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{2}}{log}\left({x}+\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}\right)+{C} \\ $$
Answered by bobhans last updated on 26/Aug/20
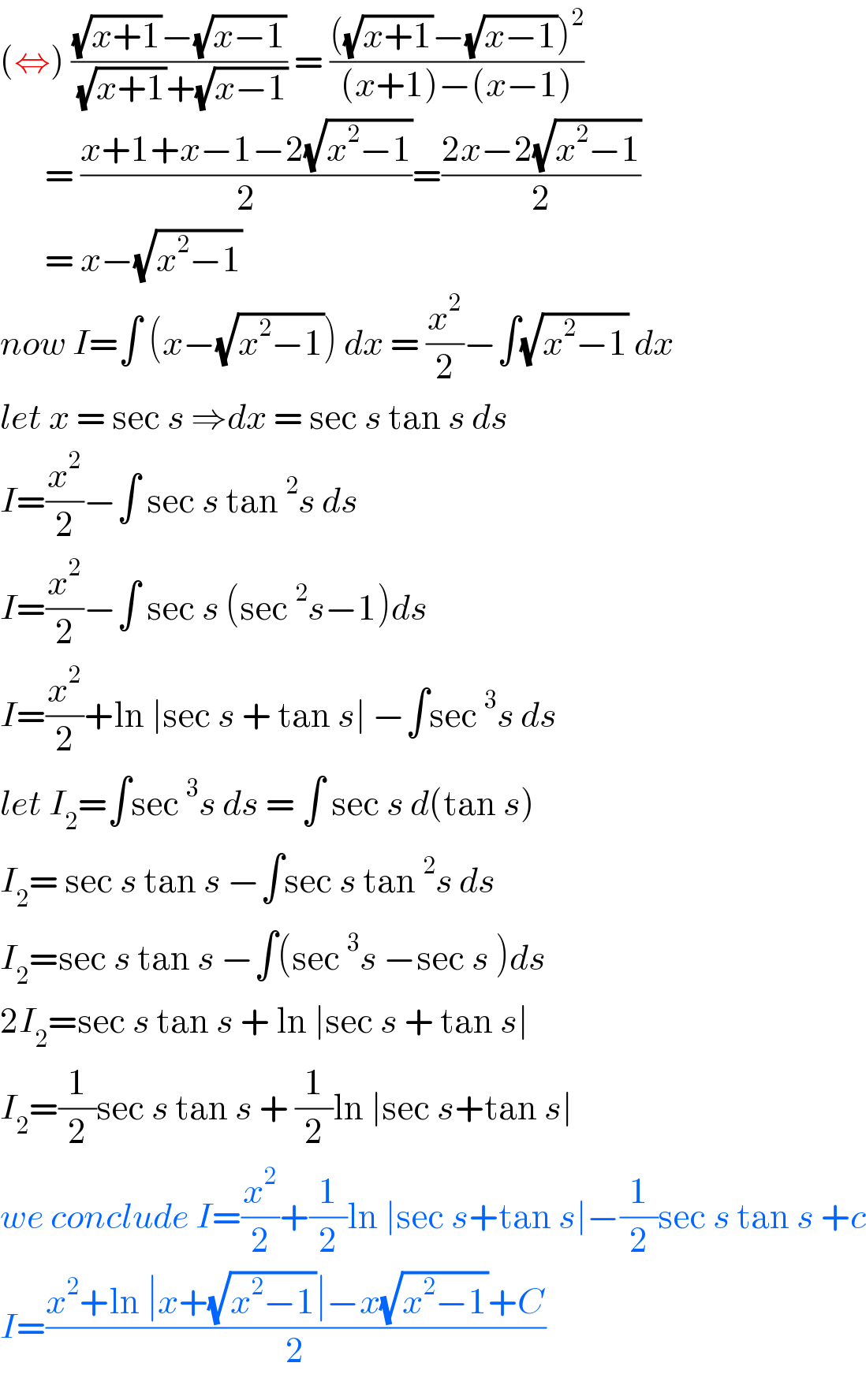
$$\left(\Leftrightarrow\right)\:\frac{\sqrt{{x}+\mathrm{1}}−\sqrt{{x}−\mathrm{1}}}{\:\sqrt{{x}+\mathrm{1}}+\sqrt{{x}−\mathrm{1}}}\:=\:\frac{\left(\sqrt{{x}+\mathrm{1}}−\sqrt{{x}−\mathrm{1}}\right)^{\mathrm{2}} }{\left({x}+\mathrm{1}\right)−\left({x}−\mathrm{1}\right)} \\ $$$$\:\:\:\:\:\:\:=\:\frac{{x}+\mathrm{1}+{x}−\mathrm{1}−\mathrm{2}\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}}{\mathrm{2}}=\frac{\mathrm{2}{x}−\mathrm{2}\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}}{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:=\:{x}−\sqrt{{x}^{\mathrm{2}} −\mathrm{1}} \\ $$$${now}\:{I}=\int\:\left({x}−\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}\right)\:{dx}\:=\:\frac{{x}^{\mathrm{2}} }{\mathrm{2}}−\int\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}\:{dx} \\ $$$${let}\:{x}\:=\:\mathrm{sec}\:{s}\:\Rightarrow{dx}\:=\:\mathrm{sec}\:{s}\:\mathrm{tan}\:{s}\:{ds} \\ $$$${I}=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}−\int\:\mathrm{sec}\:{s}\:\mathrm{tan}\:^{\mathrm{2}} {s}\:{ds}\: \\ $$$${I}=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}−\int\:\mathrm{sec}\:{s}\:\left(\mathrm{sec}\:^{\mathrm{2}} {s}−\mathrm{1}\right){ds} \\ $$$${I}=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}+\mathrm{ln}\:\mid\mathrm{sec}\:{s}\:+\:\mathrm{tan}\:{s}\mid\:−\int\mathrm{sec}\:^{\mathrm{3}} {s}\:{ds} \\ $$$${let}\:{I}_{\mathrm{2}} =\int\mathrm{sec}\:^{\mathrm{3}} {s}\:{ds}\:=\:\int\:\mathrm{sec}\:{s}\:{d}\left(\mathrm{tan}\:{s}\right) \\ $$$${I}_{\mathrm{2}} =\:\mathrm{sec}\:{s}\:\mathrm{tan}\:{s}\:−\int\mathrm{sec}\:{s}\:\mathrm{tan}\:^{\mathrm{2}} {s}\:{ds} \\ $$$${I}_{\mathrm{2}} =\mathrm{sec}\:{s}\:\mathrm{tan}\:{s}\:−\int\left(\mathrm{sec}\:^{\mathrm{3}} {s}\:−\mathrm{sec}\:{s}\:\right){ds} \\ $$$$\mathrm{2}{I}_{\mathrm{2}} =\mathrm{sec}\:{s}\:\mathrm{tan}\:{s}\:+\:\mathrm{ln}\:\mid\mathrm{sec}\:{s}\:+\:\mathrm{tan}\:{s}\mid\: \\ $$$${I}_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sec}\:{s}\:\mathrm{tan}\:{s}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\mid\mathrm{sec}\:{s}+\mathrm{tan}\:{s}\mid \\ $$$${we}\:{conclude}\:{I}=\frac{{x}^{\mathrm{2}} }{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\mid\mathrm{sec}\:{s}+\mathrm{tan}\:{s}\mid−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sec}\:{s}\:\mathrm{tan}\:{s}\:+{c} \\ $$$${I}=\frac{{x}^{\mathrm{2}} +\mathrm{ln}\:\mid{x}+\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}\mid−{x}\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}+{C}}{\mathrm{2}} \\ $$
Answered by bobhans last updated on 26/Aug/20
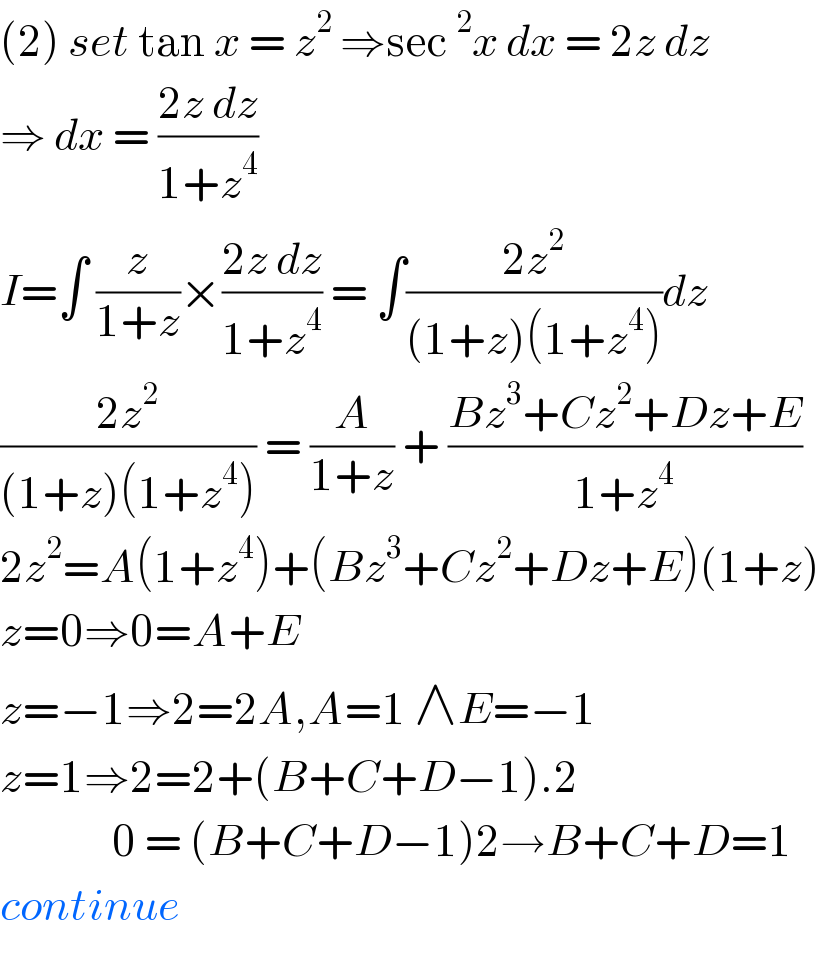
$$\left(\mathrm{2}\right)\:{set}\:\mathrm{tan}\:{x}\:=\:{z}^{\mathrm{2}} \:\Rightarrow\mathrm{sec}\:^{\mathrm{2}} {x}\:{dx}\:=\:\mathrm{2}{z}\:{dz} \\ $$$$\Rightarrow\:{dx}\:=\:\frac{\mathrm{2}{z}\:{dz}}{\mathrm{1}+{z}^{\mathrm{4}} }\: \\ $$$${I}=\int\:\frac{{z}}{\mathrm{1}+{z}}×\frac{\mathrm{2}{z}\:{dz}}{\mathrm{1}+{z}^{\mathrm{4}} }\:=\:\int\frac{\mathrm{2}{z}^{\mathrm{2}} }{\left(\mathrm{1}+{z}\right)\left(\mathrm{1}+{z}^{\mathrm{4}} \right)}{dz} \\ $$$$\frac{\mathrm{2}{z}^{\mathrm{2}} }{\left(\mathrm{1}+{z}\right)\left(\mathrm{1}+{z}^{\mathrm{4}} \right)}\:=\:\frac{{A}}{\mathrm{1}+{z}}\:+\:\frac{{Bz}^{\mathrm{3}} +{Cz}^{\mathrm{2}} +{Dz}+{E}}{\mathrm{1}+{z}^{\mathrm{4}} } \\ $$$$\mathrm{2}{z}^{\mathrm{2}} ={A}\left(\mathrm{1}+{z}^{\mathrm{4}} \right)+\left({Bz}^{\mathrm{3}} +{Cz}^{\mathrm{2}} +{Dz}+{E}\right)\left(\mathrm{1}+{z}\right) \\ $$$${z}=\mathrm{0}\Rightarrow\mathrm{0}={A}+{E} \\ $$$${z}=−\mathrm{1}\Rightarrow\mathrm{2}=\mathrm{2}{A},{A}=\mathrm{1}\:\wedge{E}=−\mathrm{1} \\ $$$${z}=\mathrm{1}\Rightarrow\mathrm{2}=\mathrm{2}+\left({B}+{C}+{D}−\mathrm{1}\right).\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{0}\:=\:\left({B}+{C}+{D}−\mathrm{1}\right)\mathrm{2}\rightarrow{B}+{C}+{D}=\mathrm{1} \\ $$$${continue} \\ $$
Commented by Sarah85 last updated on 26/Aug/20
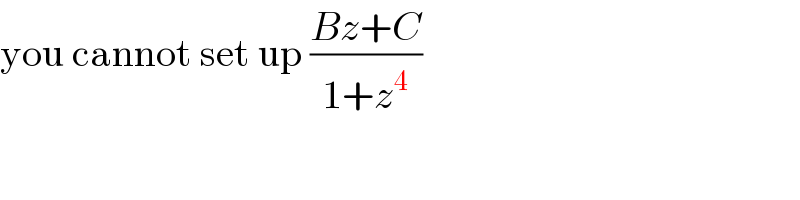
$$\mathrm{you}\:\mathrm{cannot}\:\mathrm{set}\:\mathrm{up}\:\frac{{Bz}+{C}}{\mathrm{1}+{z}^{\mathrm{4}} }\: \\ $$
Commented by bobhans last updated on 26/Aug/20
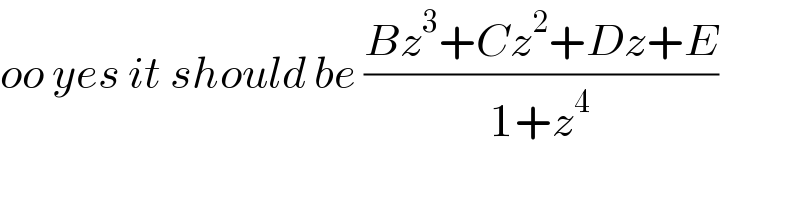
$${oo}\:{yes}\:{it}\:{should}\:{be}\:\frac{{Bz}^{\mathrm{3}} +{Cz}^{\mathrm{2}} +{Dz}+{E}}{\mathrm{1}+{z}^{\mathrm{4}} } \\ $$
Answered by Sarah85 last updated on 26/Aug/20
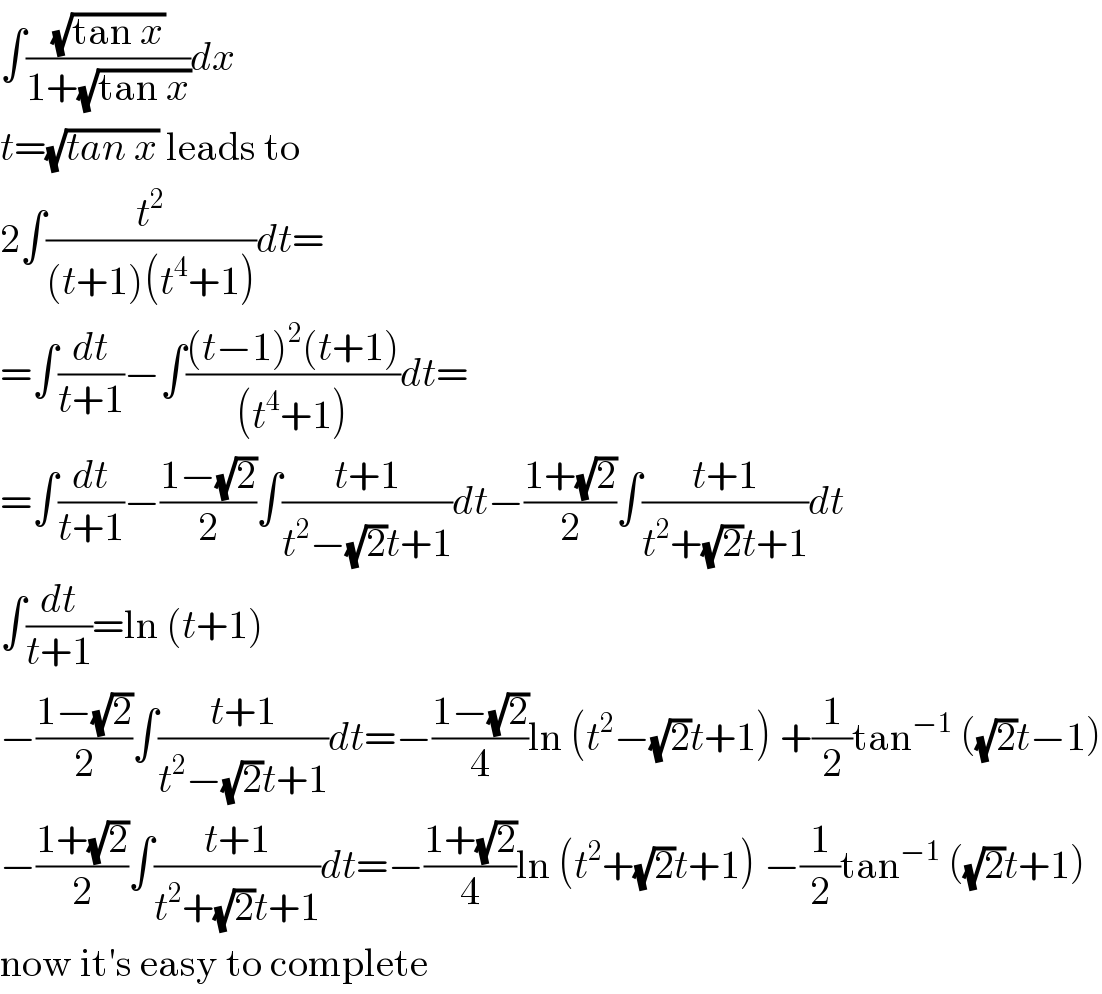
$$\int\frac{\sqrt{\mathrm{tan}\:{x}}}{\mathrm{1}+\sqrt{\mathrm{tan}\:{x}}}{dx} \\ $$$${t}=\sqrt{{tan}\:{x}}\:\mathrm{leads}\:\mathrm{to} \\ $$$$\mathrm{2}\int\frac{{t}^{\mathrm{2}} }{\left({t}+\mathrm{1}\right)\left({t}^{\mathrm{4}} +\mathrm{1}\right)}{dt}= \\ $$$$=\int\frac{{dt}}{{t}+\mathrm{1}}−\int\frac{\left({t}−\mathrm{1}\right)^{\mathrm{2}} \left({t}+\mathrm{1}\right)}{\left({t}^{\mathrm{4}} +\mathrm{1}\right)}{dt}= \\ $$$$=\int\frac{{dt}}{{t}+\mathrm{1}}−\frac{\mathrm{1}−\sqrt{\mathrm{2}}}{\mathrm{2}}\int\frac{{t}+\mathrm{1}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}{dt}−\frac{\mathrm{1}+\sqrt{\mathrm{2}}}{\mathrm{2}}\int\frac{{t}+\mathrm{1}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}{dt} \\ $$$$\int\frac{{dt}}{{t}+\mathrm{1}}=\mathrm{ln}\:\left({t}+\mathrm{1}\right) \\ $$$$−\frac{\mathrm{1}−\sqrt{\mathrm{2}}}{\mathrm{2}}\int\frac{{t}+\mathrm{1}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}{dt}=−\frac{\mathrm{1}−\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{ln}\:\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)\:+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} \:\left(\sqrt{\mathrm{2}}{t}−\mathrm{1}\right) \\ $$$$−\frac{\mathrm{1}+\sqrt{\mathrm{2}}}{\mathrm{2}}\int\frac{{t}+\mathrm{1}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}{dt}=−\frac{\mathrm{1}+\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{ln}\:\left({t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)\:−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} \:\left(\sqrt{\mathrm{2}}{t}+\mathrm{1}\right) \\ $$$$\mathrm{now}\:\mathrm{it}'\mathrm{s}\:\mathrm{easy}\:\mathrm{to}\:\mathrm{complete} \\ $$
