Menu Close

Define-the-laplace-transformation-equation-and-use-the-transformation-equation-transform-dy-dx-and-d-2-y-dx-2-hence-solve-the-equation-d-2-y-dx-2-5-dy-dx-4-e-x-sin-2x-Using-the-l

Tinku Tara 0 Comments
Pin




Question Number 110178 by Rio Michael last updated on 27/Aug/20
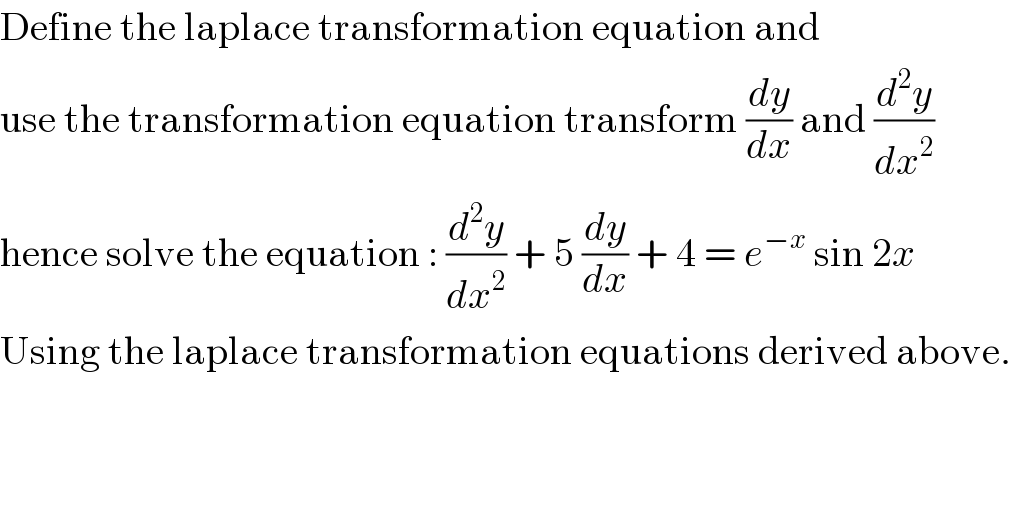
Define the laplace transformation equation and use the transformation equation transform (dy/dx) and (d^2 y/dx^2 ) hence solve the equation : (d^2 y/dx^2 ) + 5 (dy/dx) + 4 = e^(−x) sin 2x Using the laplace transformation equations derived above.
$$\mathrm{Define}\:\mathrm{the}\:\mathrm{laplace}\:\mathrm{transformation}\:\mathrm{equation}\:\mathrm{and} \\ $$$$\mathrm{use}\:\mathrm{the}\:\mathrm{transformation}\:\mathrm{equation}\:\mathrm{transform}\:\frac{{dy}}{{dx}}\:\mathrm{and}\:\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} } \\ $$$$\mathrm{hence}\:\mathrm{solve}\:\mathrm{the}\:\mathrm{equation}\::\:\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }\:+\:\mathrm{5}\:\frac{{dy}}{{dx}}\:+\:\mathrm{4}\:=\:{e}^{−{x}} \:\mathrm{sin}\:\mathrm{2}{x} \\ $$$$\mathrm{Using}\:\mathrm{the}\:\mathrm{laplace}\:\mathrm{transformation}\:\mathrm{equations}\:\mathrm{derived}\:\mathrm{above}. \\ $$
Answered by Aziztisffola last updated on 27/Aug/20
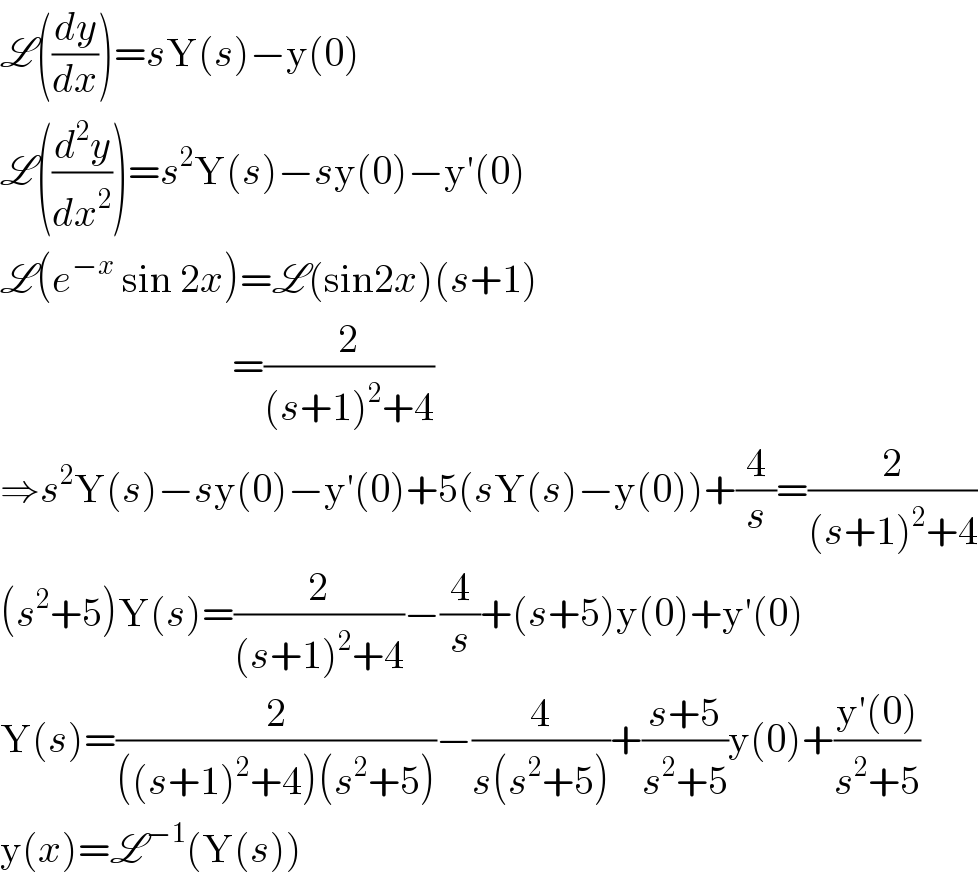
L((dy/dx))=sY(s)−y(0) L((d^2 y/dx^2 ))=s^2 Y(s)−sy(0)−y′(0) L(e^(−x) sin 2x)=L(sin2x)(s+1) =(2/((s+1)^2 +4)) ⇒s^2 Y(s)−sy(0)−y′(0)+5(sY(s)−y(0))+(4/s)=(2/((s+1)^2 +4)) (s^2 +5)Y(s)=(2/((s+1)^2 +4))−(4/s)+(s+5)y(0)+y′(0) Y(s)=(2/(((s+1)^2 +4)(s^2 +5)))−(4/(s(s^2 +5)))+((s+5)/(s^2 +5))y(0)+((y′(0))/(s^2 +5)) y(x)=L^(−1) (Y(s))
$$\mathscr{L}\left(\frac{{dy}}{{dx}}\right)={s}\mathrm{Y}\left({s}\right)−\mathrm{y}\left(\mathrm{0}\right) \\ $$$$\mathscr{L}\left(\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }\right)={s}^{\mathrm{2}} \mathrm{Y}\left({s}\right)−{s}\mathrm{y}\left(\mathrm{0}\right)−\mathrm{y}'\left(\mathrm{0}\right) \\ $$$$\mathscr{L}\left({e}^{−{x}} \:\mathrm{sin}\:\mathrm{2}{x}\right)=\mathscr{L}\left(\mathrm{sin2}{x}\right)\left({s}+\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{2}}{\left({s}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}} \\ $$$$\Rightarrow{s}^{\mathrm{2}} \mathrm{Y}\left({s}\right)−{s}\mathrm{y}\left(\mathrm{0}\right)−\mathrm{y}'\left(\mathrm{0}\right)+\mathrm{5}\left({s}\mathrm{Y}\left({s}\right)−\mathrm{y}\left(\mathrm{0}\right)\right)+\frac{\mathrm{4}}{{s}}=\frac{\mathrm{2}}{\left({s}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}} \\ $$$$\left({s}^{\mathrm{2}} +\mathrm{5}\right)\mathrm{Y}\left({s}\right)=\frac{\mathrm{2}}{\left({s}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}}−\frac{\mathrm{4}}{{s}}+\left({s}+\mathrm{5}\right)\mathrm{y}\left(\mathrm{0}\right)+\mathrm{y}'\left(\mathrm{0}\right) \\ $$$$\mathrm{Y}\left({s}\right)=\frac{\mathrm{2}}{\left(\left({s}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}\right)\left({s}^{\mathrm{2}} +\mathrm{5}\right)}−\frac{\mathrm{4}}{{s}\left({s}^{\mathrm{2}} +\mathrm{5}\right)}+\frac{{s}+\mathrm{5}}{{s}^{\mathrm{2}} +\mathrm{5}}\mathrm{y}\left(\mathrm{0}\right)+\frac{\mathrm{y}'\left(\mathrm{0}\right)}{{s}^{\mathrm{2}} +\mathrm{5}} \\ $$$$\mathrm{y}\left({x}\right)=\mathscr{L}^{−\mathrm{1}} \left(\mathrm{Y}\left({s}\right)\right) \\ $$
Commented by Rio Michael last updated on 27/Aug/20
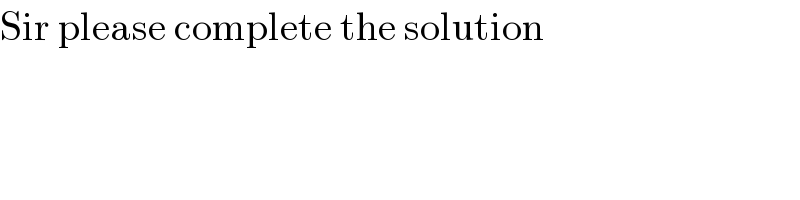
Sir please complete the solution
$$\mathrm{Sir}\:\mathrm{please}\:\mathrm{complete}\:\mathrm{the}\:\mathrm{solution} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *