Question Number 70025 by Tony Lin last updated on 30/Sep/19
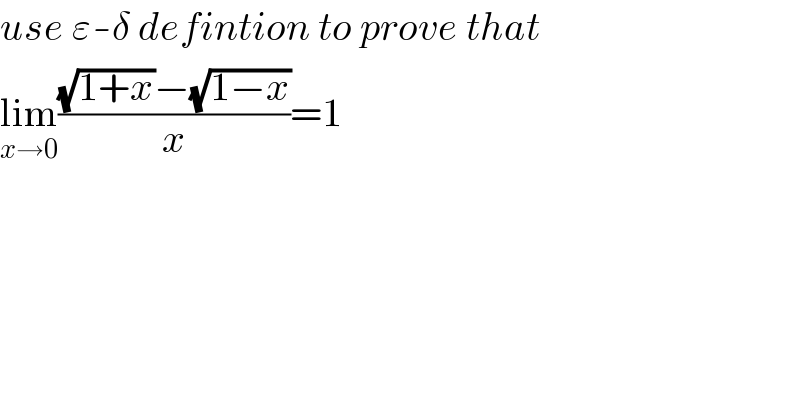
$${use}\:\varepsilon-\delta\:{defintion}\:{to}\:{prove}\:{that} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{\mathrm{1}+{x}}−\sqrt{\mathrm{1}−{x}}}{{x}}=\mathrm{1} \\ $$
Commented by mind is power last updated on 01/Oct/19

$$={li}\underset{{t}\rightarrow\mathrm{0}} {{m}}\frac{\sqrt{\mathrm{1}+{sin}\left({t}\right)}−\sqrt{\mathrm{1}−{sin}\left({t}\right)}}{{sin}\left({t}\right)} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}_{} }\frac{\mathrm{1}}{{cos}\left(\frac{{t}}{\mathrm{2}}\right)} \\ $$$$\forall\varepsilon>\mathrm{0}\:\:\exists\eta>\mathrm{0} \\ $$$$\mid{x}\mid\leqslant\eta\Rightarrow\mid{f}\left({x}\right)−\mathrm{1}\mid\leqslant\varepsilon \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{1}−\varepsilon\leqslant\frac{\mathrm{1}}{{cos}\left(\frac{{t}}{\mathrm{2}}\right)}\leqslant\mathrm{1}+\varepsilon \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{1}−\varepsilon}\geqslant{cos}\left(\frac{{t}}{\mathrm{2}}\right)\geqslant\frac{\mathrm{1}}{\mathrm{1}+\varepsilon} \\ $$$$\Rightarrow\:\:\:\:\:\:\:\:\:\:\:\frac{{t}}{\mathrm{2}}\leqslant{arcos}\left(\frac{\mathrm{1}}{\left.\mathrm{1}−\varepsilon\right)}\right. \\ $$$$\Rightarrow{t}\leqslant\mathrm{2cos}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{1}−\varepsilon}\right)=\eta_{\varepsilon} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
