Menu Close

Let-p-q-and-r-be-the-distinct-roots-of-the-polynomial-x-3-22x-2-80x-67-There-exist-real-number-A-B-and-C-such-that-1-s-3-22s-2-80s-67-A-s-p-B-s-q-C-s-r-for-all-real-numbers-

Tinku Tara 0 Comments
Pin




Question Number 135566 by bemath last updated on 14/Mar/21
Let p,q and r be the distinct roots of the polynomial x^3 −22x^2 +80x−67. There exist real number A,B and C such that (1/(s^3 −22s^2 +80s−67)) = (A/(s−p)) + (B/(s−q)) + (C/(s−r)) for all real numbers s with s ∉ {p,q,r}.What is (1/A) + (1/B) + (1/C) ? (a) 243 (b) 244 (c) 245 (d)246 (e) 247
$${Let}\:{p},{q}\:{and}\:{r}\:{be}\:{the}\:{distinct}\:{roots} \\ $$$${of}\:{the}\:{polynomial}\:{x}^{\mathrm{3}} −\mathrm{22}{x}^{\mathrm{2}} +\mathrm{80}{x}−\mathrm{67}. \\ $$$${There}\:{exist}\:{real}\:{number}\:{A},{B}\:{and} \\ $$$${C}\:{such}\:{that}\:\frac{\mathrm{1}}{{s}^{\mathrm{3}} −\mathrm{22}{s}^{\mathrm{2}} +\mathrm{80}{s}−\mathrm{67}}\:= \\ $$$$\frac{{A}}{{s}−{p}}\:+\:\frac{{B}}{{s}−{q}}\:+\:\frac{{C}}{{s}−{r}}\:{for}\:{all}\:{real}\:{numbers} \\ $$$${s}\:{with}\:{s}\:\notin\:\left\{{p},{q},{r}\right\}.{What}\:{is}\: \\ $$$$\frac{\mathrm{1}}{{A}}\:+\:\frac{\mathrm{1}}{{B}}\:+\:\frac{\mathrm{1}}{{C}}\:? \\ $$$$\left({a}\right)\:\mathrm{243}\:\:\:\:\left({b}\right)\:\mathrm{244}\:\:\:\:\left({c}\right)\:\mathrm{245}\:\:\:\left({d}\right)\mathrm{246} \\ $$$$\left({e}\right)\:\mathrm{247}\: \\ $$
Commented by EDWIN88 last updated on 14/Mar/21
very nice
$$\mathrm{very}\:\mathrm{nice} \\ $$
Answered by EDWIN88 last updated on 14/Mar/21
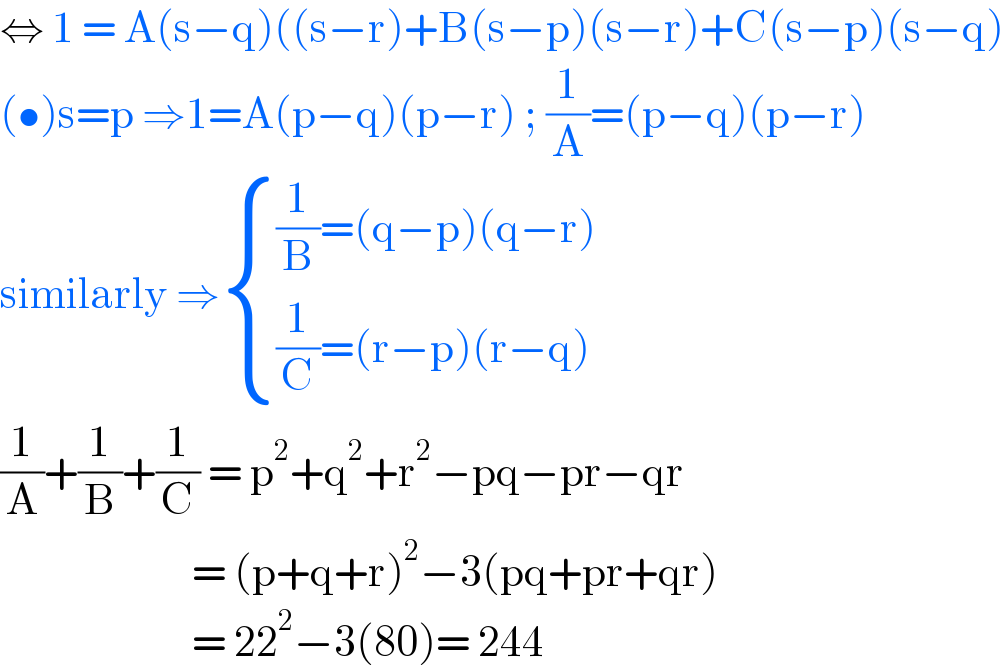
⇔ 1 = A(s−q)((s−r)+B(s−p)(s−r)+C(s−p)(s−q) (•)s=p ⇒1=A(p−q)(p−r) ; (1/A)=(p−q)(p−r) similarly ⇒ { (((1/B)=(q−p)(q−r))),(((1/C)=(r−p)(r−q))) :} (1/A)+(1/B)+(1/C) = p^2 +q^2 +r^2 −pq−pr−qr = (p+q+r)^2 −3(pq+pr+qr) = 22^2 −3(80)= 244
$$\Leftrightarrow\:\mathrm{1}\:=\:\mathrm{A}\left(\mathrm{s}−\mathrm{q}\right)\left(\left(\mathrm{s}−\mathrm{r}\right)+\mathrm{B}\left(\mathrm{s}−\mathrm{p}\right)\left(\mathrm{s}−\mathrm{r}\right)+\mathrm{C}\left(\mathrm{s}−\mathrm{p}\right)\left(\mathrm{s}−\mathrm{q}\right)\right. \\ $$$$\left(\bullet\right)\mathrm{s}=\mathrm{p}\:\Rightarrow\mathrm{1}=\mathrm{A}\left(\mathrm{p}−\mathrm{q}\right)\left(\mathrm{p}−\mathrm{r}\right)\:;\:\frac{\mathrm{1}}{\mathrm{A}}=\left(\mathrm{p}−\mathrm{q}\right)\left(\mathrm{p}−\mathrm{r}\right) \\ $$$$\mathrm{similarly}\:\Rightarrow\begin{cases}{\frac{\mathrm{1}}{\mathrm{B}}=\left(\mathrm{q}−\mathrm{p}\right)\left(\mathrm{q}−\mathrm{r}\right)}\\{\frac{\mathrm{1}}{\mathrm{C}}=\left(\mathrm{r}−\mathrm{p}\right)\left(\mathrm{r}−\mathrm{q}\right)}\end{cases} \\ $$$$\frac{\mathrm{1}}{\mathrm{A}}+\frac{\mathrm{1}}{\mathrm{B}}+\frac{\mathrm{1}}{\mathrm{C}}\:=\:\mathrm{p}^{\mathrm{2}} +\mathrm{q}^{\mathrm{2}} +\mathrm{r}^{\mathrm{2}} −\mathrm{pq}−\mathrm{pr}−\mathrm{qr} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\left(\mathrm{p}+\mathrm{q}+\mathrm{r}\right)^{\mathrm{2}} −\mathrm{3}\left(\mathrm{pq}+\mathrm{pr}+\mathrm{qr}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{22}^{\mathrm{2}} −\mathrm{3}\left(\mathrm{80}\right)=\:\mathrm{244} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *