If-ax-2-by-2-2hxy-2gx-2fy-c-0-be-the-equation-of-an-ellipse-find-coordinates-of-center-of-ellipse-Q-45506-another-solution-

Question Number 45565 by ajfour last updated on 14/Oct/18

$${If}\:\:\boldsymbol{{ax}}^{\mathrm{2}} +\boldsymbol{{by}}^{\mathrm{2}} +\mathrm{2}\boldsymbol{{hxy}}+\mathrm{2}\boldsymbol{{gx}}+\mathrm{2}\boldsymbol{{fy}}+\boldsymbol{{c}}=\mathrm{0} \\ $$$${be}\:{the}\:{equation}\:{of}\:{an}\:{ellipse},\:{find} \\ $$$${coordinates}\:{of}\:{center}\:{of}\:{ellipse}. \\ $$$${Q}.\mathrm{45506}\:\:\left({another}\:{solution}\right) \\ $$

Answered by ajfour last updated on 14/Oct/18

let centre of ellipse be (p,q). Its equation in standard orientation be (((u−p)^2 )/A^2 )+(((v−q)^2 )/B^2 )=1 when rotated by angle φ, let (u,v) → (x,y) u−p = (x−p)cos φ+(y−q)sin φ v−q = (y−q)cos φ−(x−p)sin φ Then (([(x−p)cos φ+(y−q)sin φ]^2 )/A^2 ) +(([(y−q)cos φ−(x−p)sin φ]^2 )/B^2 ) −1 = λ(ax^2 +by^2 +2hxy+2gx+2fy+c) (for all values of x and y; whether the expressions on l.h.s. and r.h.s. be equal to zero or not) let y=q_(−) , then (x−p)^2 (((cos^2 φ)/A^2 )+((sin^2 φ)/B^2 )) = λ(ax^2 +bq^2 +2hqx+2gx+2fq+c) ((coeff. of x)/(coeff. of x^2 )) = ((−2p)/1) = ((2(hq+g))/a) ⇒ ap+hq = −g ....(i) similarly if x=p_(−) , then (y−q)^2 (((sin^2 φ)/A^2 )+((cos^2 φ)/B^2 )) = λ(ap^2 +by^2 +2hpy+2gp+2fy+c) ((coeff. of y)/(coeff. of y^2 )) = ((−2q)/1) = ((2(hp+f))/b) ⇒ hp+bq = −f ....(ii) Now solving (i) & (ii) for p,q { ((ap+hq = −g )),((hp+bq = −f )) :} p = ((fh−bg)/(ab−h^2 )) ; q = ((gh−af)/(ab−h^2 )) .

$${let}\:{centre}\:{of}\:{ellipse}\:{be}\:\left(\boldsymbol{{p}},\boldsymbol{{q}}\right). \\ $$$${Its}\:{equation}\:{in}\:{standard}\: \\ $$$${orientation}\:{be}\:\:\frac{\left({u}−{p}\right)^{\mathrm{2}} }{{A}^{\mathrm{2}} }+\frac{\left({v}−{q}\right)^{\mathrm{2}} }{{B}^{\mathrm{2}} }=\mathrm{1} \\ $$$${when}\:{rotated}\:{by}\:{angle}\:\phi, \\ $$$${let}\:\:\left({u},{v}\right)\:\rightarrow\:\left({x},{y}\right) \\ $$$$\:\:\:{u}−{p}\:=\:\left({x}−{p}\right)\mathrm{cos}\:\phi+\left({y}−{q}\right)\mathrm{sin}\:\phi \\ $$$$\:\:\:{v}−{q}\:=\:\left({y}−{q}\right)\mathrm{cos}\:\phi−\left({x}−{p}\right)\mathrm{sin}\:\phi \\ $$$${Then} \\ $$$$\frac{\left[\left({x}−{p}\right)\mathrm{cos}\:\phi+\left({y}−{q}\right)\mathrm{sin}\:\phi\right]^{\mathrm{2}} }{{A}^{\mathrm{2}} }\: \\ $$$$\:\:\:\:\:+\frac{\left[\left({y}−{q}\right)\mathrm{cos}\:\phi−\left({x}−{p}\right)\mathrm{sin}\:\phi\right]^{\mathrm{2}} }{{B}^{\mathrm{2}} }\:−\mathrm{1} \\ $$$$\:\:=\:\lambda\left({ax}^{\mathrm{2}} +{by}^{\mathrm{2}} +\mathrm{2}{hxy}+\mathrm{2}{gx}+\mathrm{2}{fy}+{c}\right) \\ $$$$ \\ $$$$\left({for}\:{all}\:{values}\:{of}\:{x}\:{and}\:{y};\:{whether}\right. \\ $$$${the}\:{expressions}\:{on}\:{l}.{h}.{s}.\:{and}\:{r}.{h}.{s}. \\ $$$$\left.{be}\:{equal}\:{to}\:{zero}\:{or}\:{not}\right) \\ $$$${let}\:\:\:\underset{−} {\boldsymbol{{y}}=\boldsymbol{{q}}}\:\:\:,\:\:{then} \\ $$$$\left({x}−{p}\right)^{\mathrm{2}} \left(\frac{\mathrm{cos}\:^{\mathrm{2}} \phi}{{A}^{\mathrm{2}} }+\frac{\mathrm{sin}\:^{\mathrm{2}} \phi}{{B}^{\mathrm{2}} }\right) \\ $$$$\:\:\:=\:\lambda\left({ax}^{\mathrm{2}} +{bq}^{\mathrm{2}} +\mathrm{2}{hqx}+\mathrm{2}{gx}+\mathrm{2}{fq}+{c}\right) \\ $$$$ \\ $$$$\frac{{coeff}.\:{of}\:{x}}{{coeff}.\:{of}\:{x}^{\mathrm{2}} }\:=\:\frac{−\mathrm{2}{p}}{\mathrm{1}}\:=\:\frac{\mathrm{2}\left({hq}+{g}\right)}{{a}} \\ $$$$\Rightarrow\:\:\:\:\:\:\boldsymbol{{ap}}+\boldsymbol{{hq}}\:=\:−\boldsymbol{{g}}\:\:\:….\left(\boldsymbol{{i}}\right) \\ $$$${similarly}\:\:{if}\:\underset{−} {\boldsymbol{{x}}=\boldsymbol{{p}}}\:,\:{then} \\ $$$$\:\left({y}−{q}\right)^{\mathrm{2}} \left(\frac{\mathrm{sin}\:^{\mathrm{2}} \phi}{{A}^{\mathrm{2}} }+\frac{\mathrm{cos}\:^{\mathrm{2}} \phi}{{B}^{\mathrm{2}} }\right) \\ $$$$\:\:\:\:=\:\lambda\left({ap}^{\mathrm{2}} +{by}^{\mathrm{2}} +\mathrm{2}{hpy}+\mathrm{2}{gp}+\mathrm{2}{fy}+{c}\right) \\ $$$$ \\ $$$$\frac{{coeff}.\:{of}\:{y}}{{coeff}.\:{of}\:{y}^{\mathrm{2}} }\:=\:\frac{−\mathrm{2}{q}}{\mathrm{1}}\:=\:\frac{\mathrm{2}\left({hp}+{f}\right)}{{b}} \\ $$$$\Rightarrow\:\:\:\:\:\:\:\:\:\boldsymbol{{hp}}+\boldsymbol{{bq}}\:=\:−\boldsymbol{{f}}\:\:\:\:\:….\left(\boldsymbol{{ii}}\right) \\ $$$${Now}\:{solving}\:\left({i}\right)\:\&\:\left({ii}\right)\:{for}\:\boldsymbol{{p}},\boldsymbol{{q}} \\ $$$$\:\begin{cases}{\boldsymbol{{ap}}+\boldsymbol{{hq}}\:=\:−\boldsymbol{{g}}\:\:}\\{\boldsymbol{{hp}}+\boldsymbol{{bq}}\:=\:−\boldsymbol{{f}}\:\:}\end{cases} \\ $$$$\:\:\:\:\:\:\:\boldsymbol{{p}}\:=\:\frac{\boldsymbol{{fh}}−\boldsymbol{{bg}}}{\boldsymbol{{ab}}−\boldsymbol{{h}}^{\mathrm{2}} }\:\:;\:\:\:\boldsymbol{{q}}\:=\:\frac{\boldsymbol{{gh}}−\boldsymbol{{af}}}{\boldsymbol{{ab}}−\boldsymbol{{h}}^{\mathrm{2}} }\:\:. \\ $$$$\:\:\:\:\:\: \\ $$

Commented by ajfour last updated on 14/Oct/18

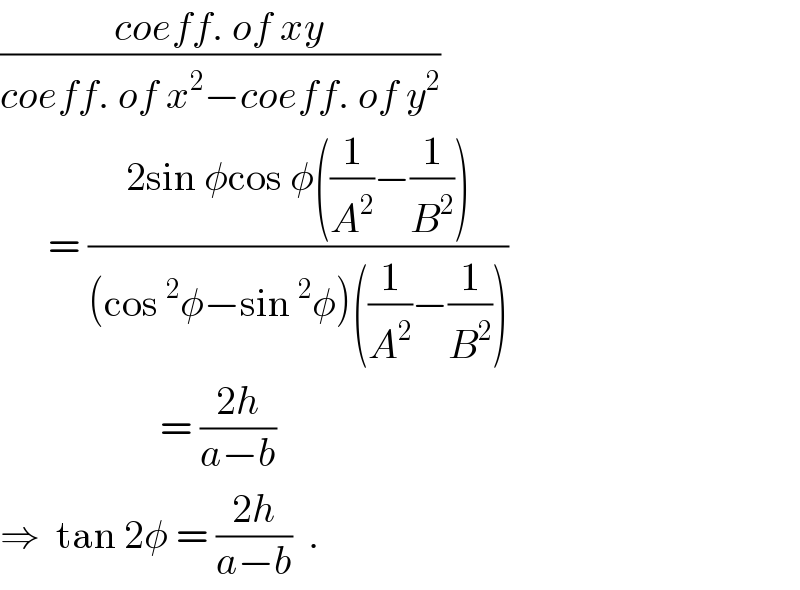
((coeff. of xy)/(coeff. of x^2 −coeff. of y^2 )) = ((2sin φcos φ((1/A^2 )−(1/B^2 )))/((cos^2 φ−sin^2 φ)((1/A^2 )−(1/B^2 )))) = ((2h)/(a−b)) ⇒ tan 2φ = ((2h)/(a−b)) .

$$\frac{{coeff}.\:{of}\:{xy}}{{coeff}.\:{of}\:{x}^{\mathrm{2}} −{coeff}.\:{of}\:{y}^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\:=\:\frac{\mathrm{2sin}\:\phi\mathrm{cos}\:\phi\left(\frac{\mathrm{1}}{{A}^{\mathrm{2}} }−\frac{\mathrm{1}}{{B}^{\mathrm{2}} }\right)}{\left(\mathrm{cos}\:^{\mathrm{2}} \phi−\mathrm{sin}\:^{\mathrm{2}} \phi\right)\left(\frac{\mathrm{1}}{{A}^{\mathrm{2}} }−\frac{\mathrm{1}}{{B}^{\mathrm{2}} }\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\frac{\mathrm{2}{h}}{{a}−{b}}\:\: \\ $$$$\Rightarrow\:\:\mathrm{tan}\:\mathrm{2}\phi\:=\:\frac{\mathrm{2}{h}}{{a}−{b}}\:\:. \\ $$

Commented by MrW3 last updated on 14/Oct/18

$${thanks}\:{a}\:{lot}\:{sir}! \\ $$

Answered by MJS last updated on 14/Oct/18

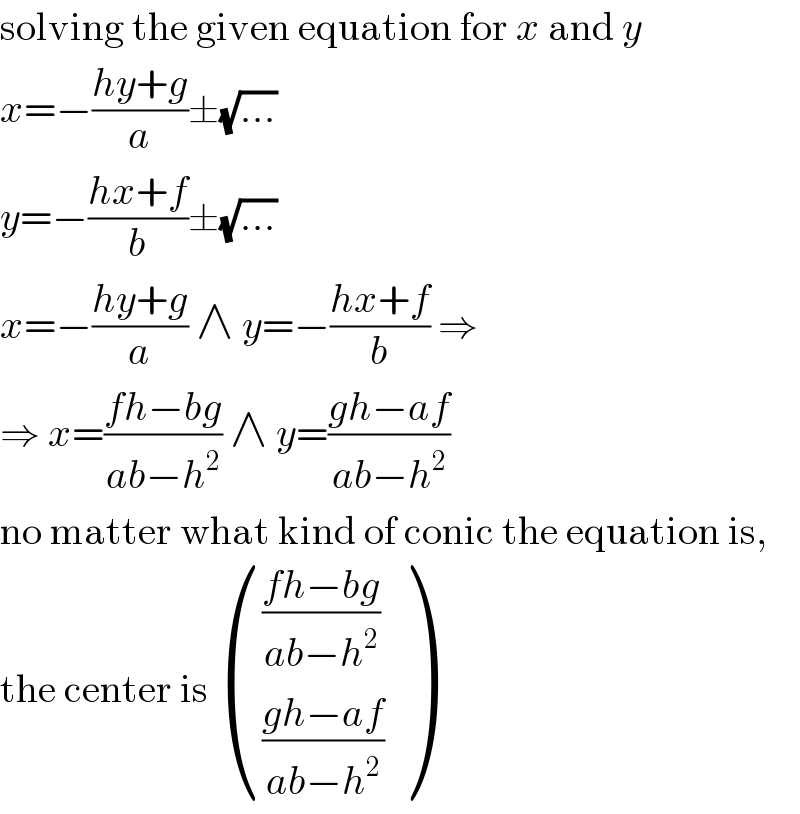
solving the given equation for x and y x=−((hy+g)/a)±(√(...)) y=−((hx+f)/b)±(√(...)) x=−((hy+g)/a) ∧ y=−((hx+f)/b) ⇒ ⇒ x=((fh−bg)/(ab−h^2 )) ∧ y=((gh−af)/(ab−h^2 )) no matter what kind of conic the equation is, the center is ((((fh−bg)/(ab−h^2 ))),(((gh−af)/(ab−h^2 ))) )

$$\mathrm{solving}\:\mathrm{the}\:\mathrm{given}\:\mathrm{equation}\:\mathrm{for}\:{x}\:\mathrm{and}\:{y} \\ $$$${x}=−\frac{{hy}+{g}}{{a}}\pm\sqrt{…} \\ $$$${y}=−\frac{{hx}+{f}}{{b}}\pm\sqrt{…} \\ $$$${x}=−\frac{{hy}+{g}}{{a}}\:\wedge\:{y}=−\frac{{hx}+{f}}{{b}}\:\Rightarrow \\ $$$$\Rightarrow\:{x}=\frac{{fh}−{bg}}{{ab}−{h}^{\mathrm{2}} }\:\wedge\:{y}=\frac{{gh}−{af}}{{ab}−{h}^{\mathrm{2}} } \\ $$$$\mathrm{no}\:\mathrm{matter}\:\mathrm{what}\:\mathrm{kind}\:\mathrm{of}\:\mathrm{conic}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{is}, \\ $$$$\mathrm{the}\:\mathrm{center}\:\mathrm{is}\:\begin{pmatrix}{\frac{{fh}−{bg}}{{ab}−{h}^{\mathrm{2}} }}\\{\frac{{gh}−{af}}{{ab}−{h}^{\mathrm{2}} }}\end{pmatrix} \\ $$

Commented by ajfour last updated on 14/Oct/18