Question Number 177353 by HeferH last updated on 04/Oct/22

Commented by HeferH last updated on 04/Oct/22

$$\left.:\right) \\ $$
Answered by BaliramKumar last updated on 04/Oct/22

$$\frac{\mathrm{3}}{\mathrm{13}}\:??? \\ $$
Commented by mr W last updated on 04/Oct/22
$${yes}!\:{but}\:{how}\:{did}\:{you}\:{get}\:{that}? \\ $$
Commented by BaliramKumar last updated on 04/Oct/22
$${similar}\:{triangle}\:\&\:{cosine}\:{rule}\: \\ $$$${see}\:{my}\:{solution}\downdownarrows \\ $$
Answered by mr W last updated on 04/Oct/22

Commented by mr W last updated on 04/Oct/22
![R=radius s=(√3)R sin α=(x/(2R)) sin β=((3x)/(2R)) α+β=(π/3) cos (α+β)=cos (π/3)=(1/2) (√((1−(x^2 /(4R^2 )))(1−((9x^2 )/(4R^2 )))))−(x/(2R))×((3x)/(2R))=(1/2) (√((4R^2 −x^2 )(4R^2 −9x^2 )))=2R^2 +3x^2 16R^4 −40R^2 x^2 +9x^4 =4R^4 +12R^2 x^2 +9x^4 3R^2 =13x^2 ⇒x=(√(3/(13)))R sin β=((3x)/(2R))=3(√(3/(52))) ⇒cos β=(5/( (√(52)))) (b/s)=((sin (π/3))/(sin ((π/3)+β))) ((a+b)/s)=((sin ((π/3)+β))/(sin (π/3))) ((a+b)/b)=[((sin ((π/3)+β))/(sin (π/3)))]^2 =[cos β+((sin β)/(tan (π/3)))]^2 (a/b)=[cos β+((sin β)/(tan (π/3)))]^2 −1 =[(5/( (√(52))))+(3/( (√3)))(√(3/(52)))]^2 −1 =((8/( (√(52)))))^2 −1=(3/(13)) ✓](https://www.tinkutara.com/question/Q177369.png)
$${R}={radius} \\ $$$${s}=\sqrt{\mathrm{3}}{R} \\ $$$$\mathrm{sin}\:\alpha=\frac{{x}}{\mathrm{2}{R}} \\ $$$$\mathrm{sin}\:\beta=\frac{\mathrm{3}{x}}{\mathrm{2}{R}} \\ $$$$\alpha+\beta=\frac{\pi}{\mathrm{3}} \\ $$$$\mathrm{cos}\:\left(\alpha+\beta\right)=\mathrm{cos}\:\frac{\pi}{\mathrm{3}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\sqrt{\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{4}{R}^{\mathrm{2}} }\right)\left(\mathrm{1}−\frac{\mathrm{9}{x}^{\mathrm{2}} }{\mathrm{4}{R}^{\mathrm{2}} }\right)}−\frac{{x}}{\mathrm{2}{R}}×\frac{\mathrm{3}{x}}{\mathrm{2}{R}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\sqrt{\left(\mathrm{4}{R}^{\mathrm{2}} −{x}^{\mathrm{2}} \right)\left(\mathrm{4}{R}^{\mathrm{2}} −\mathrm{9}{x}^{\mathrm{2}} \right)}=\mathrm{2}{R}^{\mathrm{2}} +\mathrm{3}{x}^{\mathrm{2}} \\ $$$$\mathrm{16}{R}^{\mathrm{4}} −\mathrm{40}{R}^{\mathrm{2}} {x}^{\mathrm{2}} +\mathrm{9}{x}^{\mathrm{4}} =\mathrm{4}{R}^{\mathrm{4}} +\mathrm{12}{R}^{\mathrm{2}} {x}^{\mathrm{2}} +\mathrm{9}{x}^{\mathrm{4}} \\ $$$$\mathrm{3}{R}^{\mathrm{2}} =\mathrm{13}{x}^{\mathrm{2}} \\ $$$$\Rightarrow{x}=\sqrt{\frac{\mathrm{3}}{\mathrm{13}}}{R} \\ $$$$\mathrm{sin}\:\beta=\frac{\mathrm{3}{x}}{\mathrm{2}{R}}=\mathrm{3}\sqrt{\frac{\mathrm{3}}{\mathrm{52}}}\:\Rightarrow\mathrm{cos}\:\beta=\frac{\mathrm{5}}{\:\sqrt{\mathrm{52}}} \\ $$$$\frac{{b}}{{s}}=\frac{\mathrm{sin}\:\frac{\pi}{\mathrm{3}}}{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{3}}+\beta\right)} \\ $$$$\frac{{a}+{b}}{{s}}=\frac{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{3}}+\beta\right)}{\mathrm{sin}\:\frac{\pi}{\mathrm{3}}} \\ $$$$\frac{{a}+{b}}{{b}}=\left[\frac{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{3}}+\beta\right)}{\mathrm{sin}\:\frac{\pi}{\mathrm{3}}}\right]^{\mathrm{2}} =\left[\mathrm{cos}\:\beta+\frac{\mathrm{sin}\:\beta}{\mathrm{tan}\:\frac{\pi}{\mathrm{3}}}\right]^{\mathrm{2}} \\ $$$$\frac{{a}}{{b}}=\left[\mathrm{cos}\:\beta+\frac{\mathrm{sin}\:\beta}{\mathrm{tan}\:\frac{\pi}{\mathrm{3}}}\right]^{\mathrm{2}} −\mathrm{1} \\ $$$$\:\:\:\:=\left[\frac{\mathrm{5}}{\:\sqrt{\mathrm{52}}}+\frac{\mathrm{3}}{\:\sqrt{\mathrm{3}}}\sqrt{\frac{\mathrm{3}}{\mathrm{52}}}\right]^{\mathrm{2}} −\mathrm{1} \\ $$$$\:\:\:\:=\left(\frac{\mathrm{8}}{\:\sqrt{\mathrm{52}}}\right)^{\mathrm{2}} −\mathrm{1}=\frac{\mathrm{3}}{\mathrm{13}}\:\checkmark \\ $$
Commented by Tawa11 last updated on 04/Oct/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
Answered by BaliramKumar last updated on 04/Oct/22

Commented by BaliramKumar last updated on 04/Oct/22

Commented by mr W last updated on 04/Oct/22

$${nice}\:{approach}! \\ $$
Commented by BaliramKumar last updated on 04/Oct/22

$${thanks}\:{sir} \\ $$
Commented by HeferH last updated on 04/Oct/22

$${Looks}\:{nice},\:{I}\:{did}\:{it}\:{by}\:{pure}\:{Similar}\: \\ $$$$\:{triangles} \\ $$
Answered by HeferH last updated on 04/Oct/22

Commented by HeferH last updated on 04/Oct/22
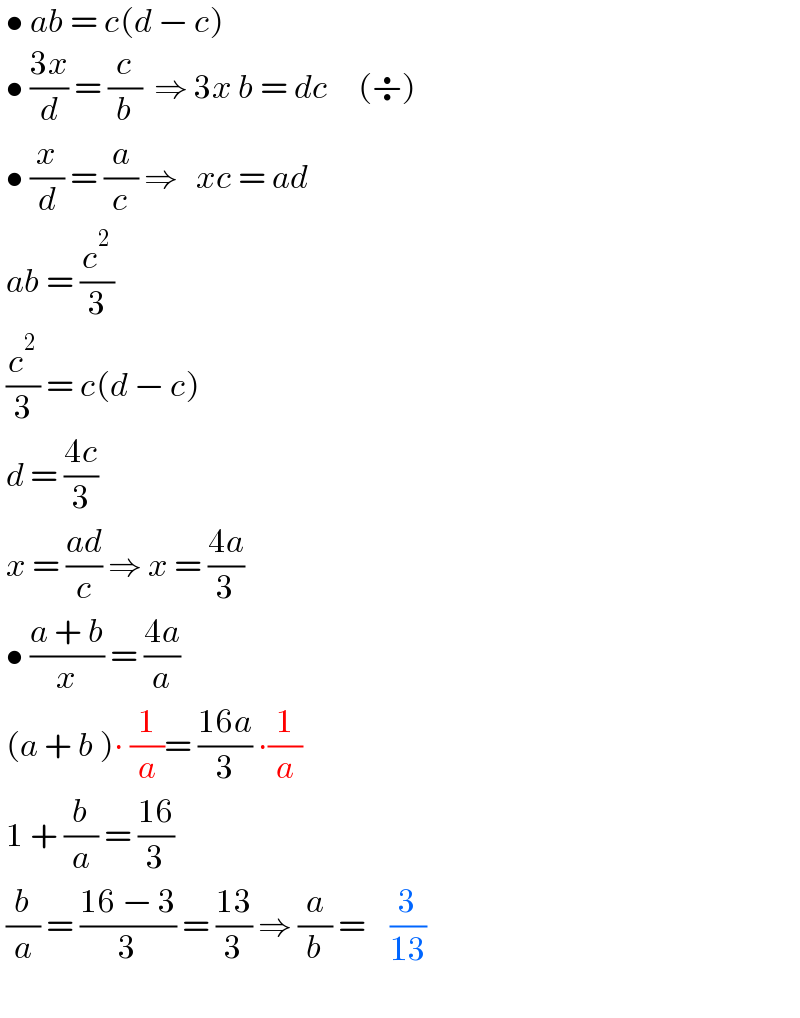
$$\:\bullet\:{ab}\:=\:{c}\left({d}\:−\:{c}\right) \\ $$$$\:\bullet\:\frac{\mathrm{3}{x}}{{d}}\:=\:\frac{{c}}{{b}}\:\:\Rightarrow\:\mathrm{3}{x}\:{b}\:=\:{dc}\:\:\:\:\:\left(\boldsymbol{\div}\right) \\ $$$$\:\bullet\:\frac{{x}}{{d}}\:=\:\frac{{a}}{{c}}\:\Rightarrow\:\:\:{xc}\:=\:{ad} \\ $$$$\:{ab}\:=\:\frac{{c}^{\mathrm{2}} }{\mathrm{3}} \\ $$$$\:\frac{{c}^{\mathrm{2}} }{\mathrm{3}}\:=\:{c}\left({d}\:−\:{c}\right) \\ $$$$\:{d}\:=\:\frac{\mathrm{4}{c}}{\mathrm{3}} \\ $$$$\:{x}\:=\:\frac{{ad}}{{c}}\:\Rightarrow\:{x}\:=\:\frac{\mathrm{4}{a}}{\mathrm{3}} \\ $$$$\:\bullet\:\frac{{a}\:+\:{b}}{{x}}\:=\:\frac{\mathrm{4}{a}}{{a}} \\ $$$$\:\left({a}\:+\:{b}\:\right)\centerdot\:\frac{\mathrm{1}}{{a}}=\:\frac{\mathrm{16}{a}}{\mathrm{3}}\:\centerdot\frac{\mathrm{1}}{{a}} \\ $$$$\:\mathrm{1}\:+\:\frac{{b}}{{a}}\:=\:\frac{\mathrm{16}}{\mathrm{3}} \\ $$$$\:\frac{{b}}{{a}}\:=\:\frac{\mathrm{16}\:−\:\mathrm{3}}{\mathrm{3}}\:=\:\frac{\mathrm{13}}{\mathrm{3}}\:\Rightarrow\:\frac{{a}}{{b}}\:=\:\:\:\:\frac{\mathrm{3}}{\mathrm{13}} \\ $$$$\: \\ $$
Commented by Tawa11 last updated on 04/Oct/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
