Question Number 177375 by a.lgnaoui last updated on 04/Oct/22

$$\mathrm{soit}\:\mathrm{k}\:\mathrm{un}\:\mathrm{entier}\:\mathrm{naturel}\:\mathrm{non}\:\mathrm{nul}\:,\mathrm{S}\:\mathrm{un}\:\mathrm{nombre}\:\mathrm{fini}\:\mathrm{de}\: \\ $$$$\mathrm{nombres}\:\mathrm{premiers}\:\mathrm{impair} \\ $$$$−\mathrm{Demontrer}\:\mathrm{qu}\:\mathrm{il}\:\mathrm{existe}\:\mathrm{au}\:\mathrm{plus}\:\mathrm{une}\:\mathrm{maniere}\left(\mathrm{a}\:\mathrm{rotation}\:\mathrm{et}\:\mathrm{symetrie}\:\mathrm{axiale}\right) \\ $$$$\mathrm{de}\:\mathrm{disposer}\:\mathrm{les}\:\mathrm{elements}\:\mathrm{de}\:\mathrm{S}\:\mathrm{sur}\:\mathrm{un}\:\mathrm{cercle}\:\mathrm{de}\:\mathrm{sorte}\:\mathrm{que}\:\mathrm{le}\:\mathrm{priduit}\:\mathrm{de}\:\mathrm{2}\:\mathrm{nombres}\: \\ $$$$\mathrm{places}\:\mathrm{l}'\mathrm{un}\:\mathrm{a}\:\mathrm{cote}\:\mathrm{de}\:\mathrm{l}'\mathrm{autre}\:\mathrm{soit}\:\mathrm{toujours}\:\mathrm{de}\:\mathrm{la}\:\mathrm{forme}\:\left(\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\boldsymbol{\mathrm{x}}+\boldsymbol{\mathrm{k}}\right),\mathrm{x}\:\mathrm{un}\:\mathrm{entier}\:\mathrm{naturel}\:\mathrm{non}\:\mathrm{nul}. \\ $$$$\:\:\:\:\:\:\:{Extrait}\:{de}\:{Olimpiad}\:{de}\:{Mathematiques}\:\left({France}\:\mathrm{2022}\right). \\ $$$$ \\ $$
Commented by a.lgnaoui last updated on 04/Oct/22

Commented by TheHoneyCat last updated on 04/Oct/22
Note pour les gens qui (comme moi) auraient mal lu:
Ya écrit, "il existe AU PLUS".
Sinon, ce serait évidemment faux (ex: k=1; S={3,5})
Commented by a.lgnaoui last updated on 04/Oct/22
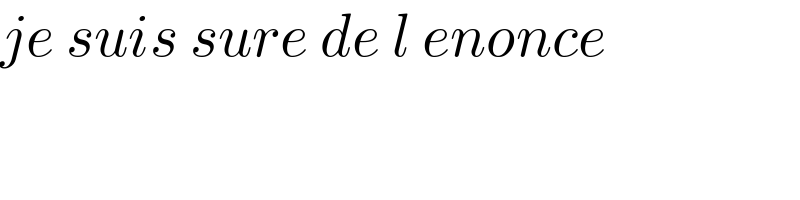
$${je}\:{suis}\:{sure}\:{de}\:{l}\:{enonce}\: \\ $$
Commented by a.lgnaoui last updated on 04/Oct/22

Commented by TheHoneyCat last updated on 04/Oct/22
je n'en doute pas.
J'ai juste mal lu, et ça m'a fait perdre du temps, donc je précise pour autrui.
btw j'ai passé 2h dessus mais j'ai pas trouvé.
donc bravo à ceux qui ont trouvé.
