Menu Close

a-If-tan-1-a-tan-1-b-tan-1-c-pi-show-that-a-b-c-abc-1-b-If-tanx-nsinycosy-1-nsin-2-y-show-that-tan-y-x-1-n-tany-

Tinku Tara 0 Comments
Pin




Question Number 46474 by peter frank last updated on 28/Oct/18
a)[If tan^(−1) a+tan^(−1) b+tan^(−1) c=π show that ((a+b+c)/(abc))=1 b)[ If tanx=((nsinycosy)/(1−nsin^2 y)) show that tan(y−x)=(1−n)tany
$$\left.\mathrm{a}\right)\left[\mathrm{If}\:\mathrm{tan}^{−\mathrm{1}} \mathrm{a}+\mathrm{tan}^{−\mathrm{1}} \mathrm{b}+\mathrm{tan}^{−\mathrm{1}} \mathrm{c}=\pi\:\:\mathrm{show}\:\mathrm{that}\:\frac{\mathrm{a}+\mathrm{b}+\mathrm{c}}{\mathrm{abc}}=\mathrm{1}\right. \\ $$$$\left.\mathrm{b}\right)\left[\:\:\mathrm{If}\:\:\mathrm{tanx}=\frac{\mathrm{nsinycosy}}{\mathrm{1}−\mathrm{nsin}^{\mathrm{2}} \mathrm{y}}\right. \\ $$$$\mathrm{show}\:\mathrm{that}\:\mathrm{tan}\left(\mathrm{y}−\mathrm{x}\right)=\left(\mathrm{1}−\mathrm{n}\right)\mathrm{tany} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by math1967 last updated on 27/Oct/18
pls.check q(a)
$${pls}.{check}\:{q}\left({a}\right) \\ $$
Commented by peter frank last updated on 28/Oct/18
ready sir
$$\mathrm{ready}\:\mathrm{sir} \\ $$
Answered by math1967 last updated on 27/Oct/18
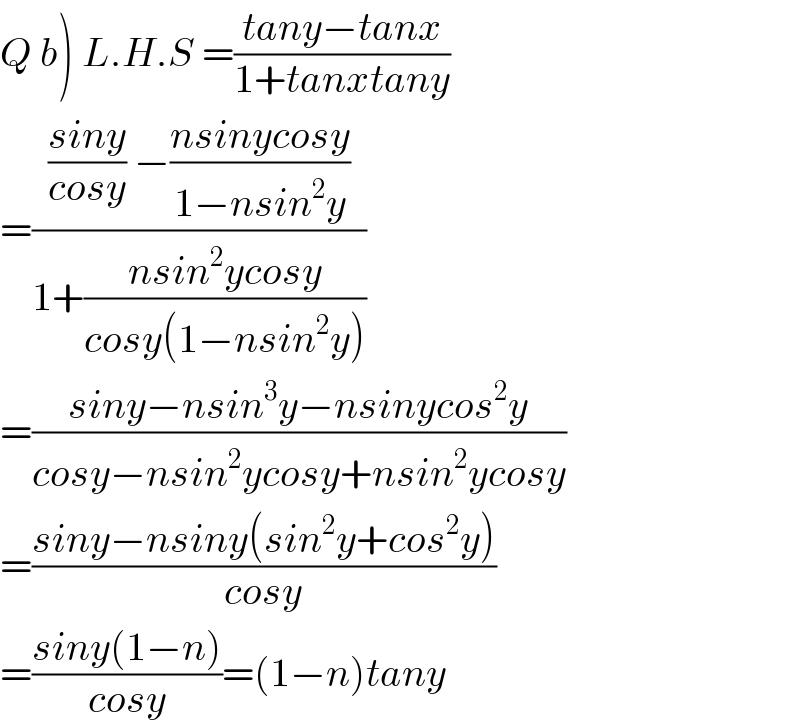
Q b) L.H.S =((tany−tanx)/(1+tanxtany)) =((((siny)/(cosy)) −((nsinycosy)/(1−nsin^2 y)))/(1+((nsin^2 ycosy)/(cosy(1−nsin^2 y))))) =((siny−nsin^3 y−nsinycos^2 y)/(cosy−nsin^2 ycosy+nsin^2 ycosy)) =((siny−nsiny(sin^2 y+cos^2 y))/(cosy)) =((siny(1−n))/(cosy))=(1−n)tany
$$\left.{Q}\:{b}\right)\:{L}.{H}.{S}\:=\frac{{tany}−{tanx}}{\mathrm{1}+{tanxtany}} \\ $$$$=\frac{\frac{{siny}}{{cosy}}\:−\frac{{nsinycosy}}{\mathrm{1}−{nsin}^{\mathrm{2}} {y}}}{\mathrm{1}+\frac{{nsin}^{\mathrm{2}} {ycosy}}{{cosy}\left(\mathrm{1}−{nsin}^{\mathrm{2}} {y}\right)}} \\ $$$$=\frac{{siny}−{nsin}^{\mathrm{3}} {y}−{nsinycos}^{\mathrm{2}} {y}}{{cosy}−{nsin}^{\mathrm{2}} {ycosy}+{nsin}^{\mathrm{2}} {ycosy}} \\ $$$$=\frac{{siny}−{nsiny}\left({sin}^{\mathrm{2}} {y}+{cos}^{\mathrm{2}} {y}\right)}{{cosy}}\:\: \\ $$$$=\frac{{siny}\left(\mathrm{1}−{n}\right)}{{cosy}}=\left(\mathrm{1}−{n}\right){tany} \\ $$
Answered by math1967 last updated on 28/Oct/18
lettan^(−1) a=α, tan^(−1) b=β ,tan^(−1) c=γ a=tan α ,b=tan β,c=tan γ Again α+β+γ=π α+β=π−γ tan (α+β)=tan (π−γ) ((tan α+tan β)/(1−tan αtan β))=−tan γ tan α+tan β=−tan γ+tan αtan βtan γ ∴a+b+c=abc ∴((a+b+c)/(abc))=1 proved
$${let}\mathrm{tan}^{−\mathrm{1}} {a}=\alpha,\:\mathrm{tan}^{−\mathrm{1}} {b}=\beta\:,\mathrm{tan}^{−\mathrm{1}} {c}=\gamma \\ $$$$\:{a}=\mathrm{tan}\:\alpha\:,{b}=\mathrm{tan}\:\beta,{c}=\mathrm{tan}\:\gamma \\ $$$${Again}\:\alpha+\beta+\gamma=\pi \\ $$$$\:\alpha+\beta=\pi−\gamma \\ $$$$\mathrm{tan}\:\left(\alpha+\beta\right)=\mathrm{tan}\:\left(\pi−\gamma\right) \\ $$$$\frac{\mathrm{tan}\:\alpha+\mathrm{tan}\:\beta}{\mathrm{1}−\mathrm{tan}\:\alpha\mathrm{tan}\:\beta}=−\mathrm{tan}\:\gamma \\ $$$$\mathrm{tan}\:\alpha+\mathrm{tan}\:\beta=−\mathrm{tan}\:\gamma+\mathrm{tan}\:\alpha\mathrm{tan}\:\beta\mathrm{tan}\:\gamma \\ $$$$\therefore{a}+{b}+{c}={abc} \\ $$$$\therefore\frac{{a}+{b}+{c}}{{abc}}=\mathrm{1}\:{proved} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *