Question-113219

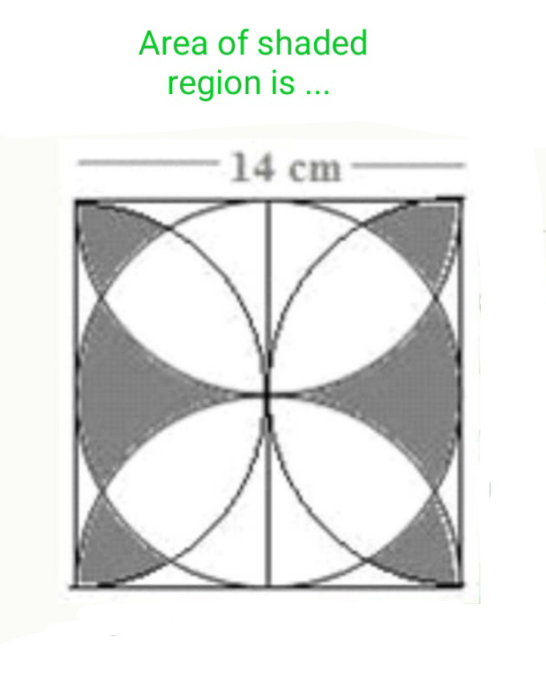
Question Number 113219 by naka3546 last updated on 11/Sep/20

Answered by 1549442205PVT last updated on 11/Sep/20

Commented by Aina Samuel Temidayo last updated on 11/Sep/20

Please how do you construct these things?

$$\mathrm{Please}\:\mathrm{how}\:\mathrm{do}\:\mathrm{you}\:\mathrm{construct}\:\mathrm{these} \\ $$$$\mathrm{things}? \\ $$

Commented by 1549442205PVT last updated on 12/Sep/20

It is easy to see that FK^(⌢) =KL^(⌢) =LG^(⌢) =GN^(⌢) =NJ^(⌢) =JE^(⌢) =(π/6)⇒FEK^(�) =15° The area of the sector IKF is S_(sectorIKF) =((πR^2 )/(12))=((49π)/(12)).The are of the equalateral triangle IKG is S_(ΔIKG) =((R^2 (√3))/4)=((49(√3))/4).The area of curve triangle DKF equal to S_(DGIF) −2.S_(sector) −S_(ΔIKG) =49−((49π)/6)−((49(√3))/4)(1) .The area of the sector ILM is S_(sectorILM) =((πR^2 )/3)=((49π)/3)(since LFM^(⌢) =120°) S_(ΔILM) =S_(ΔIKG) =((49(√3))/4).Hence,the area of part is limited by the LIM^(⌢) and the arc LFM^(⌢) is 2(S_(sectorILM) −S_(ΔILM) ) =2(((49π)/3)−((49(√3))/4))(2) The are of eight curve white triangle are S_1 =8(49−((49π)/6)−((49(√3))/4))(3).The area of two big white region are S_2 =4(((49π)/3)−((49(√3))/4)) Consequently,the area of is shaded region equal to S_(squareABCD) −(S_1 +S_2 ) =14^2 −8(49−((49π)/6)−((49(√3))/4))−4(((49π)/3)−((49(√3))/4)) =3.49(√3)−196≈58.61

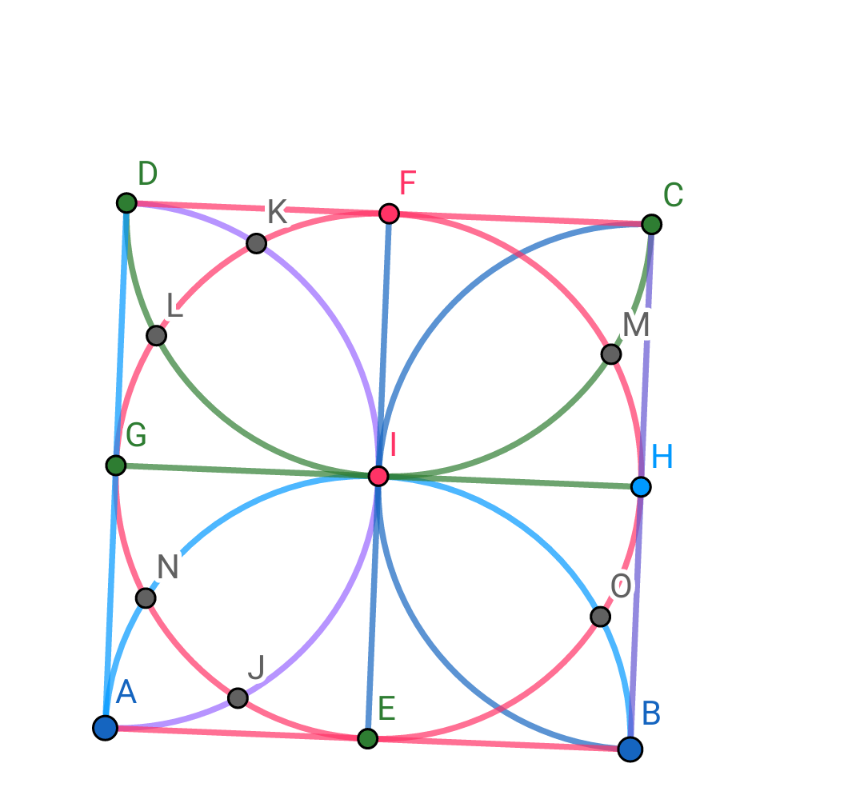
$$\mathrm{It}\:\mathrm{is}\:\mathrm{easy}\:\mathrm{to}\:\mathrm{see}\:\mathrm{that} \\ $$$$\:\overset{\frown} {\mathrm{FK}}=\overset{\frown} {\mathrm{KL}}=\overset{\frown} {\mathrm{LG}}=\overset{\frown} {\mathrm{GN}}=\overset{\frown} {\mathrm{NJ}}=\overset{\frown} {\mathrm{JE}} \\ $$$$=\frac{\pi}{\mathrm{6}}\Rightarrow\widehat {\mathrm{FEK}}=\mathrm{15}° \\ $$$$\mathrm{The}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{sector}\:\mathrm{IKF}\:\mathrm{is} \\ $$$$\mathrm{S}_{\mathrm{sectorIKF}} =\frac{\pi\mathrm{R}^{\mathrm{2}} }{\mathrm{12}}=\frac{\mathrm{49}\pi}{\mathrm{12}}.\mathrm{The}\:\mathrm{are}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{equalateral}\:\mathrm{triangle}\:\mathrm{IKG}\:\mathrm{is} \\ $$$$\mathrm{S}_{\Delta\mathrm{IKG}} =\frac{\mathrm{R}^{\mathrm{2}} \sqrt{\mathrm{3}}}{\mathrm{4}}=\frac{\mathrm{49}\sqrt{\mathrm{3}}}{\mathrm{4}}.\mathrm{The}\:\mathrm{area}\:\mathrm{of}\:\mathrm{curve} \\ $$$$\mathrm{triangle}\:\mathrm{DKF}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{S}_{\mathrm{DGIF}} −\mathrm{2}.\mathrm{S}_{\mathrm{sector}} \\ $$$$−\mathrm{S}_{\Delta\mathrm{IKG}} =\mathrm{49}−\frac{\mathrm{49}\pi}{\mathrm{6}}−\frac{\mathrm{49}\sqrt{\mathrm{3}}}{\mathrm{4}}\left(\mathrm{1}\right) \\ $$$$.\mathrm{The}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{sector} \\ $$$$\mathrm{ILM}\:\mathrm{is}\:\mathrm{S}_{\mathrm{sectorILM}} =\frac{\pi\mathrm{R}^{\mathrm{2}} }{\mathrm{3}}=\frac{\mathrm{49}\pi}{\mathrm{3}}\left(\mathrm{since}\:\overset{\frown} {\mathrm{LFM}}=\mathrm{120}°\right) \\ $$$$\mathrm{S}_{\Delta\mathrm{ILM}} =\mathrm{S}_{\Delta\mathrm{IKG}} =\frac{\mathrm{49}\sqrt{\mathrm{3}}}{\mathrm{4}}.\mathrm{Hence},\mathrm{the}\:\mathrm{area} \\ $$$$\mathrm{of}\:\mathrm{part}\:\mathrm{is}\:\mathrm{limited}\:\mathrm{by}\:\mathrm{the}\:\overset{\frown} {\mathrm{LIM}}\:\mathrm{and} \\ $$$$\mathrm{the}\:\mathrm{arc}\:\overset{\frown} {\mathrm{LFM}}\:\mathrm{is}\:\mathrm{2}\left(\mathrm{S}_{\mathrm{sectorILM}} −\mathrm{S}_{\Delta\mathrm{ILM}} \right) \\ $$$$=\mathrm{2}\left(\frac{\mathrm{49}\pi}{\mathrm{3}}−\frac{\mathrm{49}\sqrt{\mathrm{3}}}{\mathrm{4}}\right)\left(\mathrm{2}\right) \\ $$$$\mathrm{The}\:\mathrm{are}\:\mathrm{of}\:\mathrm{eight}\:\mathrm{curve}\:\mathrm{white}\:\mathrm{triangle}\:\mathrm{are} \\ $$$$\mathrm{S}_{\mathrm{1}} =\mathrm{8}\left(\mathrm{49}−\frac{\mathrm{49}\pi}{\mathrm{6}}−\frac{\mathrm{49}\sqrt{\mathrm{3}}}{\mathrm{4}}\right)\left(\mathrm{3}\right).\mathrm{The}\:\mathrm{area}\:\mathrm{of}\:\mathrm{two}\: \\ $$$$\mathrm{big}\:\mathrm{white}\:\mathrm{region}\:\mathrm{are}\:\mathrm{S}_{\mathrm{2}} =\mathrm{4}\left(\frac{\mathrm{49}\pi}{\mathrm{3}}−\frac{\mathrm{49}\sqrt{\mathrm{3}}}{\mathrm{4}}\right) \\ $$$$\mathrm{Consequently},\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{is}\:\mathrm{shaded}\: \\ $$$$\mathrm{region}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{S}_{\mathrm{squareABCD}} −\left(\mathrm{S}_{\mathrm{1}} +\mathrm{S}_{\mathrm{2}} \right) \\ $$$$=\mathrm{14}^{\mathrm{2}} −\mathrm{8}\left(\mathrm{49}−\frac{\mathrm{49}\pi}{\mathrm{6}}−\frac{\mathrm{49}\sqrt{\mathrm{3}}}{\mathrm{4}}\right)−\mathrm{4}\left(\frac{\mathrm{49}\pi}{\mathrm{3}}−\frac{\mathrm{49}\sqrt{\mathrm{3}}}{\mathrm{4}}\right) \\ $$$$=\mathrm{3}.\mathrm{49}\sqrt{\mathrm{3}}−\mathrm{196}\approx\mathrm{58}.\mathrm{61} \\ $$

Commented by 1549442205PVT last updated on 12/Sep/20

Using Geogebra software.Drawing in tinkutara also did this thing

$$\mathrm{Using}\:\mathrm{Geogebra}\:\mathrm{software}.\mathrm{Drawing} \\ $$$$\mathrm{in}\:\mathrm{tinkutara}\:\mathrm{also}\:\mathrm{did}\:\:\mathrm{this}\:\mathrm{thing} \\ $$

Commented by Aina Samuel Temidayo last updated on 12/Sep/20

$$\mathrm{Ok}.\:\mathrm{Thanks}. \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply