Question Number 48611 by Tawa1 last updated on 25/Nov/18

Answered by mr W last updated on 26/Nov/18
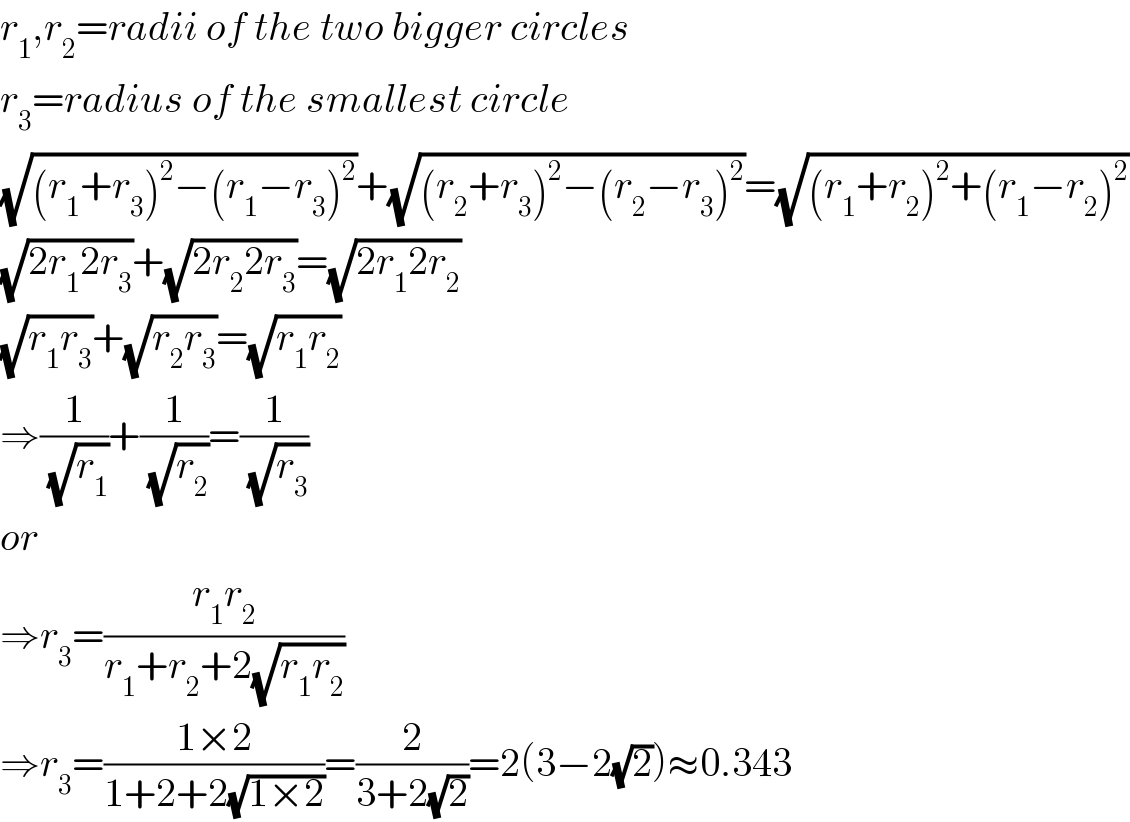
$${r}_{\mathrm{1}} ,{r}_{\mathrm{2}} ={radii}\:{of}\:{the}\:{two}\:{bigger}\:{circles} \\ $$$${r}_{\mathrm{3}} ={radius}\:{of}\:{the}\:{smallest}\:{circle} \\ $$$$\sqrt{\left({r}_{\mathrm{1}} +{r}_{\mathrm{3}} \right)^{\mathrm{2}} −\left({r}_{\mathrm{1}} −{r}_{\mathrm{3}} \right)^{\mathrm{2}} }+\sqrt{\left({r}_{\mathrm{2}} +{r}_{\mathrm{3}} \right)^{\mathrm{2}} −\left({r}_{\mathrm{2}} −{r}_{\mathrm{3}} \right)^{\mathrm{2}} }=\sqrt{\left({r}_{\mathrm{1}} +{r}_{\mathrm{2}} \right)^{\mathrm{2}} +\left({r}_{\mathrm{1}} −{r}_{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$$$\sqrt{\mathrm{2}{r}_{\mathrm{1}} \mathrm{2}{r}_{\mathrm{3}} }+\sqrt{\mathrm{2}{r}_{\mathrm{2}} \mathrm{2}{r}_{\mathrm{3}} }=\sqrt{\mathrm{2}{r}_{\mathrm{1}} \mathrm{2}{r}_{\mathrm{2}} } \\ $$$$\sqrt{{r}_{\mathrm{1}} {r}_{\mathrm{3}} }+\sqrt{{r}_{\mathrm{2}} {r}_{\mathrm{3}} }=\sqrt{{r}_{\mathrm{1}} {r}_{\mathrm{2}} } \\ $$$$\Rightarrow\frac{\mathrm{1}}{\:\sqrt{{r}_{\mathrm{1}} }}+\frac{\mathrm{1}}{\:\sqrt{{r}_{\mathrm{2}} }}=\frac{\mathrm{1}}{\:\sqrt{{r}_{\mathrm{3}} }} \\ $$$${or} \\ $$$$\Rightarrow{r}_{\mathrm{3}} =\frac{{r}_{\mathrm{1}} {r}_{\mathrm{2}} }{{r}_{\mathrm{1}} +{r}_{\mathrm{2}} +\mathrm{2}\sqrt{{r}_{\mathrm{1}} {r}_{\mathrm{2}} }} \\ $$$$\Rightarrow{r}_{\mathrm{3}} =\frac{\mathrm{1}×\mathrm{2}}{\mathrm{1}+\mathrm{2}+\mathrm{2}\sqrt{\mathrm{1}×\mathrm{2}}}=\frac{\mathrm{2}}{\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}}=\mathrm{2}\left(\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\right)\approx\mathrm{0}.\mathrm{343} \\ $$
Commented by Tawa1 last updated on 26/Nov/18
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by Tawa1 last updated on 26/Nov/18
$$\mathrm{Sir},\:\mathrm{does}\:\mathrm{this}\:\mathrm{work}\:\mathrm{for}\:\mathrm{every}\:\mathrm{circle}\:\mathrm{like}\:\mathrm{this}\:?\: \\ $$
Commented by Tawa1 last updated on 26/Nov/18

$$\mathrm{And}\:\mathrm{please}\:\mathrm{sir},\:\mathrm{reference}\:\mathrm{prove}\:\mathrm{of}\:\mathrm{similar}\:\mathrm{circle}\:\mathrm{you}\:\mathrm{have}\:\mathrm{prove} \\ $$$$\mathrm{here}\:\mathrm{before}.\:\:\mathrm{I}\:\mathrm{want}\:\mathrm{to}\:\mathrm{understand}\:\mathrm{the}\:\mathrm{concept}\:\mathrm{of}\:\mathrm{the}\:\mathrm{question} \\ $$$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by mr W last updated on 26/Nov/18

Commented by mr W last updated on 26/Nov/18
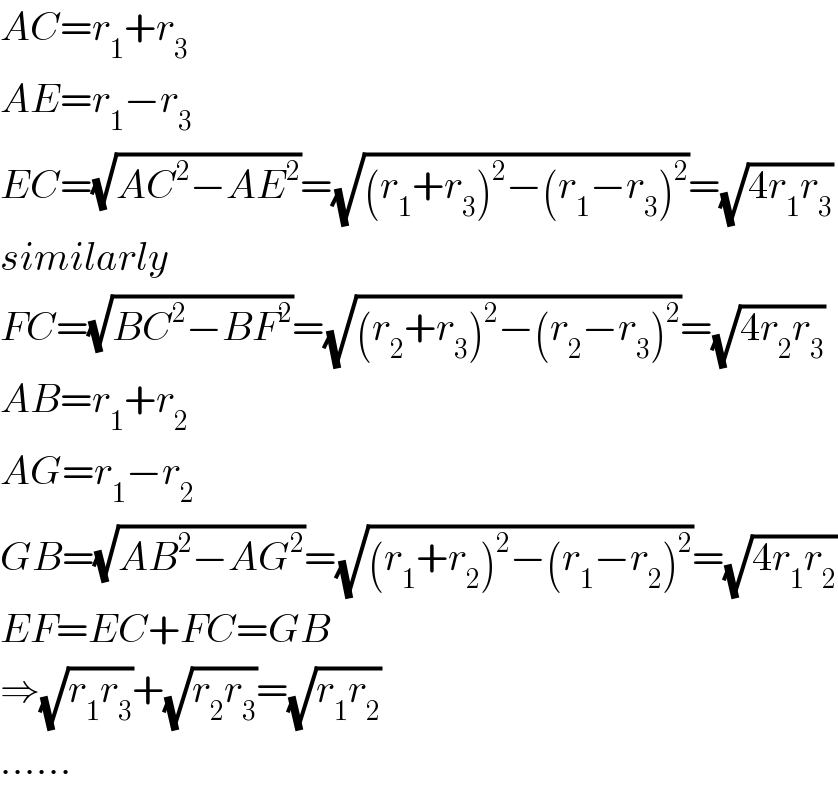
$${AC}={r}_{\mathrm{1}} +{r}_{\mathrm{3}} \\ $$$${AE}={r}_{\mathrm{1}} −{r}_{\mathrm{3}} \\ $$$${EC}=\sqrt{{AC}^{\mathrm{2}} −{AE}^{\mathrm{2}} }=\sqrt{\left({r}_{\mathrm{1}} +{r}_{\mathrm{3}} \right)^{\mathrm{2}} −\left({r}_{\mathrm{1}} −{r}_{\mathrm{3}} \right)^{\mathrm{2}} }=\sqrt{\mathrm{4}{r}_{\mathrm{1}} {r}_{\mathrm{3}} } \\ $$$${similarly} \\ $$$${FC}=\sqrt{{BC}^{\mathrm{2}} −{BF}^{\mathrm{2}} }=\sqrt{\left({r}_{\mathrm{2}} +{r}_{\mathrm{3}} \right)^{\mathrm{2}} −\left({r}_{\mathrm{2}} −{r}_{\mathrm{3}} \right)^{\mathrm{2}} }=\sqrt{\mathrm{4}{r}_{\mathrm{2}} {r}_{\mathrm{3}} } \\ $$$${AB}={r}_{\mathrm{1}} +{r}_{\mathrm{2}} \\ $$$${AG}={r}_{\mathrm{1}} −{r}_{\mathrm{2}} \\ $$$${GB}=\sqrt{{AB}^{\mathrm{2}} −{AG}^{\mathrm{2}} }=\sqrt{\left({r}_{\mathrm{1}} +{r}_{\mathrm{2}} \right)^{\mathrm{2}} −\left({r}_{\mathrm{1}} −{r}_{\mathrm{2}} \right)^{\mathrm{2}} }=\sqrt{\mathrm{4}{r}_{\mathrm{1}} {r}_{\mathrm{2}} } \\ $$$${EF}={EC}+{FC}={GB} \\ $$$$\Rightarrow\sqrt{{r}_{\mathrm{1}} {r}_{\mathrm{3}} }+\sqrt{{r}_{\mathrm{2}} {r}_{\mathrm{3}} }=\sqrt{{r}_{\mathrm{1}} {r}_{\mathrm{2}} } \\ $$$$…… \\ $$
Commented by Tawa1 last updated on 26/Nov/18
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{appreciate}\:\mathrm{your}\:\mathrm{time} \\ $$
