Question Number 135952 by Dwaipayan Shikari last updated on 17/Mar/21
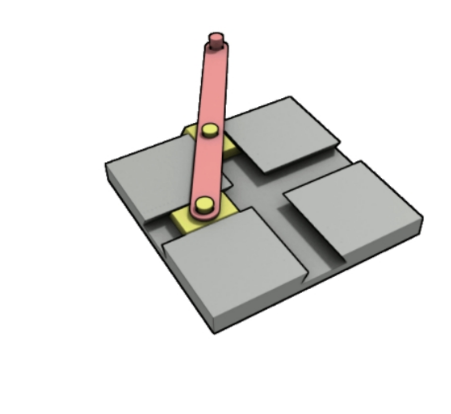
Commented by Dwaipayan Shikari last updated on 17/Mar/21

$${It}\:{isArchimedes}'{s}\:{Nothing}\:{Grinder}.\:{We}\:{can}\:{move}\:{this}\:{back} \\ $$$${and}\:{forth}\:{and}\:{sideways}\:.{Prove}\:{that}\:{the}\:{tip}\:{on}\:{various} \\ $$$${positions}\:{on}\:{that}\:{hand}\:{of}\:{the}\:{wodden}\:{grinder}\:{creates}\: \\ $$$${various}\:{shapes}\:{of}\:{ellipses} \\ $$
Commented by Dwaipayan Shikari last updated on 17/Mar/21
Take a look at this animation https://en.wikipedia.org/wiki/File:Trammel_of_Archimedes_Large.gif
Answered by mr W last updated on 17/Mar/21
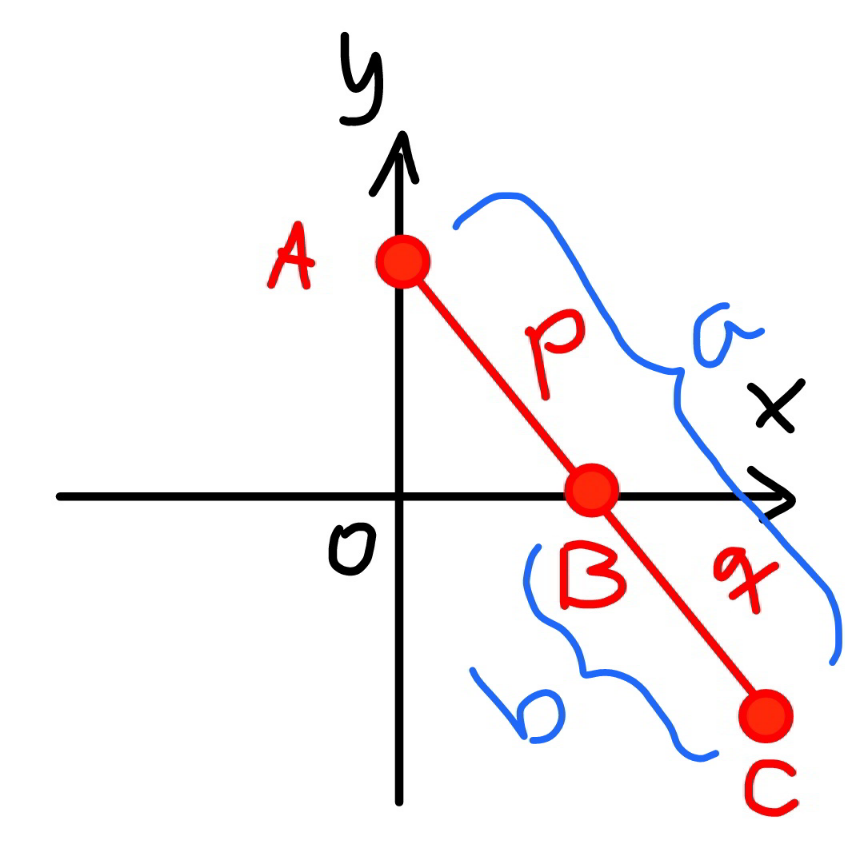
Commented by mr W last updated on 17/Mar/21

$${say} \\ $$$${B}\left({t},\mathrm{0}\right) \\ $$$${A}\left(\mathrm{0},{s}\right) \\ $$$${s}^{\mathrm{2}} +{t}^{\mathrm{2}} ={p}^{\mathrm{2}} \\ $$$${x}_{{C}} =\frac{{q}+{p}}{{p}}{t}\:\Rightarrow{t}=\frac{{p}}{{p}+{q}}{x}_{{C}} \\ $$$${y}_{{C}} =−\frac{{q}}{{p}}{s}\:\Rightarrow{s}=−\frac{{p}}{{q}}{y}_{{C}} \\ $$$$\left(\frac{{p}}{{p}+{q}}{x}_{{C}} \right)^{\mathrm{2}} +\left(−\frac{{p}}{{q}}{y}_{{C}} \right)^{\mathrm{2}} ={p}^{\mathrm{2}} \\ $$$$\frac{{x}_{{C}} ^{\mathrm{2}} }{\left({p}+{q}\right)^{\mathrm{2}} }+\frac{{y}_{{C}} ^{\mathrm{2}} }{{q}^{\mathrm{2}} }=\mathrm{1} \\ $$$${or} \\ $$$$\frac{{x}^{\mathrm{2}} }{\left({p}+{q}\right)^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{q}^{\mathrm{2}} }=\mathrm{1} \\ $$$${i}.{e}.\:{the}\:{locus}\:{of}\:{C}\:{is}\:{an}\:{ellipse}\:{with} \\ $$$${semiaxses}: \\ $$$${a}={p}+{q} \\ $$$${b}={q} \\ $$
Commented by Dwaipayan Shikari last updated on 17/Mar/21

$${Thanks}\:{sir}!\:{i}\:{didn}'{t}\:{think}\:{that}\:{the}\:{proof}\:{is}\:{simple} \\ $$
