Menu Close

C-e-z-1-cos-z-dz-C-z-1-

Tinku Tara 0 Comments
Pin




Question Number 115009 by arcana last updated on 22/Sep/20
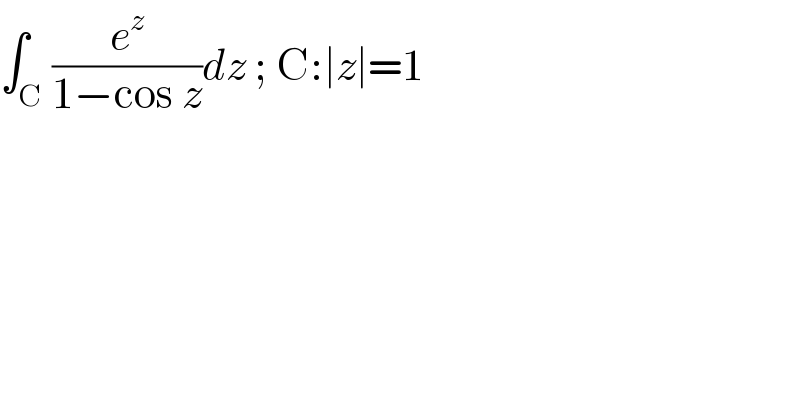
∫_C (e^z /(1−cos z))dz ; C:∣z∣=1
$$\int_{\mathrm{C}} \frac{{e}^{{z}} }{\mathrm{1}−\mathrm{cos}\:{z}}{dz}\:;\:\mathrm{C}:\mid{z}\mid=\mathrm{1} \\ $$
Answered by Olaf last updated on 24/Sep/20
∫_C ((coz+isinz)/(1−cosz))dz ∫_C ((1+isinz+(cosz−1))/(1−cosz))dz ∫_C (dz/(1−cosz))+i∫_C ((sinz)/(1−cosz))−∫_C dz ∫_C ((2dz)/(sin^2 (z/2)))+i∫_C ((sinz)/(1−cosz))−∫_C dz [−4cot(z/2)+iln(1−cosz)−z]_C Let z = e^(iθ) , θ∈[0 ; 2π] [−4cot(e^(iθ) /2)+iln(1−cose^(iθ) )−e^(iθ) ]_0 ^(2π) = 0
$$ \\ $$$$\int_{\mathrm{C}} \frac{\mathrm{co}{z}+{i}\mathrm{sin}{z}}{\mathrm{1}−\mathrm{cos}{z}}{dz} \\ $$$$\int_{\mathrm{C}} \frac{\mathrm{1}+{i}\mathrm{sin}{z}+\left(\mathrm{cos}{z}−\mathrm{1}\right)}{\mathrm{1}−\mathrm{cos}{z}}{dz} \\ $$$$\int_{\mathrm{C}} \frac{{dz}}{\mathrm{1}−\mathrm{cos}{z}}+{i}\int_{\mathrm{C}} \frac{\mathrm{sin}{z}}{\mathrm{1}−\mathrm{cos}{z}}−\int_{\mathrm{C}} {dz} \\ $$$$\int_{\mathrm{C}} \frac{\mathrm{2}{dz}}{\mathrm{sin}^{\mathrm{2}} \frac{{z}}{\mathrm{2}}}+{i}\int_{\mathrm{C}} \frac{\mathrm{sin}{z}}{\mathrm{1}−\mathrm{cos}{z}}−\int_{\mathrm{C}} {dz} \\ $$$$\left[−\mathrm{4cot}\frac{{z}}{\mathrm{2}}+{i}\mathrm{ln}\left(\mathrm{1}−\mathrm{cos}{z}\right)−{z}\right]_{\mathrm{C}} \\ $$$$\mathrm{Let}\:{z}\:=\:{e}^{{i}\theta} ,\:\theta\in\left[\mathrm{0}\:;\:\mathrm{2}\pi\right] \\ $$$$\left[−\mathrm{4cot}\frac{{e}^{{i}\theta} }{\mathrm{2}}+{i}\mathrm{ln}\left(\mathrm{1}−\mathrm{cos}{e}^{{i}\theta} \right)−{e}^{{i}\theta} \right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$=\:\mathrm{0} \\ $$
Answered by Bird last updated on 24/Sep/20
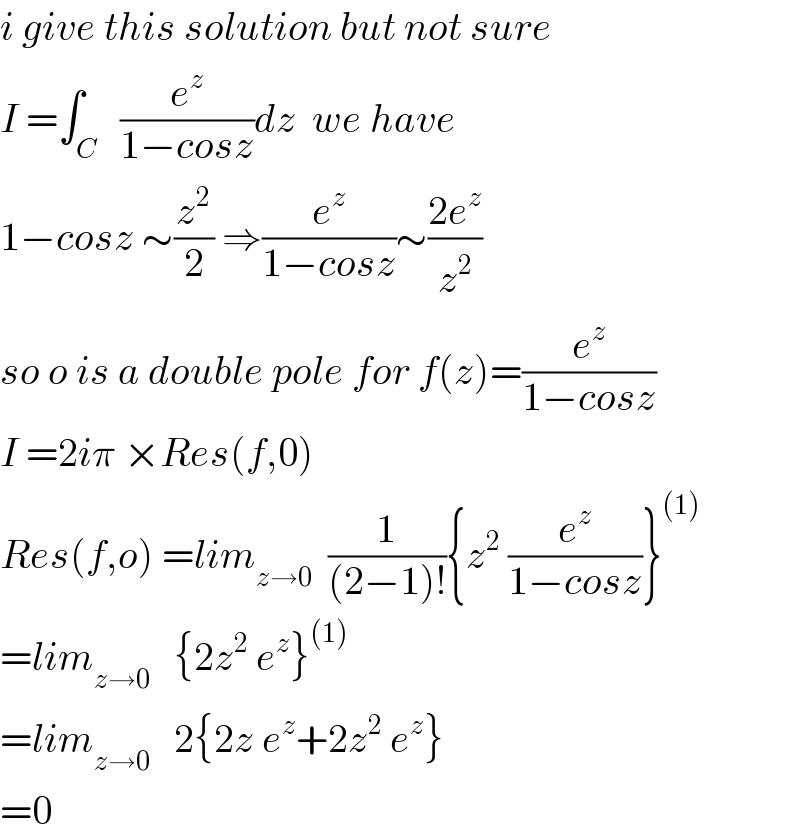
i give this solution but not sure I =∫_C (e^z /(1−cosz))dz we have 1−cosz ∼(z^2 /2) ⇒(e^z /(1−cosz))∼((2e^z )/z^2 ) so o is a double pole for f(z)=(e^z /(1−cosz)) I =2iπ ×Res(f,0) Res(f,o) =lim_(z→0) (1/((2−1)!)){z^2 (e^z /(1−cosz))}^((1)) =lim_(z→0) {2z^2 e^z }^((1)) =lim_(z→0) 2{2z e^z +2z^2 e^z } =0
$${i}\:{give}\:{this}\:{solution}\:{but}\:{not}\:{sure} \\ $$$${I}\:=\int_{{C}} \:\:\frac{{e}^{{z}} }{\mathrm{1}−{cosz}}{dz}\:\:{we}\:{have} \\ $$$$\mathrm{1}−{cosz}\:\sim\frac{{z}^{\mathrm{2}} }{\mathrm{2}}\:\Rightarrow\frac{{e}^{{z}} }{\mathrm{1}−{cosz}}\sim\frac{\mathrm{2}{e}^{{z}} }{{z}^{\mathrm{2}} } \\ $$$${so}\:{o}\:{is}\:{a}\:{double}\:{pole}\:{for}\:{f}\left({z}\right)=\frac{{e}^{{z}} }{\mathrm{1}−{cosz}} \\ $$$${I}\:=\mathrm{2}{i}\pi\:×{Res}\left({f},\mathrm{0}\right) \\ $$$${Res}\left({f},{o}\right)\:={lim}_{{z}\rightarrow\mathrm{0}} \:\:\frac{\mathrm{1}}{\left(\mathrm{2}−\mathrm{1}\right)!}\left\{{z}^{\mathrm{2}} \:\frac{{e}^{{z}} }{\mathrm{1}−{cosz}}\right\}^{\left(\mathrm{1}\right)} \\ $$$$={lim}_{{z}\rightarrow\mathrm{0}} \:\:\:\left\{\mathrm{2}{z}^{\mathrm{2}} \:{e}^{{z}} \right\}^{\left(\mathrm{1}\right)} \\ $$$$={lim}_{{z}\rightarrow\mathrm{0}} \:\:\:\mathrm{2}\left\{\mathrm{2}{z}\:{e}^{{z}} +\mathrm{2}{z}^{\mathrm{2}} \:{e}^{{z}} \right\} \\ $$$$=\mathrm{0} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *