Question Number 4911 by FilupSmith last updated on 21/Mar/16
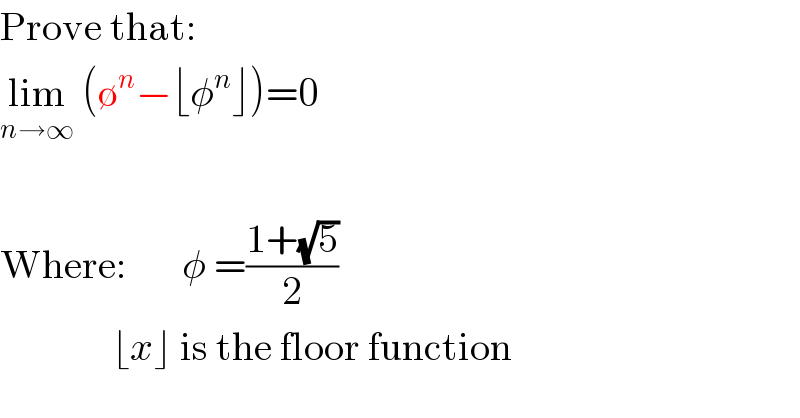
$$\mathrm{Prove}\:\mathrm{that}: \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\:\left(\emptyset^{{n}} −\lfloor\phi^{{n}} \rfloor\right)=\mathrm{0} \\ $$$$ \\ $$$$\mathrm{Where}:\:\:\:\:\:\:\:\phi\:=\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\lfloor{x}\rfloor\:\mathrm{is}\:\mathrm{the}\:\mathrm{floor}\:\mathrm{function} \\ $$
Commented by Yozzii last updated on 21/Mar/16

$${Let}\:{f}\left({x}\right)={a}^{{x}} \:{where}\:{a}>\mathrm{1},{x}\in\mathbb{R}^{+} . \\ $$$$\Rightarrow{f}^{'} \left({x}\right)={a}^{{x}} {lna}. \\ $$$${a}>\mathrm{1}\Rightarrow{lna}>\mathrm{0}\:{and}\:{a}>\mathrm{1}\Rightarrow{a}^{{x}} >\mathrm{1}>\mathrm{0}\:{for}\:{x}\in\mathbb{R}^{+} . \\ $$$${So},\:{f}^{'} \left({x}\right)>\mathrm{0}\:{for}\:{all}\:{x}\in\mathbb{R}^{+} .\:{Hence}, \\ $$$${f}\:{is}\:{perpetually}\:{increasing}\:{as}\:{x}\:{increases}. \\ $$$${Treating}\:{x}\:{as}\:{an}\:{natural}\:{number}\:{n}, \\ $$$${f}\left({n}\right)={a}^{{n}} \:{is}\:{increasing}\:{for}\:{all}\:{n}\in\mathbb{N}. \\ $$$${Now},\:\phi\approx\mathrm{1}.\mathrm{618033989}>\mathrm{1}. \\ $$$$\Rightarrow\phi^{{n}} >\mathrm{1}\Rightarrow{f}\left({n}\right)>\mathrm{1}.\Rightarrow\lfloor{f}\left({n}\right)\rfloor\geqslant\mathrm{1}>\mathrm{0}\:{for}\: \\ $$$${all}\:{n}\in\mathbb{N}.\:{So},\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}\lfloor{f}\left({n}\right)\rfloor\neq\mathrm{0}.\: \\ $$$$ \\ $$$$ \\ $$
Commented by FilupSmith last updated on 21/Mar/16

$${sorry}\:{i}\:{made}\:{a}\:{horrible}\:{typo}\:{on}\:{my}\:{question} \\ $$
Answered by Algebro last updated on 21/Mar/16
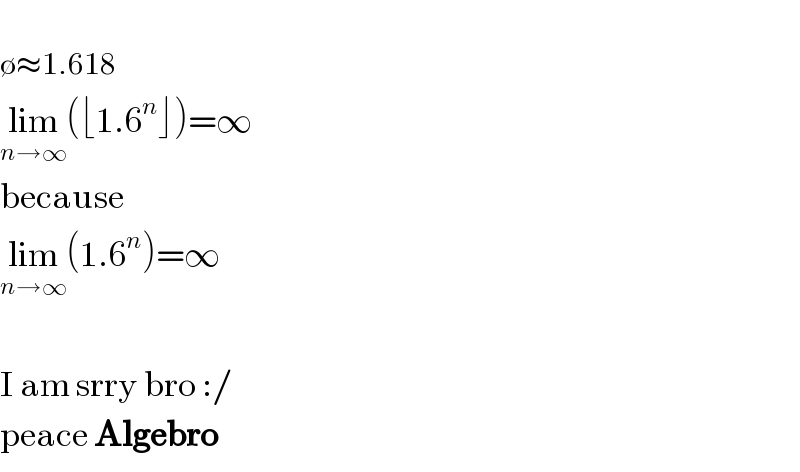
$$ \\ $$$$\emptyset\approx\mathrm{1}.\mathrm{618} \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\lfloor\mathrm{1}.\mathrm{6}^{{n}} \rfloor\right)=\infty \\ $$$$\mathrm{because} \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\mathrm{1}.\mathrm{6}^{{n}} \right)=\infty \\ $$$$ \\ $$$$\mathrm{I}\:\mathrm{am}\:\mathrm{srry}\:\mathrm{bro}\::/ \\ $$$$\mathrm{peace}\:\boldsymbol{\mathrm{Algebro}} \\ $$
