Menu Close

proof-that-for-a-function-f-continuos-on-0-and-integrable-if-0-f-x-dx-converge-then-0-f-x-dx-converge-

Tinku Tara
Pin




Question Number 404 by 123456 last updated on 30/Dec/14
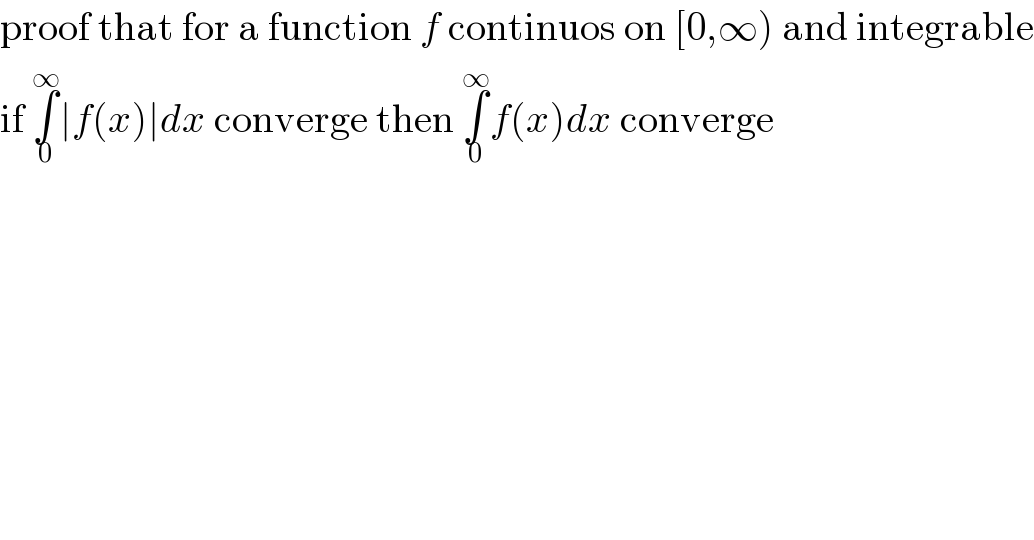
proof that for a function f continuos on [0,∞) and integrable if ∫_0 ^∞ ∣f(x)∣dx converge then ∫_0 ^∞ f(x)dx converge
$$\mathrm{proof}\:\mathrm{that}\:\mathrm{for}\:\mathrm{a}\:\mathrm{function}\:{f}\:\mathrm{continuos}\:\mathrm{on}\:\left[\mathrm{0},\infty\right)\:\mathrm{and}\:\mathrm{integrable} \\ $$$$\mathrm{if}\:\underset{\mathrm{0}} {\overset{\infty} {\int}}\mid{f}\left({x}\right)\mid{dx}\:\mathrm{converge}\:\mathrm{then}\:\underset{\mathrm{0}} {\overset{\infty} {\int}}{f}\left({x}\right){dx}\:\mathrm{converge} \\ $$

Pin