0-pi-ln-1-1-2-cos-x-cos-x-dx-

Question Number 116216 by bemath last updated on 02/Oct/20

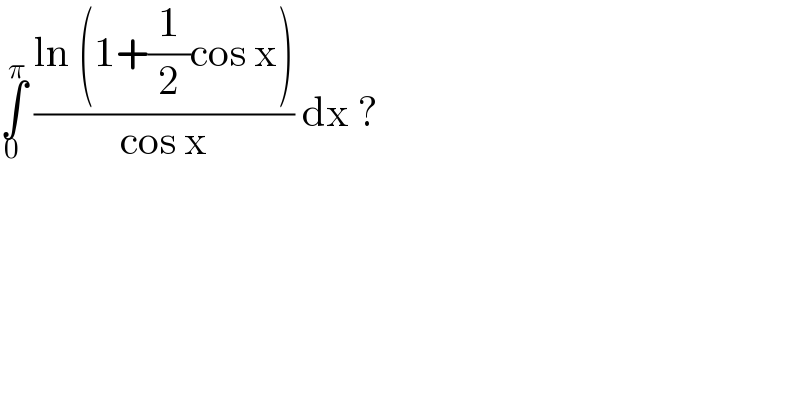
∫_0 ^π ((ln (1+(1/2)cos x))/(cos x)) dx ?

$$\underset{\mathrm{0}} {\overset{\pi} {\int}}\:\frac{\mathrm{ln}\:\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\mathrm{x}\right)}{\mathrm{cos}\:\mathrm{x}}\:\mathrm{dx}\:? \\ $$

Answered by Bird last updated on 02/Oct/20

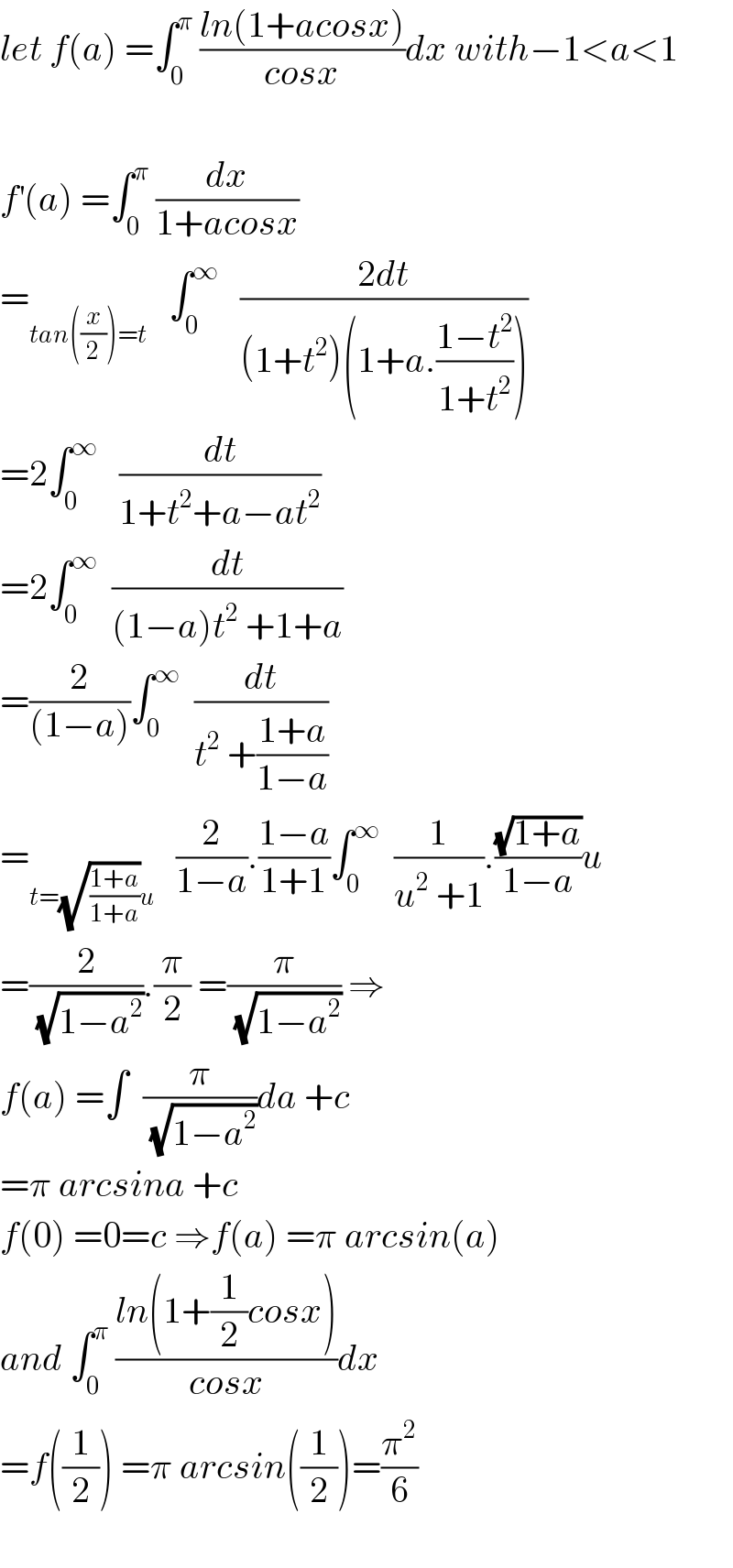
let f(a) =∫_0 ^π ((ln(1+acosx))/(cosx))dx with−1<a<1 f^′ (a) =∫_0 ^π (dx/(1+acosx)) =_(tan((x/2))=t) ∫_0 ^∞ ((2dt)/((1+t^2 )(1+a.((1−t^2 )/(1+t^2 ))))) =2∫_0 ^∞ (dt/(1+t^2 +a−at^2 )) =2∫_0 ^∞ (dt/((1−a)t^2 +1+a)) =(2/((1−a)))∫_0 ^∞ (dt/(t^2 +((1+a)/(1−a)))) =_(t=(√((1+a)/(1+a)))u) (2/(1−a)).((1−a)/(1+1))∫_0 ^∞ (1/(u^2 +1)).((√(1+a))/(1−a))u =(2/( (√(1−a^2 )))).(π/2) =(π/( (√(1−a^2 )))) ⇒ f(a) =∫ (π/( (√(1−a^2 ))))da +c =π arcsina +c f(0) =0=c ⇒f(a) =π arcsin(a) and ∫_0 ^π ((ln(1+(1/2)cosx))/(cosx))dx =f((1/2)) =π arcsin((1/2))=(π^2 /6)

$${let}\:{f}\left({a}\right)\:=\int_{\mathrm{0}} ^{\pi} \:\frac{{ln}\left(\mathrm{1}+{acosx}\right)}{{cosx}}{dx}\:{with}−\mathrm{1}<{a}<\mathrm{1} \\ $$$$ \\ $$$${f}^{'} \left({a}\right)\:=\int_{\mathrm{0}} ^{\pi} \:\frac{{dx}}{\mathrm{1}+{acosx}} \\ $$$$=_{{tan}\left(\frac{{x}}{\mathrm{2}}\right)={t}} \:\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{2}{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\left(\mathrm{1}+{a}.\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }\right)} \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} +{a}−{at}^{\mathrm{2}} } \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dt}}{\left(\mathrm{1}−{a}\right){t}^{\mathrm{2}} \:+\mathrm{1}+{a}} \\ $$$$=\frac{\mathrm{2}}{\left(\mathrm{1}−{a}\right)}\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\frac{\mathrm{1}+{a}}{\mathrm{1}−{a}}} \\ $$$$=_{{t}=\sqrt{\frac{\mathrm{1}+{a}}{\mathrm{1}+{a}}}{u}} \:\:\:\frac{\mathrm{2}}{\mathrm{1}−{a}}.\frac{\mathrm{1}−{a}}{\mathrm{1}+\mathrm{1}}\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{1}}{{u}^{\mathrm{2}} \:+\mathrm{1}}.\frac{\sqrt{\mathrm{1}+{a}}}{\mathrm{1}−{a}}{u} \\ $$$$=\frac{\mathrm{2}}{\:\sqrt{\mathrm{1}−{a}^{\mathrm{2}} }}.\frac{\pi}{\mathrm{2}}\:=\frac{\pi}{\:\sqrt{\mathrm{1}−{a}^{\mathrm{2}} }}\:\Rightarrow \\ $$$${f}\left({a}\right)\:=\int\:\:\frac{\pi}{\:\sqrt{\mathrm{1}−{a}^{\mathrm{2}} }}{da}\:+{c} \\ $$$$=\pi\:{arcsina}\:+{c} \\ $$$${f}\left(\mathrm{0}\right)\:=\mathrm{0}={c}\:\Rightarrow{f}\left({a}\right)\:=\pi\:{arcsin}\left({a}\right) \\ $$$${and}\:\int_{\mathrm{0}} ^{\pi} \:\frac{{ln}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}{cosx}\right)}{{cosx}}{dx} \\ $$$$={f}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\:=\pi\:{arcsin}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)=\frac{\pi^{\mathrm{2}} }{\mathrm{6}} \\ $$$$ \\ $$

Commented by bemath last updated on 02/Oct/20