Menu Close

0-1-x-1-x-1-lnx-dx-

Tinku Tara 0 Comments
Pin




Question Number 182529 by sciencestudent last updated on 10/Dec/22
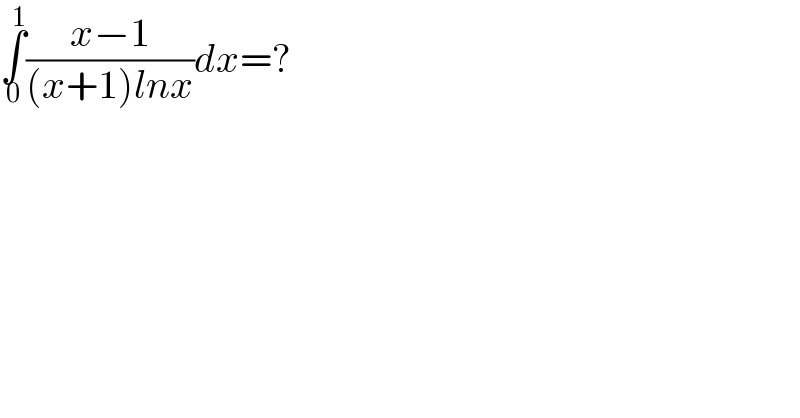
∫_0 ^1 ((x−1)/((x+1)lnx))dx=?
$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{{x}−\mathrm{1}}{\left({x}+\mathrm{1}\right){lnx}}{dx}=? \\ $$
Answered by Ar Brandon last updated on 10/Dec/22
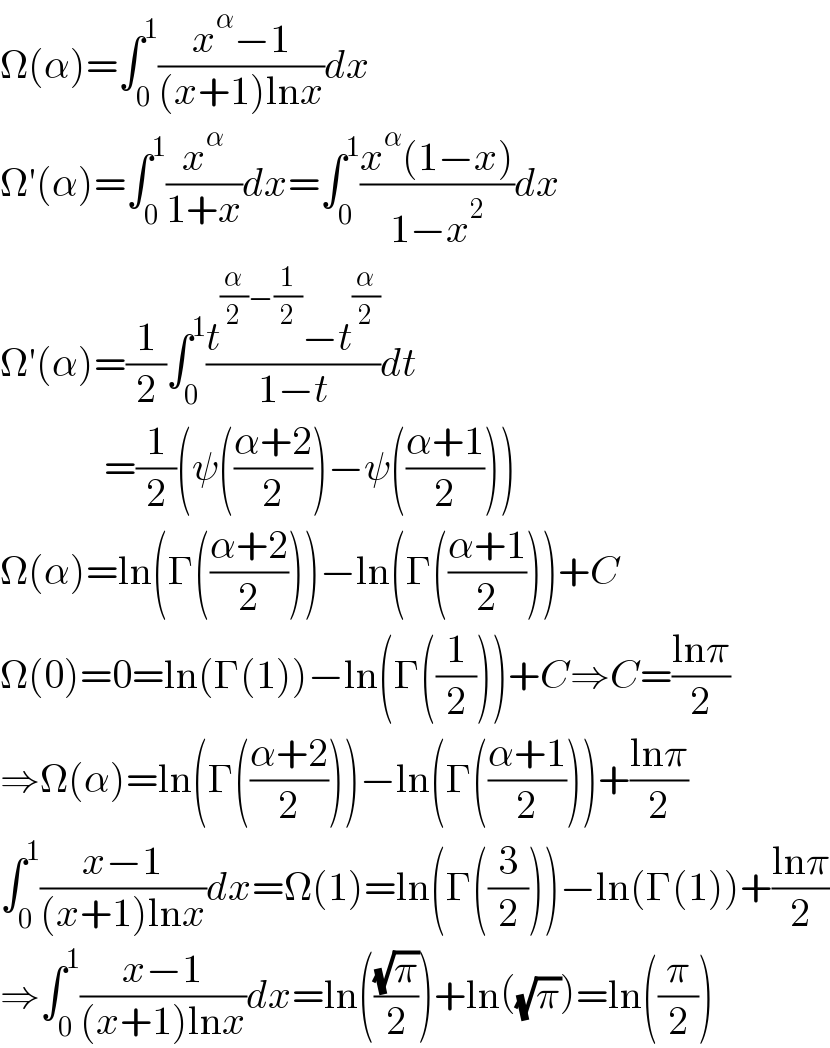
Ω(α)=∫_0 ^1 ((x^α −1)/((x+1)lnx))dx Ω′(α)=∫_0 ^1 (x^α /(1+x))dx=∫_0 ^1 ((x^α (1−x))/(1−x^2 ))dx Ω′(α)=(1/2)∫_0 ^1 ((t^((α/2)−(1/2)) −t^(α/2) )/(1−t))dt =(1/2)(ψ(((α+2)/2))−ψ(((α+1)/2))) Ω(α)=ln(Γ(((α+2)/2)))−ln(Γ(((α+1)/2)))+C Ω(0)=0=ln(Γ(1))−ln(Γ((1/2)))+C⇒C=((lnπ)/2) ⇒Ω(α)=ln(Γ(((α+2)/2)))−ln(Γ(((α+1)/2)))+((lnπ)/2) ∫_0 ^1 ((x−1)/((x+1)lnx))dx=Ω(1)=ln(Γ((3/2)))−ln(Γ(1))+((lnπ)/2) ⇒∫_0 ^1 ((x−1)/((x+1)lnx))dx=ln(((√π)/2))+ln((√π))=ln((π/2))
$$\Omega\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\alpha} −\mathrm{1}}{\left({x}+\mathrm{1}\right)\mathrm{ln}{x}}{dx} \\ $$$$\Omega'\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\alpha} }{\mathrm{1}+{x}}{dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\alpha} \left(\mathrm{1}−{x}\right)}{\mathrm{1}−{x}^{\mathrm{2}} }{dx} \\ $$$$\Omega'\left(\alpha\right)=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{t}^{\frac{\alpha}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}} −{t}^{\frac{\alpha}{\mathrm{2}}} }{\mathrm{1}−{t}}{dt} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\left(\psi\left(\frac{\alpha+\mathrm{2}}{\mathrm{2}}\right)−\psi\left(\frac{\alpha+\mathrm{1}}{\mathrm{2}}\right)\right) \\ $$$$\Omega\left(\alpha\right)=\mathrm{ln}\left(\Gamma\left(\frac{\alpha+\mathrm{2}}{\mathrm{2}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\alpha+\mathrm{1}}{\mathrm{2}}\right)\right)+{C} \\ $$$$\Omega\left(\mathrm{0}\right)=\mathrm{0}=\mathrm{ln}\left(\Gamma\left(\mathrm{1}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)+{C}\Rightarrow{C}=\frac{\mathrm{ln}\pi}{\mathrm{2}} \\ $$$$\Rightarrow\Omega\left(\alpha\right)=\mathrm{ln}\left(\Gamma\left(\frac{\alpha+\mathrm{2}}{\mathrm{2}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\alpha+\mathrm{1}}{\mathrm{2}}\right)\right)+\frac{\mathrm{ln}\pi}{\mathrm{2}} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}−\mathrm{1}}{\left({x}+\mathrm{1}\right)\mathrm{ln}{x}}{dx}=\Omega\left(\mathrm{1}\right)=\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{3}}{\mathrm{2}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\mathrm{1}\right)\right)+\frac{\mathrm{ln}\pi}{\mathrm{2}} \\ $$$$\Rightarrow\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}−\mathrm{1}}{\left({x}+\mathrm{1}\right)\mathrm{ln}{x}}{dx}=\mathrm{ln}\left(\frac{\sqrt{\pi}}{\mathrm{2}}\right)+\mathrm{ln}\left(\sqrt{\pi}\right)=\mathrm{ln}\left(\frac{\pi}{\mathrm{2}}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *