Question Number 117052 by bemath last updated on 09/Oct/20
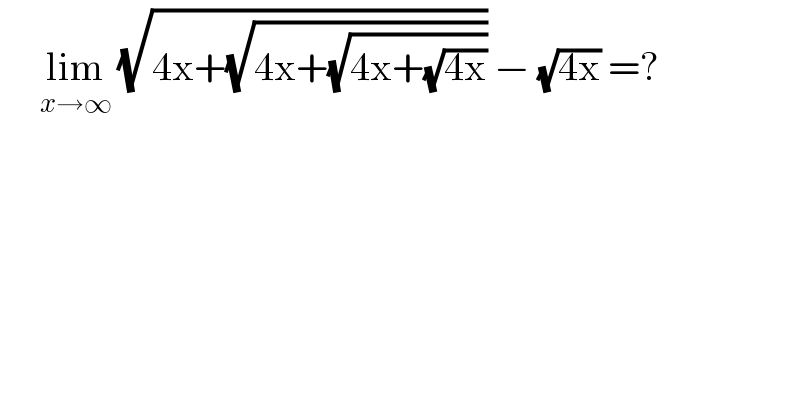
$$\:\:\:\:\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\sqrt{\mathrm{4x}+\sqrt{\mathrm{4x}+\sqrt{\mathrm{4x}+\sqrt{\mathrm{4x}}}}}\:−\:\sqrt{\mathrm{4x}}\:=? \\ $$
Answered by bobhans last updated on 09/Oct/20
![lim_(x→∞) (√(4x(1+((√(4x+(√(4x+(√(4x))))))/(4x))))) −(√(4x)) lim_(x→∞) (√(4x)) [ (√(1+(√((1/(4x))+(√((1/((4x)^3 ))+(√(1/((4x)^7 ))))))))) −1 ] = letting (√(4x)) = (1/t)→ { (((1/(4x))=t^2 )),(((1/((4x)^3 )) = t^6 )),(((1/((4x)^7 )) = t^(14) )) :} lim_(t→0) (((√(1+(√(t^2 +(√(t^6 +(√t^(14) ))))))) −1)/t) = (1/2) × lim_(t→0) ((√(t^2 +(√(t^6 +t^7 ))))/t) = (1/2)×lim_(t→0) ((√(t^2 +t^3 (√(1+t))))/t) = (1/2) × lim_(t→0) ((t(√(1+t(√(1+t)))))/t) = (1/2)](https://www.tinkutara.com/question/Q117057.png)
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\sqrt{\mathrm{4x}\left(\mathrm{1}+\frac{\sqrt{\mathrm{4x}+\sqrt{\mathrm{4x}+\sqrt{\mathrm{4x}}}}}{\mathrm{4x}}\right)}\:−\sqrt{\mathrm{4x}} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\sqrt{\mathrm{4x}}\:\left[\:\sqrt{\mathrm{1}+\sqrt{\frac{\mathrm{1}}{\mathrm{4x}}+\sqrt{\frac{\mathrm{1}}{\left(\mathrm{4x}\right)^{\mathrm{3}} }+\sqrt{\frac{\mathrm{1}}{\left(\mathrm{4x}\right)^{\mathrm{7}} }}}}}\:−\mathrm{1}\:\right]\:= \\ $$$$\mathrm{letting}\:\sqrt{\mathrm{4x}}\:=\:\frac{\mathrm{1}}{\mathrm{t}}\rightarrow\begin{cases}{\frac{\mathrm{1}}{\mathrm{4x}}=\mathrm{t}^{\mathrm{2}} }\\{\frac{\mathrm{1}}{\left(\mathrm{4x}\right)^{\mathrm{3}} }\:=\:\mathrm{t}^{\mathrm{6}} \:}\\{\frac{\mathrm{1}}{\left(\mathrm{4x}\right)^{\mathrm{7}} }\:=\:\mathrm{t}^{\mathrm{14}} }\end{cases} \\ $$$$\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{1}+\sqrt{\mathrm{t}^{\mathrm{2}} +\sqrt{\mathrm{t}^{\mathrm{6}} +\sqrt{\mathrm{t}^{\mathrm{14}} }}}}\:−\mathrm{1}}{\mathrm{t}}\:=\: \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\:×\:\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{t}^{\mathrm{2}} +\sqrt{\mathrm{t}^{\mathrm{6}} +\mathrm{t}^{\mathrm{7}} }}}{\mathrm{t}}\:=\:\frac{\mathrm{1}}{\mathrm{2}}×\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{t}^{\mathrm{2}} +\mathrm{t}^{\mathrm{3}} \sqrt{\mathrm{1}+\mathrm{t}}}}{\mathrm{t}} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}\:×\:\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{t}\sqrt{\mathrm{1}+\mathrm{t}\sqrt{\mathrm{1}+\mathrm{t}}}}{\mathrm{t}}\:=\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$
