Question-117311

Question Number 117311 by eric last updated on 10/Oct/20

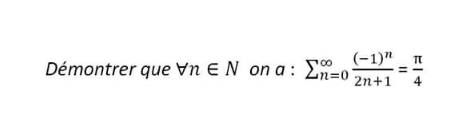
Answered by Dwaipayan Shikari last updated on 10/Oct/20

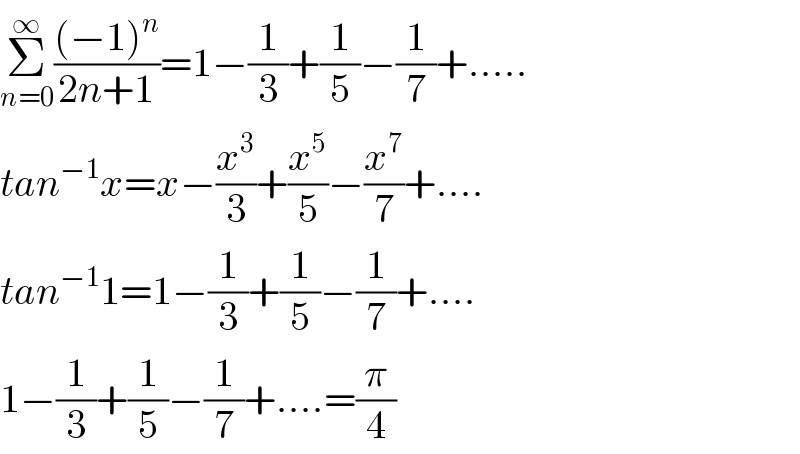
Σ_(n=0) ^∞ (((−1)^n )/(2n+1))=1−(1/3)+(1/5)−(1/7)+..... tan^(−1) x=x−(x^3 /3)+(x^5 /5)−(x^7 /7)+.... tan^(−1) 1=1−(1/3)+(1/5)−(1/7)+.... 1−(1/3)+(1/5)−(1/7)+....=(π/4)

$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{2}{n}+\mathrm{1}}=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{5}}−\frac{\mathrm{1}}{\mathrm{7}}+….. \\ $$$${tan}^{−\mathrm{1}} {x}={x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}+\frac{{x}^{\mathrm{5}} }{\mathrm{5}}−\frac{{x}^{\mathrm{7}} }{\mathrm{7}}+…. \\ $$$${tan}^{−\mathrm{1}} \mathrm{1}=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{5}}−\frac{\mathrm{1}}{\mathrm{7}}+…. \\ $$$$\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{5}}−\frac{\mathrm{1}}{\mathrm{7}}+….=\frac{\pi}{\mathrm{4}} \\ $$

Commented by TANMAY PANACEA last updated on 10/Oct/20

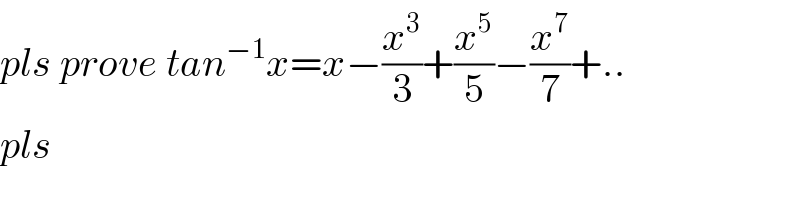
pls prove tan^(−1) x=x−(x^3 /3)+(x^5 /5)−(x^7 /7)+.. pls

$${pls}\:{prove}\:{tan}^{−\mathrm{1}} {x}={x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}+\frac{{x}^{\mathrm{5}} }{\mathrm{5}}−\frac{{x}^{\mathrm{7}} }{\mathrm{7}}+.. \\ $$$${pls} \\ $$

Commented by Dwaipayan Shikari last updated on 10/Oct/20

f(x)=tan^(−1) x f′(x)=(1/(1+x^2 ))=(1+x^2 )^(−1) =1−x^2 +x^4 −x^6 +... f(x)=∫1−x^2 +x^4 −x^6 +.. f(x)=x−(x^3 /3)+(x^5 /5)−(x^7 /7)+....+C f(0)=tan^(−1) (0)=0 C=0 f(x)=tan^(−1) x=x−(x^3 /3)+(x^5 /5)−(x^7 /7)+....

$${f}\left({x}\right)={tan}^{−\mathrm{1}} {x} \\ $$$${f}'\left({x}\right)=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }=\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{−\mathrm{1}} =\mathrm{1}−{x}^{\mathrm{2}} +{x}^{\mathrm{4}} −{x}^{\mathrm{6}} +… \\ $$$${f}\left({x}\right)=\int\mathrm{1}−{x}^{\mathrm{2}} +{x}^{\mathrm{4}} −{x}^{\mathrm{6}} +.. \\ $$$${f}\left({x}\right)={x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}+\frac{{x}^{\mathrm{5}} }{\mathrm{5}}−\frac{{x}^{\mathrm{7}} }{\mathrm{7}}+….+{C} \\ $$$${f}\left(\mathrm{0}\right)={tan}^{−\mathrm{1}} \left(\mathrm{0}\right)=\mathrm{0} \\ $$$${C}=\mathrm{0} \\ $$$${f}\left({x}\right)={tan}^{−\mathrm{1}} {x}={x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}+\frac{{x}^{\mathrm{5}} }{\mathrm{5}}−\frac{{x}^{\mathrm{7}} }{\mathrm{7}}+…. \\ $$$$ \\ $$

Commented by TANMAY PANACEA last updated on 10/Oct/20

$${excellent} \\ $$

Answered by mathmax by abdo last updated on 10/Oct/20

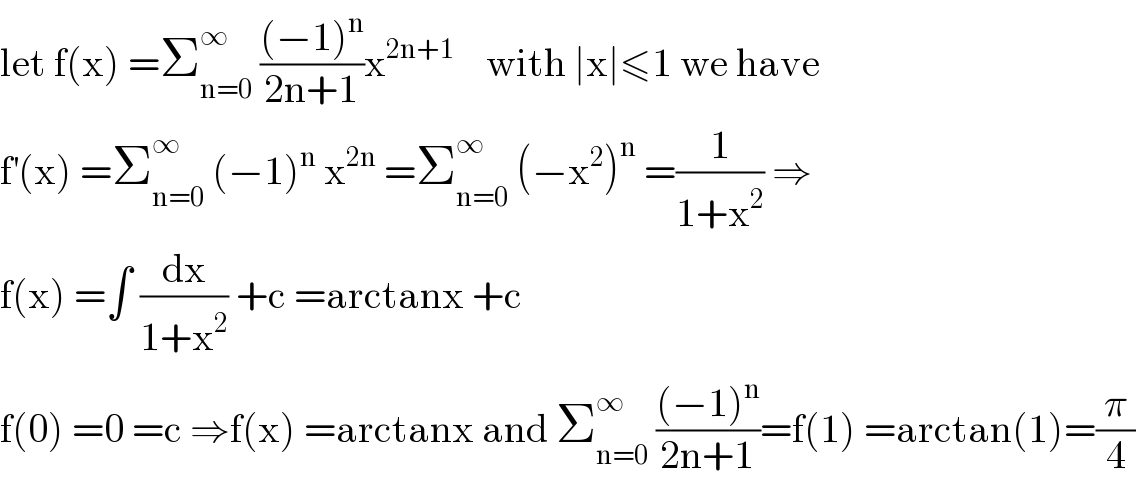
let f(x) =Σ_(n=0) ^∞ (((−1)^n )/(2n+1))x^(2n+1) with ∣x∣≤1 we have f^′ (x) =Σ_(n=0) ^∞ (−1)^n x^(2n) =Σ_(n=0) ^∞ (−x^2 )^n =(1/(1+x^2 )) ⇒ f(x) =∫ (dx/(1+x^2 )) +c =arctanx +c f(0) =0 =c ⇒f(x) =arctanx and Σ_(n=0) ^∞ (((−1)^n )/(2n+1))=f(1) =arctan(1)=(π/4)

$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{2n}+\mathrm{1}}\mathrm{x}^{\mathrm{2n}+\mathrm{1}} \:\:\:\:\mathrm{with}\:\mid\mathrm{x}\mid\leqslant\mathrm{1}\:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{f}^{'} \left(\mathrm{x}\right)\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{x}^{\mathrm{2n}} \:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{x}^{\mathrm{2}} \right)^{\mathrm{n}} \:=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\:=\int\:\frac{\mathrm{dx}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:+\mathrm{c}\:=\mathrm{arctanx}\:+\mathrm{c} \\ $$$$\mathrm{f}\left(\mathrm{0}\right)\:=\mathrm{0}\:=\mathrm{c}\:\Rightarrow\mathrm{f}\left(\mathrm{x}\right)\:=\mathrm{arctanx}\:\mathrm{and}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{2n}+\mathrm{1}}=\mathrm{f}\left(\mathrm{1}\right)\:=\mathrm{arctan}\left(\mathrm{1}\right)=\frac{\pi}{\mathrm{4}} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply