Menu Close

Part-1-S-ln-a-bi-a-b-R-i-2-1-is-S-C-b-0-Part-2-t-ln-ln-ln-k-k-R-k-gt-1-t-C-is-t-undefined-

Tinku Tara 0 Comments
Pin




Question Number 5091 by FilupSmith last updated on 11/Apr/16
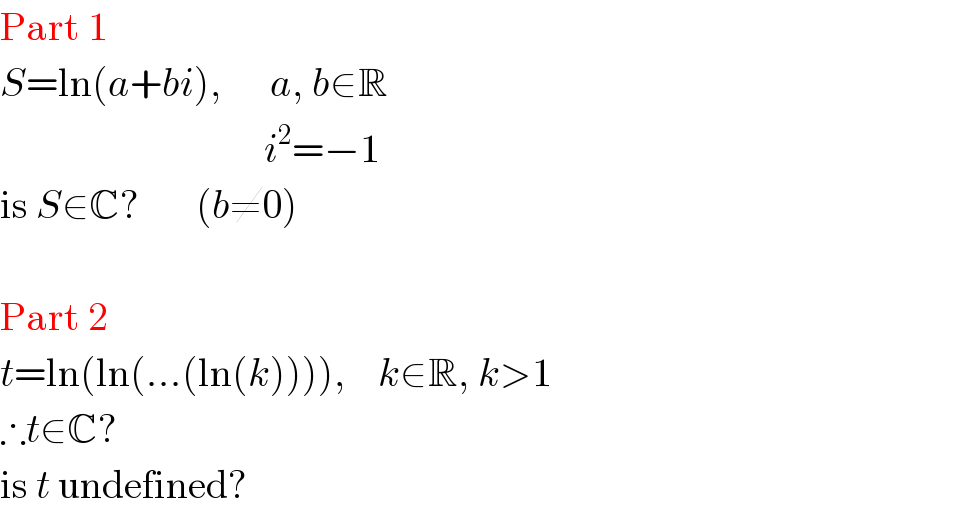
Part 1 S=ln(a+bi), a, b∈R i^2 =−1 is S∈C? (b≠0) Part 2 t=ln(ln(...(ln(k)))), k∈R, k>1 ∴t∈C? is t undefined?
$$\mathrm{Part}\:\mathrm{1} \\ $$$${S}=\mathrm{ln}\left({a}+{bi}\right),\:\:\:\:\:\:{a},\:{b}\in\mathbb{R} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{i}^{\mathrm{2}} =−\mathrm{1} \\ $$$$\mathrm{is}\:{S}\in\mathbb{C}?\:\:\:\:\:\:\:\left({b}\neq\mathrm{0}\right) \\ $$$$ \\ $$$$\mathrm{Part}\:\mathrm{2} \\ $$$${t}=\mathrm{ln}\left(\mathrm{ln}\left(…\left(\mathrm{ln}\left({k}\right)\right)\right)\right),\:\:\:\:{k}\in\mathbb{R},\:{k}>\mathrm{1} \\ $$$$\therefore{t}\in\mathbb{C}? \\ $$$$\mathrm{is}\:{t}\:\mathrm{undefined}? \\ $$
Commented by prakash jain last updated on 11/Apr/16
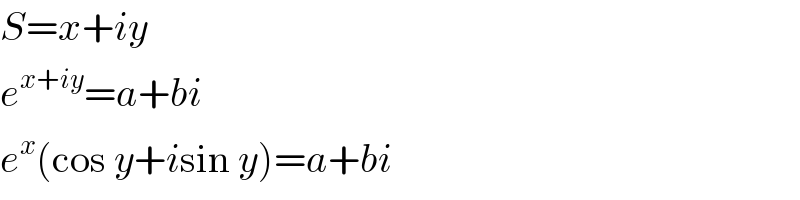
S=x+iy e^(x+iy) =a+bi e^x (cos y+isin y)=a+bi
$${S}={x}+{iy} \\ $$$${e}^{{x}+{iy}} ={a}+{bi} \\ $$$${e}^{{x}} \left(\mathrm{cos}\:{y}+{i}\mathrm{sin}\:{y}\right)={a}+{bi} \\ $$
Commented by 123456 last updated on 11/Apr/16
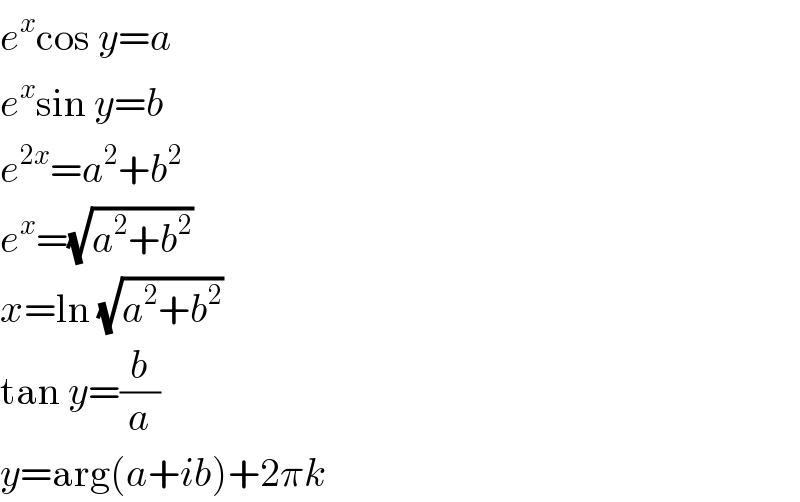
e^x cos y=a e^x sin y=b e^(2x) =a^2 +b^2 e^x =(√(a^2 +b^2 )) x=ln (√(a^2 +b^2 )) tan y=(b/a) y=arg(a+ib)+2πk
$${e}^{{x}} \mathrm{cos}\:{y}={a} \\ $$$${e}^{{x}} \mathrm{sin}\:{y}={b} \\ $$$${e}^{\mathrm{2}{x}} ={a}^{\mathrm{2}} +{b}^{\mathrm{2}} \\ $$$${e}^{{x}} =\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} } \\ $$$${x}=\mathrm{ln}\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} } \\ $$$$\mathrm{tan}\:{y}=\frac{{b}}{{a}} \\ $$$${y}=\mathrm{arg}\left({a}+{ib}\right)+\mathrm{2}\pi{k} \\ $$
Commented by Yozzii last updated on 12/Apr/16
After a certain number of successive ln−applications to k>1, the result is negative so that the ln−applications thereafter give t having a complex result. t would become undefined if ever t_n =0 in t_(n+1) =lnt_n , t_1 =lnk, n≥1. Suppose after k+1 ln−applications t_(k+1) <0.⇒lnt_k <0⇒0<t_k <1. lnt_k =(−lnt_k )(−1+0)=(−lnt_k )(cosπ+isinπ) lnt_k =(−lnt_k )e^(πi) =t_(k+1) ⇒t_(k+2) =lnt_(k+1) =ln((−lnt_k )e^(πi) ) t_(k+2) =ln(−lnt_k )+πi ⇒t_(k+2) ∈C. If {t_n } converges we′d need to solve u=e^u where for sufficiently large n, t_(n+1) =t_(n+2) =....=u.
$${After}\:{a}\:{certain}\:{number}\:{of} \\ $$$${successive}\:{ln}−{applications}\:{to}\:{k}>\mathrm{1},\:{the}\:{result} \\ $$$${is}\:{negative}\:{so}\:{that}\:{the}\:{ln}−{applications} \\ $$$${thereafter}\:{give}\:{t}\:{having}\:{a}\:{complex} \\ $$$${result}.\:{t}\:{would}\:{become}\:{undefined}\:{if} \\ $$$${ever}\:{t}_{{n}} =\mathrm{0}\:{in}\:{t}_{{n}+\mathrm{1}} ={lnt}_{{n}} \:,\:{t}_{\mathrm{1}} ={lnk},\:{n}\geqslant\mathrm{1}. \\ $$$$ \\ $$$${Suppose}\:{after}\:{k}+\mathrm{1}\:{ln}−{applications} \\ $$$${t}_{{k}+\mathrm{1}} <\mathrm{0}.\Rightarrow{lnt}_{{k}} <\mathrm{0}\Rightarrow\mathrm{0}<{t}_{{k}} <\mathrm{1}. \\ $$$${lnt}_{{k}} =\left(−{lnt}_{{k}} \right)\left(−\mathrm{1}+\mathrm{0}\right)=\left(−{lnt}_{{k}} \right)\left({cos}\pi+{isin}\pi\right) \\ $$$${lnt}_{{k}} =\left(−{lnt}_{{k}} \right){e}^{\pi{i}} ={t}_{{k}+\mathrm{1}} \\ $$$$\Rightarrow{t}_{{k}+\mathrm{2}} ={lnt}_{{k}+\mathrm{1}} ={ln}\left(\left(−{lnt}_{{k}} \right){e}^{\pi{i}} \right) \\ $$$${t}_{{k}+\mathrm{2}} ={ln}\left(−{lnt}_{{k}} \right)+\pi{i} \\ $$$$\Rightarrow{t}_{{k}+\mathrm{2}} \in\mathbb{C}. \\ $$$${If}\:\left\{{t}_{{n}} \right\}\:{converges}\:{we}'{d}\:{need}\:{to}\:{solve} \\ $$$${u}={e}^{{u}} \:\:{where}\:{for}\:{sufficiently}\:{large}\:{n}, \\ $$$${t}_{{n}+\mathrm{1}} ={t}_{{n}+\mathrm{2}} =….={u}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *