Menu Close

Question-183295

Tinku Tara 0 Comments
Pin




Question Number 183295 by ajfour last updated on 24/Dec/22
Commented by ajfour last updated on 24/Dec/22
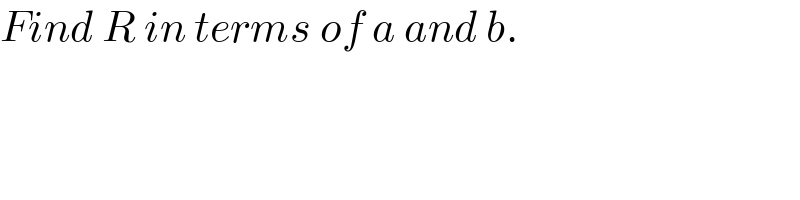
Find R in terms of a and b.
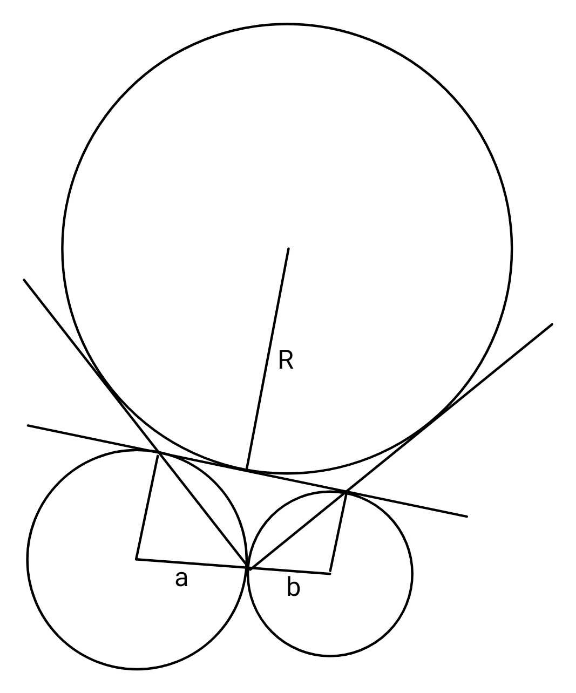
$${Find}\:{R}\:{in}\:{terms}\:{of}\:{a}\:{and}\:{b}. \\ $$
Answered by ajfour last updated on 24/Dec/22
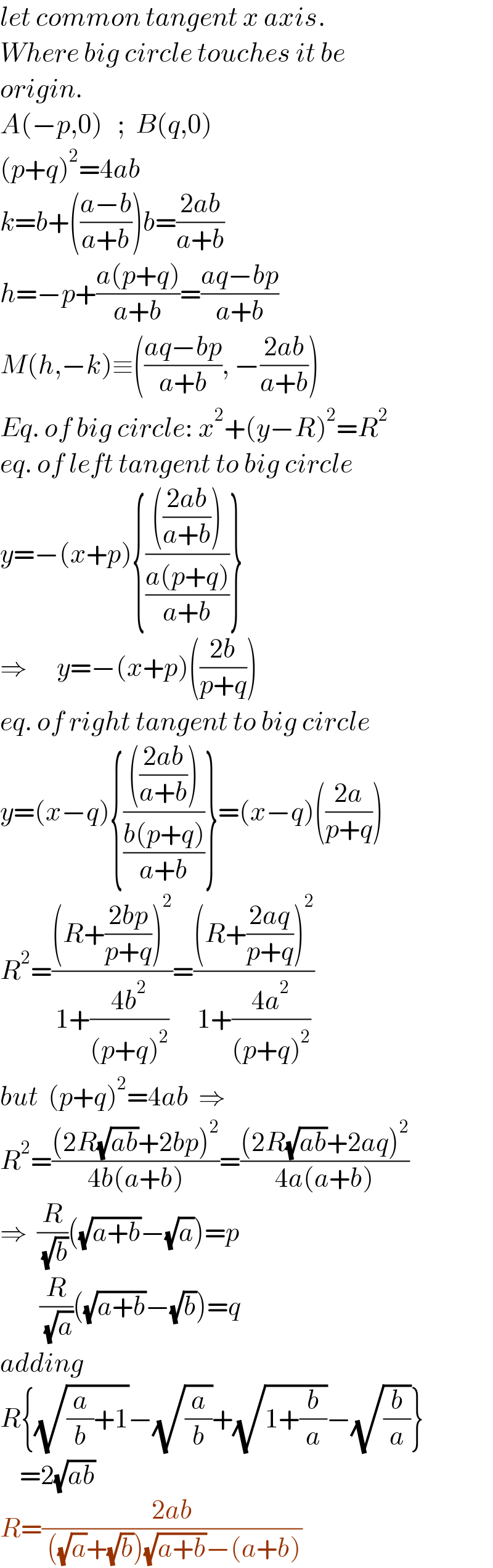
let common tangent x axis. Where big circle touches it be origin. A(−p,0) ; B(q,0) (p+q)^2 =4ab k=b+(((a−b)/(a+b)))b=((2ab)/(a+b)) h=−p+((a(p+q))/(a+b))=((aq−bp)/(a+b)) M(h,−k)≡(((aq−bp)/(a+b)), −((2ab)/(a+b))) Eq. of big circle: x^2 +(y−R)^2 =R^2 eq. of left tangent to big circle y=−(x+p){(((((2ab)/(a+b))))/((a(p+q))/(a+b)))} ⇒ y=−(x+p)(((2b)/(p+q))) eq. of right tangent to big circle y=(x−q){(((((2ab)/(a+b))))/((b(p+q))/(a+b)))}=(x−q)(((2a)/(p+q))) R^2 =(((R+((2bp)/(p+q)))^2 )/(1+((4b^2 )/((p+q)^2 ))))=(((R+((2aq)/(p+q)))^2 )/(1+((4a^2 )/((p+q)^2 )))) but (p+q)^2 =4ab ⇒ R^2 =(((2R(√(ab))+2bp)^2 )/(4b(a+b)))=(((2R(√(ab))+2aq)^2 )/(4a(a+b))) ⇒ (R/( (√b)))((√(a+b))−(√a))=p (R/( (√a)))((√(a+b))−(√b))=q adding R{(√((a/b)+1))−(√(a/b))+(√(1+(b/a)))−(√(b/a))} =2(√(ab)) R=((2ab)/( ((√a)+(√b))(√(a+b))−(a+b)))
$${let}\:{common}\:{tangent}\:{x}\:{axis}. \\ $$$${Where}\:{big}\:{circle}\:{touches}\:{it}\:{be} \\ $$$${origin}. \\ $$$${A}\left(−{p},\mathrm{0}\right)\:\:\:;\:\:{B}\left({q},\mathrm{0}\right) \\ $$$$\left({p}+{q}\right)^{\mathrm{2}} =\mathrm{4}{ab} \\ $$$${k}={b}+\left(\frac{{a}−{b}}{{a}+{b}}\right){b}=\frac{\mathrm{2}{ab}}{{a}+{b}} \\ $$$${h}=−{p}+\frac{{a}\left({p}+{q}\right)}{{a}+{b}}=\frac{{aq}−{bp}}{{a}+{b}} \\ $$$${M}\left({h},−{k}\right)\equiv\left(\frac{{aq}−{bp}}{{a}+{b}},\:−\frac{\mathrm{2}{ab}}{{a}+{b}}\right) \\ $$$${Eq}.\:{of}\:{big}\:{circle}:\:{x}^{\mathrm{2}} +\left({y}−{R}\right)^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$${eq}.\:{of}\:{left}\:{tangent}\:{to}\:{big}\:{circle} \\ $$$${y}=−\left({x}+{p}\right)\left\{\frac{\left(\frac{\mathrm{2}{ab}}{{a}+{b}}\right)}{\frac{{a}\left({p}+{q}\right)}{{a}+{b}}}\right\} \\ $$$$\Rightarrow\:\:\:\:\:\:{y}=−\left({x}+{p}\right)\left(\frac{\mathrm{2}{b}}{{p}+{q}}\right) \\ $$$${eq}.\:{of}\:{right}\:{tangent}\:{to}\:{big}\:{circle} \\ $$$${y}=\left({x}−{q}\right)\left\{\frac{\left(\frac{\mathrm{2}{ab}}{{a}+{b}}\right)}{\frac{{b}\left({p}+{q}\right)}{{a}+{b}}}\right\}=\left({x}−{q}\right)\left(\frac{\mathrm{2}{a}}{{p}+{q}}\right) \\ $$$${R}^{\mathrm{2}} =\frac{\left({R}+\frac{\mathrm{2}{bp}}{{p}+{q}}\right)^{\mathrm{2}} }{\mathrm{1}+\frac{\mathrm{4}{b}^{\mathrm{2}} }{\left({p}+{q}\right)^{\mathrm{2}} }}=\frac{\left({R}+\frac{\mathrm{2}{aq}}{{p}+{q}}\right)^{\mathrm{2}} }{\mathrm{1}+\frac{\mathrm{4}{a}^{\mathrm{2}} }{\left({p}+{q}\right)^{\mathrm{2}} }} \\ $$$${but}\:\:\left({p}+{q}\right)^{\mathrm{2}} =\mathrm{4}{ab}\:\:\Rightarrow \\ $$$${R}^{\mathrm{2}} =\frac{\left(\mathrm{2}{R}\sqrt{{ab}}+\mathrm{2}{bp}\right)^{\mathrm{2}} }{\mathrm{4}{b}\left({a}+{b}\right)}=\frac{\left(\mathrm{2}{R}\sqrt{{ab}}+\mathrm{2}{aq}\right)^{\mathrm{2}} }{\mathrm{4}{a}\left({a}+{b}\right)} \\ $$$$\Rightarrow\:\:\frac{{R}}{\:\sqrt{{b}}}\left(\sqrt{{a}+{b}}−\sqrt{{a}}\right)={p} \\ $$$$\:\:\:\:\:\:\:\:\frac{{R}}{\:\sqrt{{a}}}\left(\sqrt{{a}+{b}}−\sqrt{{b}}\right)={q} \\ $$$${adding} \\ $$$${R}\left\{\sqrt{\frac{{a}}{{b}}+\mathrm{1}}−\sqrt{\frac{{a}}{{b}}}+\sqrt{\mathrm{1}+\frac{{b}}{{a}}}−\sqrt{\frac{{b}}{{a}}}\right\} \\ $$$$\:\:\:\:=\mathrm{2}\sqrt{{ab}} \\ $$$${R}=\frac{\mathrm{2}{ab}}{\:\left(\sqrt{{a}}+\sqrt{{b}}\right)\sqrt{{a}+{b}}−\left({a}+{b}\right)} \\ $$
Commented by mr W last updated on 24/Dec/22
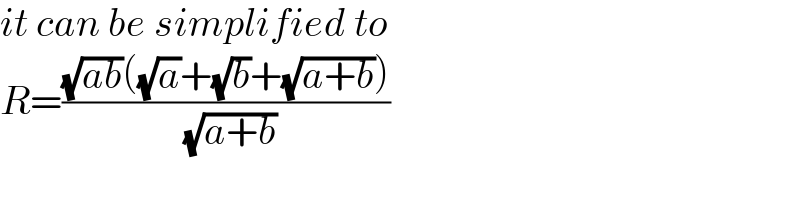
it can be simplified to R=(((√(ab))((√a)+(√b)+(√(a+b))))/( (√(a+b))))
$${it}\:{can}\:{be}\:{simplified}\:{to} \\ $$$${R}=\frac{\sqrt{{ab}}\left(\sqrt{{a}}+\sqrt{{b}}+\sqrt{{a}+{b}}\right)}{\:\sqrt{{a}+{b}}} \\ $$
Answered by mr W last updated on 24/Dec/22
Commented by mr W last updated on 24/Dec/22
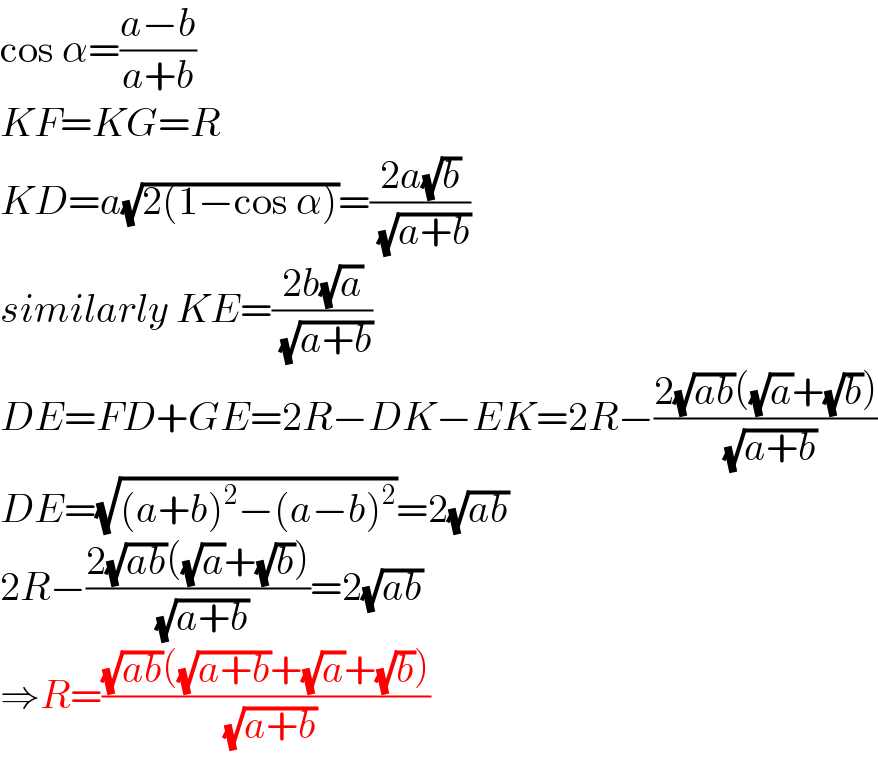
cos α=((a−b)/(a+b)) KF=KG=R KD=a(√(2(1−cos α)))=((2a(√b))/( (√(a+b)))) similarly KE=((2b(√a))/( (√(a+b)))) DE=FD+GE=2R−DK−EK=2R−((2(√(ab))((√a)+(√b)))/( (√(a+b)))) DE=(√((a+b)^2 −(a−b)^2 ))=2(√(ab)) 2R−((2(√(ab))((√a)+(√b)))/( (√(a+b))))=2(√(ab)) ⇒R=(((√(ab))((√(a+b))+(√a)+(√b)))/( (√(a+b))))
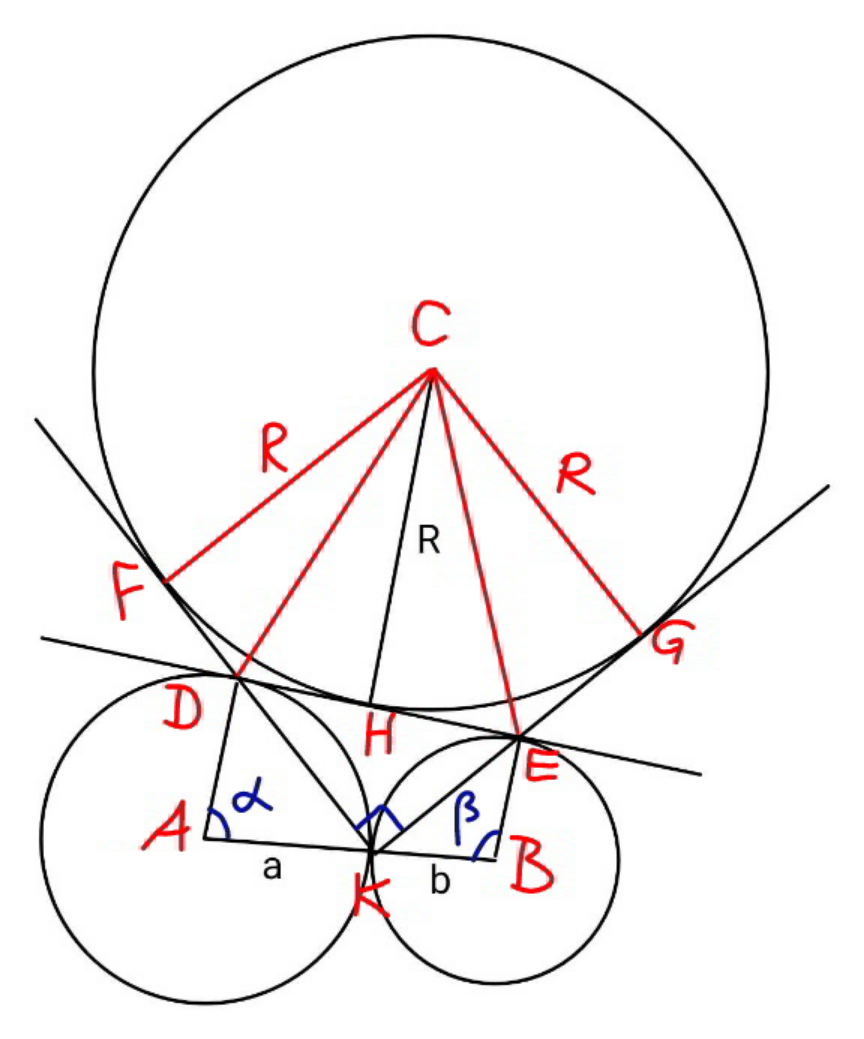
$$\mathrm{cos}\:\alpha=\frac{{a}−{b}}{{a}+{b}} \\ $$$${KF}={KG}={R} \\ $$$${KD}={a}\sqrt{\mathrm{2}\left(\mathrm{1}−\mathrm{cos}\:\alpha\right)}=\frac{\mathrm{2}{a}\sqrt{{b}}}{\:\sqrt{{a}+{b}}} \\ $$$${similarly}\:{KE}=\frac{\mathrm{2}{b}\sqrt{{a}}}{\:\sqrt{{a}+{b}}} \\ $$$${DE}={FD}+{GE}=\mathrm{2}{R}−{DK}−{EK}=\mathrm{2}{R}−\frac{\mathrm{2}\sqrt{{ab}}\left(\sqrt{{a}}+\sqrt{{b}}\right)}{\:\sqrt{{a}+{b}}} \\ $$$${DE}=\sqrt{\left({a}+{b}\right)^{\mathrm{2}} −\left({a}−{b}\right)^{\mathrm{2}} }=\mathrm{2}\sqrt{{ab}} \\ $$$$\mathrm{2}{R}−\frac{\mathrm{2}\sqrt{{ab}}\left(\sqrt{{a}}+\sqrt{{b}}\right)}{\:\sqrt{{a}+{b}}}=\mathrm{2}\sqrt{{ab}} \\ $$$$\Rightarrow{R}=\frac{\sqrt{{ab}}\left(\sqrt{{a}+{b}}+\sqrt{{a}}+\sqrt{{b}}\right)}{\:\sqrt{{a}+{b}}} \\ $$
Commented by ajfour last updated on 24/Dec/22
Thanks Sir. Excellent way!
$${Thanks}\:{Sir}.\:{Excellent}\:{way}! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *