Question Number 117934 by Ar Brandon last updated on 14/Oct/20
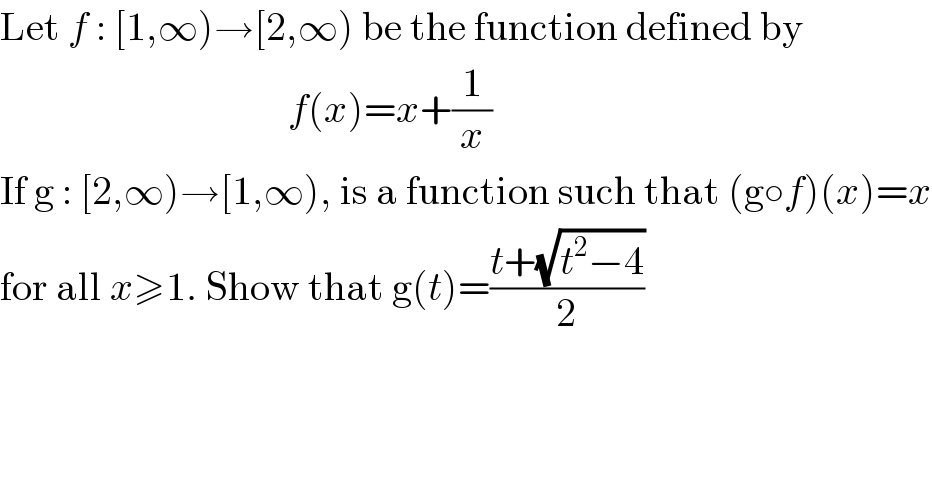
$$\mathrm{Let}\:{f}\::\:\left[\mathrm{1},\infty\right)\rightarrow\left[\mathrm{2},\infty\right)\:\mathrm{be}\:\mathrm{the}\:\mathrm{function}\:\mathrm{defined}\:\mathrm{by} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{f}\left({x}\right)={x}+\frac{\mathrm{1}}{{x}} \\ $$$$\mathrm{If}\:\mathrm{g}\::\:\left[\mathrm{2},\infty\right)\rightarrow\left[\mathrm{1},\infty\right),\:\mathrm{is}\:\mathrm{a}\:\mathrm{function}\:\mathrm{such}\:\mathrm{that}\:\left(\mathrm{g}\circ{f}\right)\left({x}\right)={x} \\ $$$$\mathrm{for}\:\mathrm{all}\:{x}\geqslant\mathrm{1}.\:\mathrm{Show}\:\mathrm{that}\:\mathrm{g}\left({t}\right)=\frac{{t}+\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}} \\ $$
Answered by Lordose last updated on 14/Oct/20
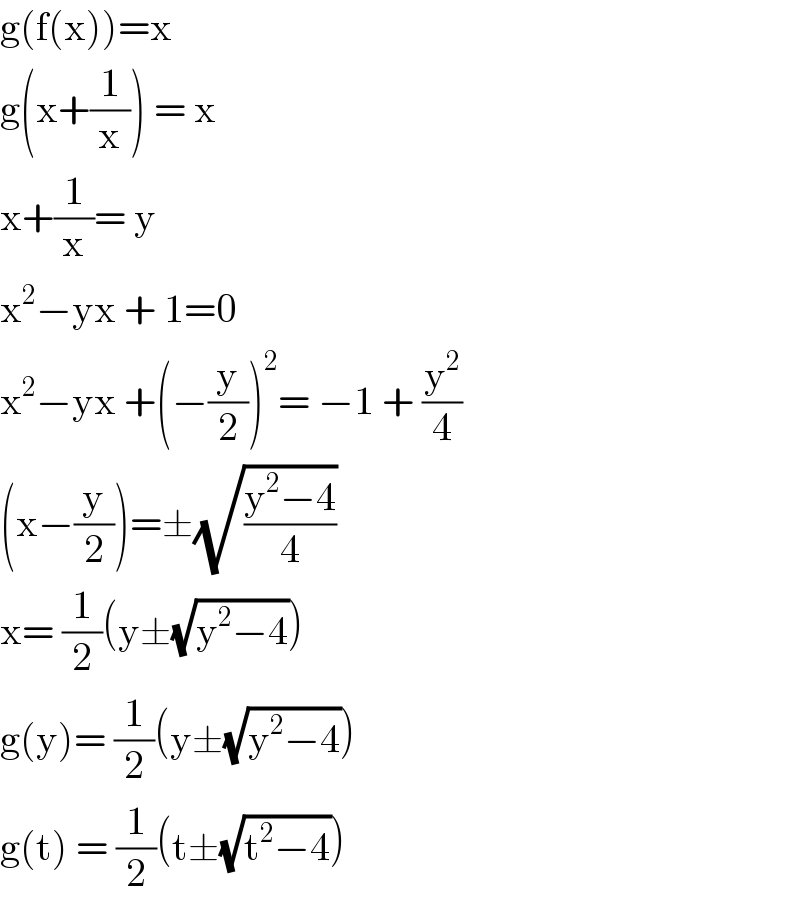
$$\mathrm{g}\left(\mathrm{f}\left(\mathrm{x}\right)\right)=\mathrm{x} \\ $$$$\mathrm{g}\left(\mathrm{x}+\frac{\mathrm{1}}{\mathrm{x}}\right)\:=\:\mathrm{x} \\ $$$$\mathrm{x}+\frac{\mathrm{1}}{\mathrm{x}}=\:\mathrm{y} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{yx}\:+\:\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{yx}\:+\left(−\frac{\mathrm{y}}{\mathrm{2}}\right)^{\mathrm{2}} =\:−\mathrm{1}\:+\:\frac{\mathrm{y}^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\left(\mathrm{x}−\frac{\mathrm{y}}{\mathrm{2}}\right)=\pm\sqrt{\frac{\mathrm{y}^{\mathrm{2}} −\mathrm{4}}{\mathrm{4}}} \\ $$$$\mathrm{x}=\:\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{y}\pm\sqrt{\mathrm{y}^{\mathrm{2}} −\mathrm{4}}\right) \\ $$$$\mathrm{g}\left(\mathrm{y}\right)=\:\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{y}\pm\sqrt{\mathrm{y}^{\mathrm{2}} −\mathrm{4}}\right) \\ $$$$\mathrm{g}\left(\mathrm{t}\right)\:=\:\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{t}\pm\sqrt{\mathrm{t}^{\mathrm{2}} −\mathrm{4}}\right) \\ $$
Commented by Ar Brandon last updated on 14/Oct/20
Thank You ��
