Question Number 184240 by ajfour last updated on 04/Jan/23
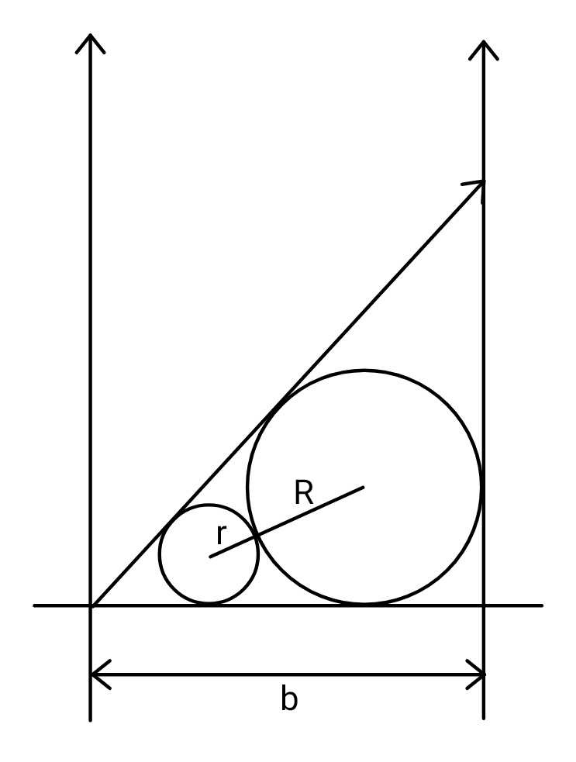
Commented by ajfour last updated on 04/Jan/23
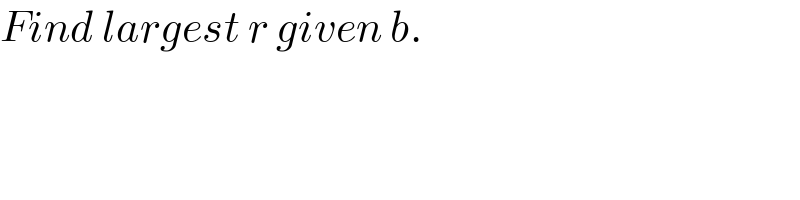
$${Find}\:{largest}\:{r}\:{given}\:{b}. \\ $$
Answered by mr W last updated on 04/Jan/23
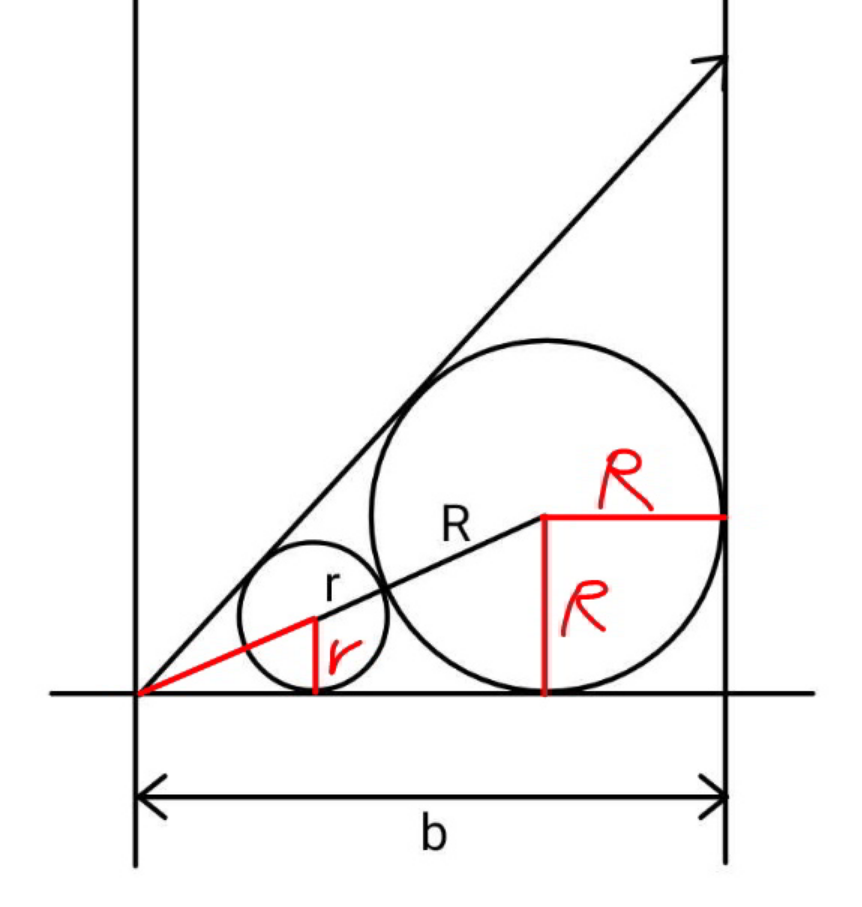
Commented by mr W last updated on 04/Jan/23
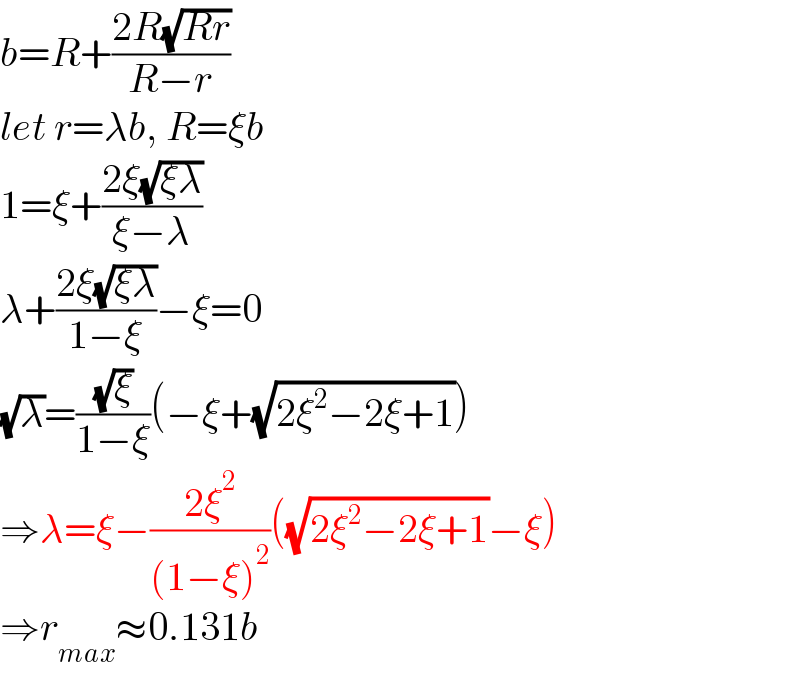
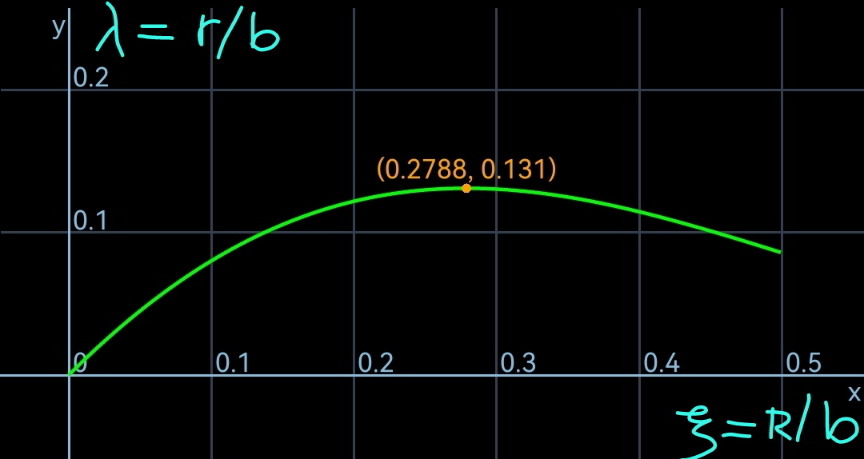
$${b}={R}+\frac{\mathrm{2}{R}\sqrt{{Rr}}}{{R}−{r}} \\ $$$${let}\:{r}=\lambda{b},\:{R}=\xi{b} \\ $$$$\mathrm{1}=\xi+\frac{\mathrm{2}\xi\sqrt{\xi\lambda}}{\xi−\lambda} \\ $$$$\lambda+\frac{\mathrm{2}\xi\sqrt{\xi\lambda}}{\mathrm{1}−\xi}−\xi=\mathrm{0} \\ $$$$\sqrt{\lambda}=\frac{\sqrt{\xi}}{\mathrm{1}−\xi}\left(−\xi+\sqrt{\mathrm{2}\xi^{\mathrm{2}} −\mathrm{2}\xi+\mathrm{1}}\right) \\ $$$$\Rightarrow\lambda=\xi−\frac{\mathrm{2}\xi^{\mathrm{2}} }{\left(\mathrm{1}−\xi\right)^{\mathrm{2}} }\left(\sqrt{\mathrm{2}\xi^{\mathrm{2}} −\mathrm{2}\xi+\mathrm{1}}−\xi\right) \\ $$$$\Rightarrow{r}_{{max}} \approx\mathrm{0}.\mathrm{131}{b} \\ $$
Commented by mr W last updated on 04/Jan/23
Answered by ajfour last updated on 04/Jan/23
−2) =m^2 (m−(1/m))(1+(1/m^2 )) ⇒ (3m^2 −1)(m−(1/m)−2) =(m^2 +1)(m−(1/m)) or 1−(2/((m−(1/m))))=((m^2 +1)/(3(m^2 −(1/3)))) ⇒ (m^2 −1)(m−(1/m))=(3m^2 −1) ⇒ (m^2 −1)^2 =m(3m^2 −1) ⇒ m≈0.6855](https://www.tinkutara.com/question/Q184254.png)
$$\mathrm{cos}\:\mathrm{2}\theta=\frac{{R}−{r}}{{R}+{r}} \\ $$$$\Rightarrow\:\:{r}={R}\left(\frac{\mathrm{1}−\mathrm{cos}\:\mathrm{2}\theta}{\mathrm{1}+\mathrm{cos}\:\mathrm{2}\theta}\right)={R}\mathrm{tan}\:^{\mathrm{2}} \theta \\ $$$${b}={r}\mathrm{tan}\:\mathrm{2}\theta+\mathrm{2}\sqrt{{Rr}}+{R} \\ $$$${say}\:\:\mathrm{tan}\:\theta={m} \\ $$$$\:\:{b}={R}\left(\frac{\mathrm{2}{m}^{\mathrm{3}} }{\mathrm{1}−{m}^{\mathrm{2}} }+\mathrm{2}{m}+\mathrm{1}\right) \\ $$$${r}=\frac{{bm}^{\mathrm{2}} }{\frac{\mathrm{2}{m}^{\mathrm{3}} }{\mathrm{1}−{m}^{\mathrm{2}} }+\mathrm{2}{m}+\mathrm{1}}=\frac{{bm}^{\mathrm{2}} \left({m}^{\mathrm{2}} −\mathrm{1}\right)}{{m}^{\mathrm{2}} −\mathrm{2}{m}−\mathrm{1}} \\ $$$$\Rightarrow\:\frac{{r}}{{b}}\:=\frac{{m}^{\mathrm{2}} \left({m}−\frac{\mathrm{1}}{{m}}\right)}{{m}−\frac{\mathrm{1}}{{m}}−\mathrm{2}} \\ $$$$\:\:\:{r}_{{max}} =\mathrm{0}.\mathrm{13103}{b} \\ $$$$\frac{{dr}}{{dm}}=\mathrm{0}\:\:\Rightarrow \\ $$$$\left[\mathrm{2}{m}\left({m}−\frac{\mathrm{1}}{{m}}\right)+{m}^{\mathrm{2}} \left(\mathrm{1}+\frac{\mathrm{1}}{{m}^{\mathrm{2}} }\right)\right]\left({m}−\frac{\mathrm{1}}{{m}}−\mathrm{2}\right) \\ $$$$\:\:\:={m}^{\mathrm{2}} \left({m}−\frac{\mathrm{1}}{{m}}\right)\left(\mathrm{1}+\frac{\mathrm{1}}{{m}^{\mathrm{2}} }\right) \\ $$$$\Rightarrow\:\left(\mathrm{3}{m}^{\mathrm{2}} −\mathrm{1}\right)\left({m}−\frac{\mathrm{1}}{{m}}−\mathrm{2}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:=\left({m}^{\mathrm{2}} +\mathrm{1}\right)\left({m}−\frac{\mathrm{1}}{{m}}\right) \\ $$$${or}\:\:\:\:\mathrm{1}−\frac{\mathrm{2}}{\left({m}−\frac{\mathrm{1}}{{m}}\right)}=\frac{{m}^{\mathrm{2}} +\mathrm{1}}{\mathrm{3}\left({m}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{3}}\right)} \\ $$$$\Rightarrow\:\:\left({m}^{\mathrm{2}} −\mathrm{1}\right)\left({m}−\frac{\mathrm{1}}{{m}}\right)=\left(\mathrm{3}{m}^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\Rightarrow\:\:\left({m}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} ={m}\left(\mathrm{3}{m}^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\Rightarrow\:\:{m}\approx\mathrm{0}.\mathrm{6855} \\ $$$$ \\ $$
Commented by mr W last updated on 04/Jan/23
$${great}!\:{you}\:{got}\:{it}\:{even}\:{exactly}! \\ $$
