Menu Close

In-xy-plane-which-of-the-following-is-the-reflection-of-the-graph-of-y-1-x-x-2-1-about-the-line-y-2x-

Tinku Tara 0 Comments
Pin




Question Number 118874 by bemath last updated on 20/Oct/20
In xy−plane , which of the following is the reflection of the graph of y = ((1+x)/(x^2 +1)) about the line y=2x.
$${In}\:{xy}−{plane}\:,\:{which}\:{of}\:{the}\:{following} \\ $$$${is}\:{the}\:{reflection}\:{of}\:{the}\:{graph} \\ $$$${of}\:{y}\:=\:\frac{\mathrm{1}+{x}}{{x}^{\mathrm{2}} +\mathrm{1}}\:{about}\:{the}\:{line}\:{y}=\mathrm{2}{x}.\: \\ $$
Answered by bramlexs22 last updated on 20/Oct/20
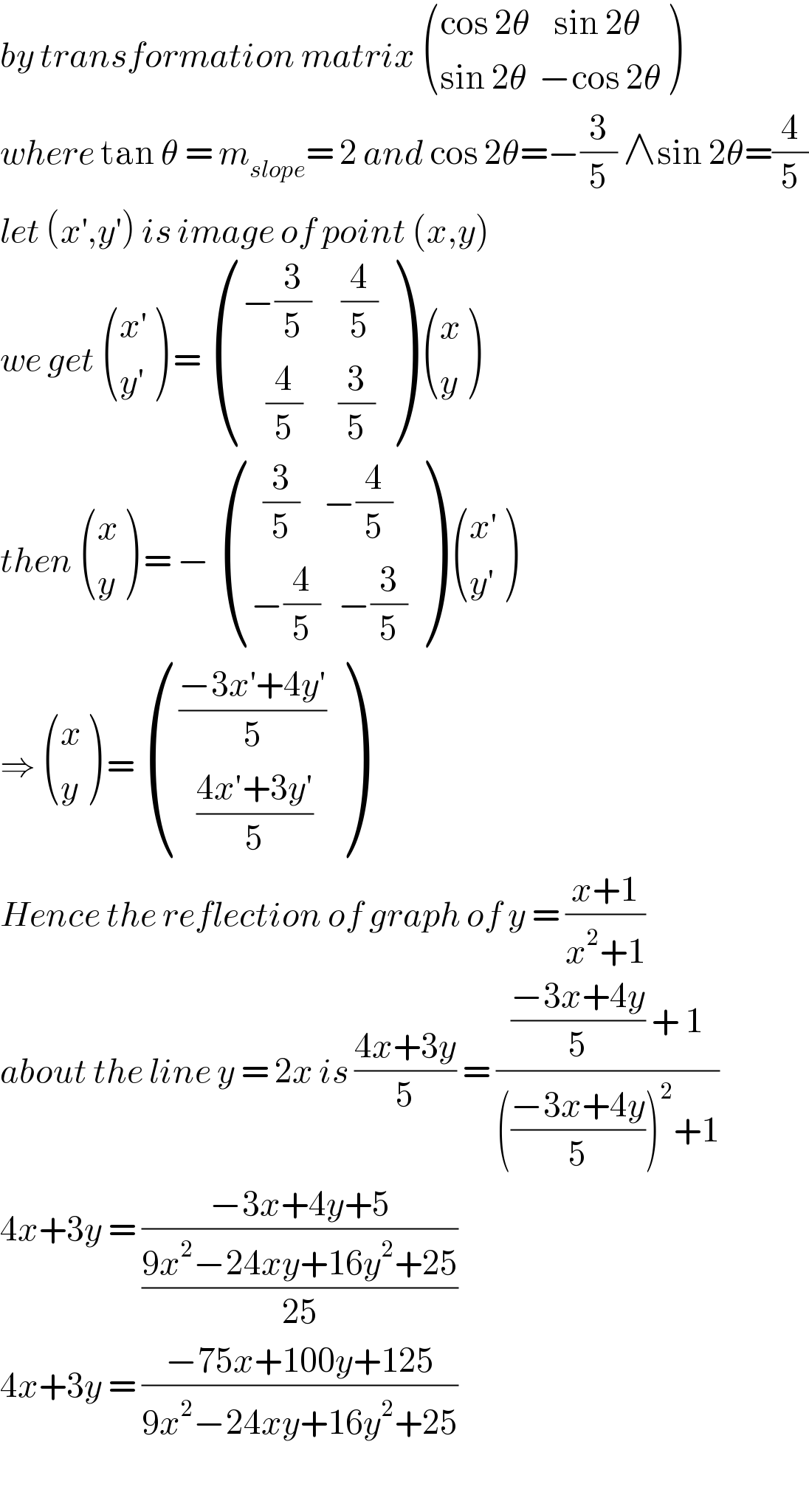
by transformation matrix (((cos 2θ sin 2θ)),((sin 2θ −cos 2θ)) ) where tan θ = m_(slope) = 2 and cos 2θ=−(3/5) ∧sin 2θ=(4/5) let (x^′ ,y^′ ) is image of point (x,y) we get (((x′)),((y′)) ) = (((−(3/5) (4/5))),(( (4/5) (3/5))) ) ((x),(y) ) then ((x),(y) ) = − ((( (3/5) −(4/5))),((−(4/5) −(3/5))) ) (((x′)),((y′)) ) ⇒ ((x),(y) ) = ((((−3x^′ +4y′)/5)),(( ((4x′+3y′)/5))) ) Hence the reflection of graph of y = ((x+1)/(x^2 +1)) about the line y = 2x is ((4x+3y)/5) = ((((−3x+4y)/5) + 1)/((((−3x+4y)/5))^2 +1)) 4x+3y = ((−3x+4y+5)/((9x^2 −24xy+16y^2 +25)/(25))) 4x+3y = ((−75x+100y+125)/(9x^2 −24xy+16y^2 +25))
$${by}\:{transformation}\:{matrix}\:\begin{pmatrix}{\mathrm{cos}\:\mathrm{2}\theta\:\:\:\:\mathrm{sin}\:\mathrm{2}\theta}\\{\mathrm{sin}\:\mathrm{2}\theta\:\:−\mathrm{cos}\:\mathrm{2}\theta}\end{pmatrix} \\ $$$${where}\:\mathrm{tan}\:\theta\:=\:{m}_{{slope}} =\:\mathrm{2}\:{and}\:\mathrm{cos}\:\mathrm{2}\theta=−\frac{\mathrm{3}}{\mathrm{5}}\:\wedge\mathrm{sin}\:\mathrm{2}\theta=\frac{\mathrm{4}}{\mathrm{5}} \\ $$$${let}\:\left({x}^{'} ,{y}^{'} \right)\:{is}\:{image}\:{of}\:{point}\:\left({x},{y}\right) \\ $$$${we}\:{get}\:\begin{pmatrix}{{x}'}\\{{y}'}\end{pmatrix}\:=\:\begin{pmatrix}{−\frac{\mathrm{3}}{\mathrm{5}}\:\:\:\:\:\frac{\mathrm{4}}{\mathrm{5}}}\\{\:\:\:\:\frac{\mathrm{4}}{\mathrm{5}}\:\:\:\:\:\:\frac{\mathrm{3}}{\mathrm{5}}}\end{pmatrix}\:\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix} \\ $$$${then}\:\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:=\:−\:\begin{pmatrix}{\:\:\frac{\mathrm{3}}{\mathrm{5}}\:\:\:\:−\frac{\mathrm{4}}{\mathrm{5}}}\\{−\frac{\mathrm{4}}{\mathrm{5}}\:\:\:−\frac{\mathrm{3}}{\mathrm{5}}}\end{pmatrix}\:\begin{pmatrix}{{x}'}\\{{y}'}\end{pmatrix} \\ $$$$\Rightarrow\:\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:=\:\begin{pmatrix}{\frac{−\mathrm{3}{x}^{'} +\mathrm{4}{y}'}{\mathrm{5}}}\\{\:\:\:\frac{\mathrm{4}{x}'+\mathrm{3}{y}'}{\mathrm{5}}}\end{pmatrix} \\ $$$${Hence}\:{the}\:{reflection}\:{of}\:{graph}\:{of}\:{y}\:=\:\frac{{x}+\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$$${about}\:{the}\:{line}\:{y}\:=\:\mathrm{2}{x}\:{is}\:\frac{\mathrm{4}{x}+\mathrm{3}{y}}{\mathrm{5}}\:=\:\frac{\frac{−\mathrm{3}{x}+\mathrm{4}{y}}{\mathrm{5}}\:+\:\mathrm{1}}{\left(\frac{−\mathrm{3}{x}+\mathrm{4}{y}}{\mathrm{5}}\right)^{\mathrm{2}} +\mathrm{1}} \\ $$$$\mathrm{4}{x}+\mathrm{3}{y}\:=\:\frac{−\mathrm{3}{x}+\mathrm{4}{y}+\mathrm{5}}{\frac{\mathrm{9}{x}^{\mathrm{2}} −\mathrm{24}{xy}+\mathrm{16}{y}^{\mathrm{2}} +\mathrm{25}}{\mathrm{25}}} \\ $$$$\mathrm{4}{x}+\mathrm{3}{y}\:=\:\frac{−\mathrm{75}{x}+\mathrm{100}{y}+\mathrm{125}}{\mathrm{9}{x}^{\mathrm{2}} −\mathrm{24}{xy}+\mathrm{16}{y}^{\mathrm{2}} +\mathrm{25}} \\ $$$$ \\ $$
Commented by benjo_mathlover last updated on 20/Oct/20

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *