Menu Close

let-x-y-z-be-positive-real-numbers-such-that-x-y-z-1-Determine-the-minimum-value-of-1-x-4-y-9-z-

Tinku Tara 0 Comments
Pin




Question Number 119303 by bobhans last updated on 23/Oct/20
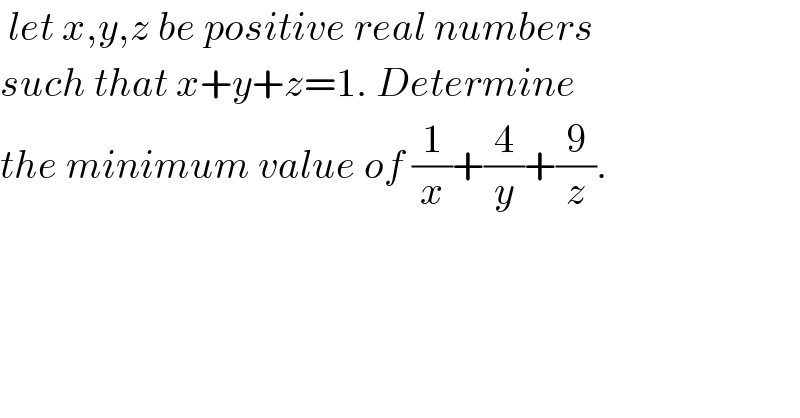
let x,y,z be positive real numbers such that x+y+z=1. Determine the minimum value of (1/x)+(4/y)+(9/z).
$$\:{let}\:{x},{y},{z}\:{be}\:{positive}\:{real}\:{numbers}\: \\ $$$${such}\:{that}\:{x}+{y}+{z}=\mathrm{1}.\:{Determine}\: \\ $$$${the}\:{minimum}\:{value}\:{of}\:\frac{\mathrm{1}}{{x}}+\frac{\mathrm{4}}{{y}}+\frac{\mathrm{9}}{{z}}. \\ $$
Answered by TANMAY PANACEA last updated on 23/Oct/20
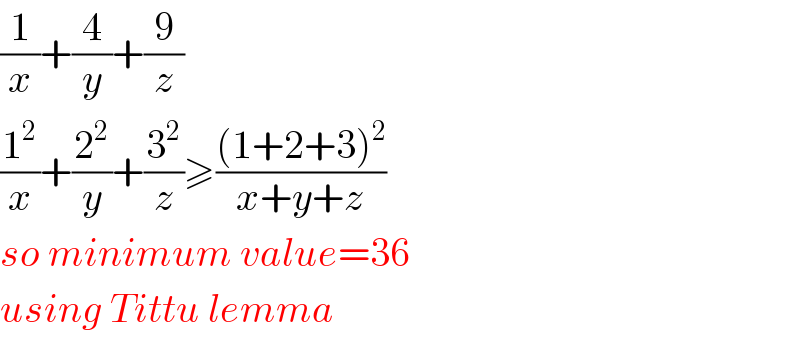
(1/x)+(4/y)+(9/z) (1^2 /x)+(2^2 /y)+(3^2 /z)≥(((1+2+3)^2 )/(x+y+z)) so minimum value=36 using Tittu lemma
$$\frac{\mathrm{1}}{{x}}+\frac{\mathrm{4}}{{y}}+\frac{\mathrm{9}}{{z}} \\ $$$$\frac{\mathrm{1}^{\mathrm{2}} }{{x}}+\frac{\mathrm{2}^{\mathrm{2}} }{{y}}+\frac{\mathrm{3}^{\mathrm{2}} }{{z}}\geqslant\frac{\left(\mathrm{1}+\mathrm{2}+\mathrm{3}\right)^{\mathrm{2}} }{{x}+{y}+{z}} \\ $$$${so}\:{minimum}\:{value}=\mathrm{36} \\ $$$${using}\:{Tittu}\:{lemma} \\ $$
Answered by bobhans last updated on 24/Oct/20
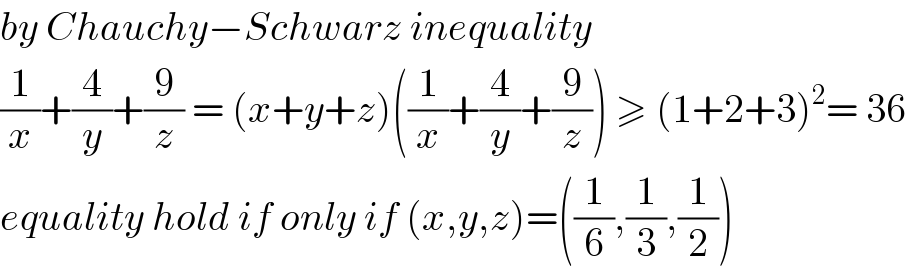
by Chauchy−Schwarz inequality (1/x)+(4/y)+(9/z) = (x+y+z)((1/x)+(4/y)+(9/z)) ≥ (1+2+3)^2 = 36 equality hold if only if (x,y,z)=((1/6),(1/3),(1/2))
$${by}\:{Chauchy}−{Schwarz}\:{inequality} \\ $$$$\frac{\mathrm{1}}{{x}}+\frac{\mathrm{4}}{{y}}+\frac{\mathrm{9}}{{z}}\:=\:\left({x}+{y}+{z}\right)\left(\frac{\mathrm{1}}{{x}}+\frac{\mathrm{4}}{{y}}+\frac{\mathrm{9}}{{z}}\right)\:\geqslant\:\left(\mathrm{1}+\mathrm{2}+\mathrm{3}\right)^{\mathrm{2}} =\:\mathrm{36} \\ $$$${equality}\:{hold}\:{if}\:{only}\:{if}\:\left({x},{y},{z}\right)=\left(\frac{\mathrm{1}}{\mathrm{6}},\frac{\mathrm{1}}{\mathrm{3}},\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$
Answered by 1549442205PVT last updated on 24/Oct/20
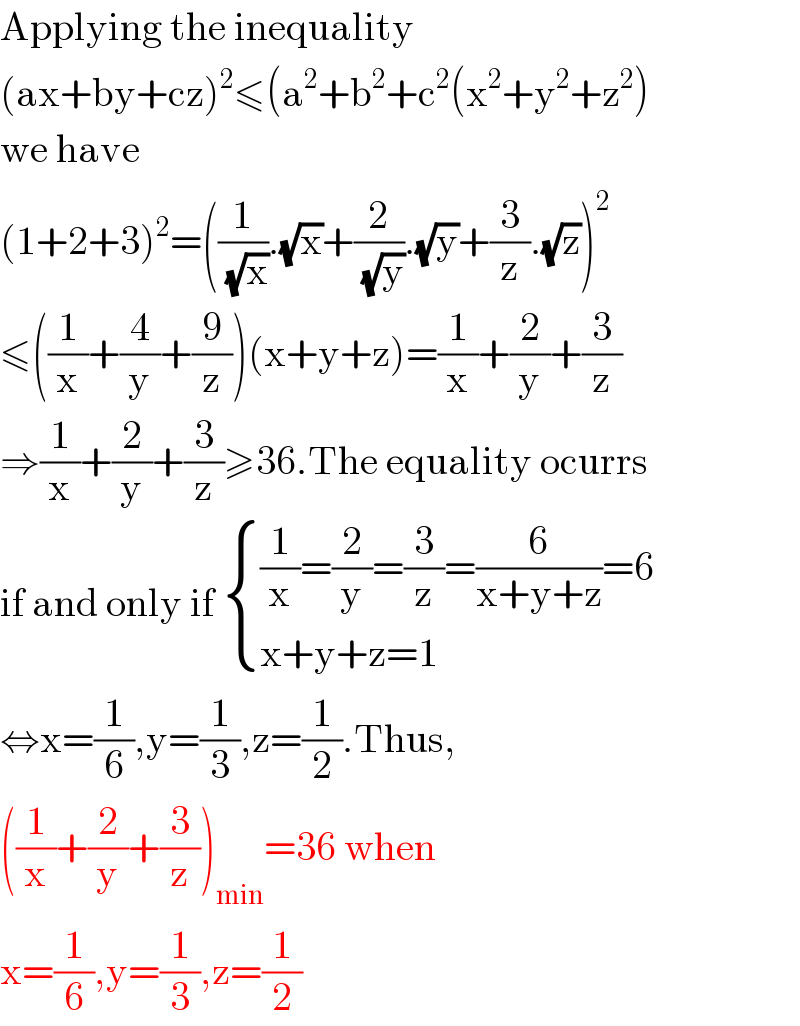
Applying the inequality (ax+by+cz)^2 ≤(a^2 +b^2 +c^2 (x^2 +y^2 +z^2 ) we have (1+2+3)^2 =((1/( (√x))).(√x)+(2/( (√y))).(√y)+(3/z).(√z))^2 ≤((1/x)+(4/y)+(9/z))(x+y+z)=(1/x)+(2/y)+(3/z) ⇒(1/x)+(2/y)+(3/z)≥36.The equality ocurrs if and only if { (((1/x)=(2/y)=(3/z)=(6/(x+y+z))=6)),((x+y+z=1)) :} ⇔x=(1/6),y=(1/3),z=(1/2).Thus, ((1/x)+(2/y)+(3/z))_(min) =36 when x=(1/6),y=(1/3),z=(1/2)
$$\mathrm{Applying}\:\mathrm{the}\:\mathrm{inequality} \\ $$$$\left(\mathrm{ax}+\mathrm{by}+\mathrm{cz}\right)^{\mathrm{2}} \leqslant\left(\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} \left(\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} \right)\right. \\ $$$$\mathrm{we}\:\mathrm{have} \\ $$$$\left(\mathrm{1}+\mathrm{2}+\mathrm{3}\right)^{\mathrm{2}} =\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{x}}}.\sqrt{\mathrm{x}}+\frac{\mathrm{2}}{\:\sqrt{\mathrm{y}}}.\sqrt{\mathrm{y}}+\frac{\mathrm{3}}{\mathrm{z}}.\sqrt{\mathrm{z}}\right)^{\mathrm{2}} \\ $$$$\leqslant\left(\frac{\mathrm{1}}{\mathrm{x}}+\frac{\mathrm{4}}{\mathrm{y}}+\frac{\mathrm{9}}{\mathrm{z}}\right)\left(\mathrm{x}+\mathrm{y}+\mathrm{z}\right)=\frac{\mathrm{1}}{\mathrm{x}}+\frac{\mathrm{2}}{\mathrm{y}}+\frac{\mathrm{3}}{\mathrm{z}} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{x}}+\frac{\mathrm{2}}{\mathrm{y}}+\frac{\mathrm{3}}{\mathrm{z}}\geqslant\mathrm{36}.\mathrm{The}\:\mathrm{equality}\:\mathrm{ocurrs} \\ $$$$\mathrm{if}\:\mathrm{and}\:\mathrm{only}\:\mathrm{if}\:\begin{cases}{\frac{\mathrm{1}}{\mathrm{x}}=\frac{\mathrm{2}}{\mathrm{y}}=\frac{\mathrm{3}}{\mathrm{z}}=\frac{\mathrm{6}}{\mathrm{x}+\mathrm{y}+\mathrm{z}}=\mathrm{6}}\\{\mathrm{x}+\mathrm{y}+\mathrm{z}=\mathrm{1}}\end{cases} \\ $$$$\Leftrightarrow\mathrm{x}=\frac{\mathrm{1}}{\mathrm{6}},\mathrm{y}=\frac{\mathrm{1}}{\mathrm{3}},\mathrm{z}=\frac{\mathrm{1}}{\mathrm{2}}.\mathrm{Thus}, \\ $$$$\left(\frac{\mathrm{1}}{\mathrm{x}}+\frac{\mathrm{2}}{\mathrm{y}}+\frac{\mathrm{3}}{\mathrm{z}}\right)_{\mathrm{min}} =\mathrm{36}\:\mathrm{when} \\ $$$$\mathrm{x}=\frac{\mathrm{1}}{\mathrm{6}},\mathrm{y}=\frac{\mathrm{1}}{\mathrm{3}},\mathrm{z}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *