Menu Close

Question-53828

Tinku Tara 0 Comments
Pin




Question Number 53828 by ajfour last updated on 26/Jan/19
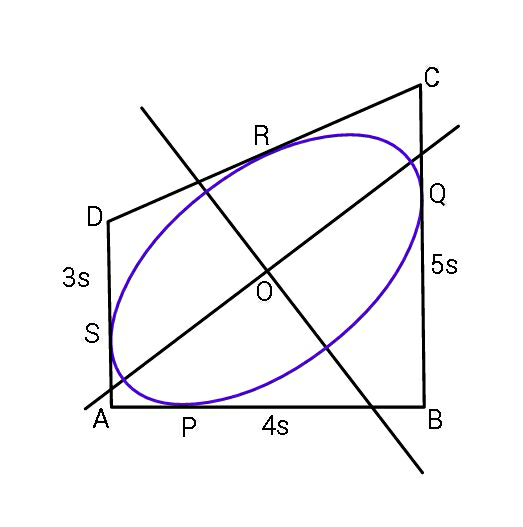
Commented by ajfour last updated on 26/Jan/19
Find a and b of maximum area ellipse within the trapezium.
$${Find}\:{a}\:{and}\:{b}\:{of}\:{maximum}\:{area}\:{ellipse} \\ $$$${within}\:{the}\:{trapezium}. \\ $$
Answered by ajfour last updated on 26/Jan/19
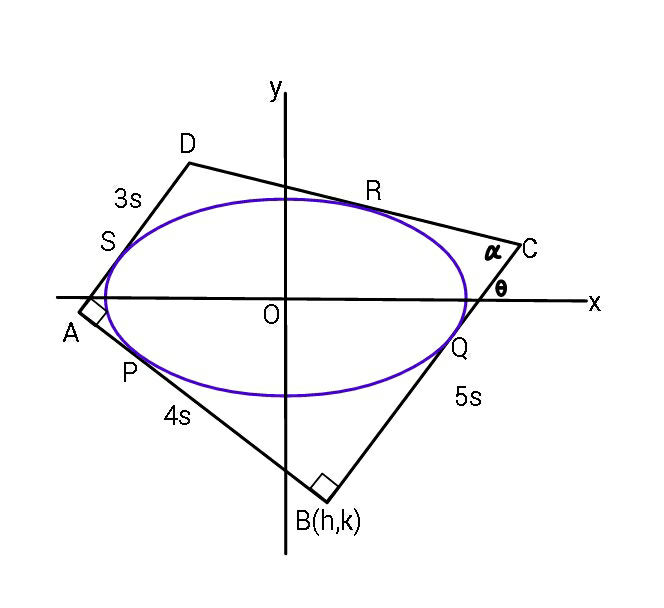
Commented by ajfour last updated on 26/Jan/19
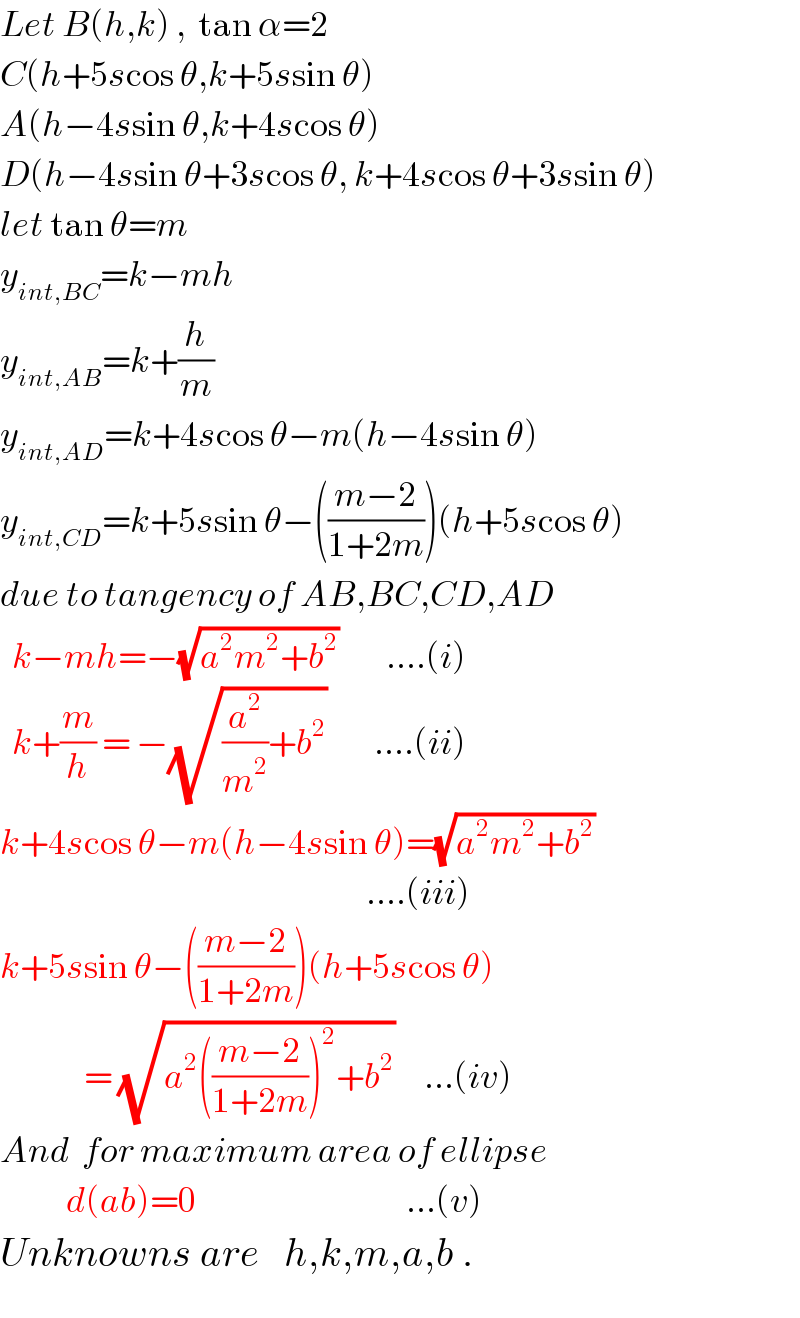
Let B(h,k) , tan α=2 C(h+5scos θ,k+5ssin θ) A(h−4ssin θ,k+4scos θ) D(h−4ssin θ+3scos θ, k+4scos θ+3ssin θ) let tan θ=m y_(int,BC) =k−mh y_(int,AB) =k+(h/m) y_(int,AD) =k+4scos θ−m(h−4ssin θ) y_(int,CD) =k+5ssin θ−(((m−2)/(1+2m)))(h+5scos θ) due to tangency of AB,BC,CD,AD k−mh=−(√(a^2 m^2 +b^2 )) ....(i) k+(m/h) = −(√((a^2 /m^2 )+b^2 )) ....(ii) k+4scos θ−m(h−4ssin θ)=(√(a^2 m^2 +b^2 )) ....(iii) k+5ssin θ−(((m−2)/(1+2m)))(h+5scos θ) = (√(a^2 (((m−2)/(1+2m)))^2 +b^2 )) ...(iv) And for maximum area of ellipse d(ab)=0 ...(v) Unknowns are h,k,m,a,b .
$${Let}\:{B}\left({h},{k}\right)\:,\:\:\mathrm{tan}\:\alpha=\mathrm{2} \\ $$$${C}\left({h}+\mathrm{5}{s}\mathrm{cos}\:\theta,{k}+\mathrm{5}{s}\mathrm{sin}\:\theta\right) \\ $$$${A}\left({h}−\mathrm{4}{s}\mathrm{sin}\:\theta,{k}+\mathrm{4}{s}\mathrm{cos}\:\theta\right) \\ $$$${D}\left({h}−\mathrm{4}{s}\mathrm{sin}\:\theta+\mathrm{3}{s}\mathrm{cos}\:\theta,\:{k}+\mathrm{4}{s}\mathrm{cos}\:\theta+\mathrm{3}{s}\mathrm{sin}\:\theta\right) \\ $$$${let}\:\mathrm{tan}\:\theta={m} \\ $$$${y}_{{int},{BC}} ={k}−{mh} \\ $$$${y}_{{int},{AB}} ={k}+\frac{{h}}{{m}} \\ $$$${y}_{{int},{AD}} ={k}+\mathrm{4}{s}\mathrm{cos}\:\theta−{m}\left({h}−\mathrm{4}{s}\mathrm{sin}\:\theta\right) \\ $$$${y}_{{int},{CD}} ={k}+\mathrm{5}{s}\mathrm{sin}\:\theta−\left(\frac{{m}−\mathrm{2}}{\mathrm{1}+\mathrm{2}{m}}\right)\left({h}+\mathrm{5}{s}\mathrm{cos}\:\theta\right) \\ $$$${due}\:{to}\:{tangency}\:{of}\:{AB},{BC},{CD},{AD} \\ $$$$\:\:{k}−{mh}=−\sqrt{{a}^{\mathrm{2}} {m}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:\:\:\:\:\:\:\:….\left({i}\right) \\ $$$$\:\:{k}+\frac{{m}}{{h}}\:=\:−\sqrt{\frac{{a}^{\mathrm{2}} }{{m}^{\mathrm{2}} }+{b}^{\mathrm{2}} }\:\:\:\:\:\:\:\:….\left({ii}\right) \\ $$$${k}+\mathrm{4}{s}\mathrm{cos}\:\theta−{m}\left({h}−\mathrm{4}{s}\mathrm{sin}\:\theta\right)=\sqrt{{a}^{\mathrm{2}} {m}^{\mathrm{2}} +{b}^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:….\left({iii}\right) \\ $$$${k}+\mathrm{5}{s}\mathrm{sin}\:\theta−\left(\frac{{m}−\mathrm{2}}{\mathrm{1}+\mathrm{2}{m}}\right)\left({h}+\mathrm{5}{s}\mathrm{cos}\:\theta\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\sqrt{{a}^{\mathrm{2}} \left(\frac{{m}−\mathrm{2}}{\mathrm{1}+\mathrm{2}{m}}\right)^{\mathrm{2}} +{b}^{\mathrm{2}} }\:\:\:\:\:…\left({iv}\right) \\ $$$${And}\:\:{for}\:{maximum}\:{area}\:{of}\:{ellipse} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:{d}\left({ab}\right)=\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:…\left({v}\right) \\ $$$${Unknowns}\:{are}\:\:\:{h},{k},{m},{a},{b}\:. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *