Question Number 120812 by bramlexs22 last updated on 03/Nov/20
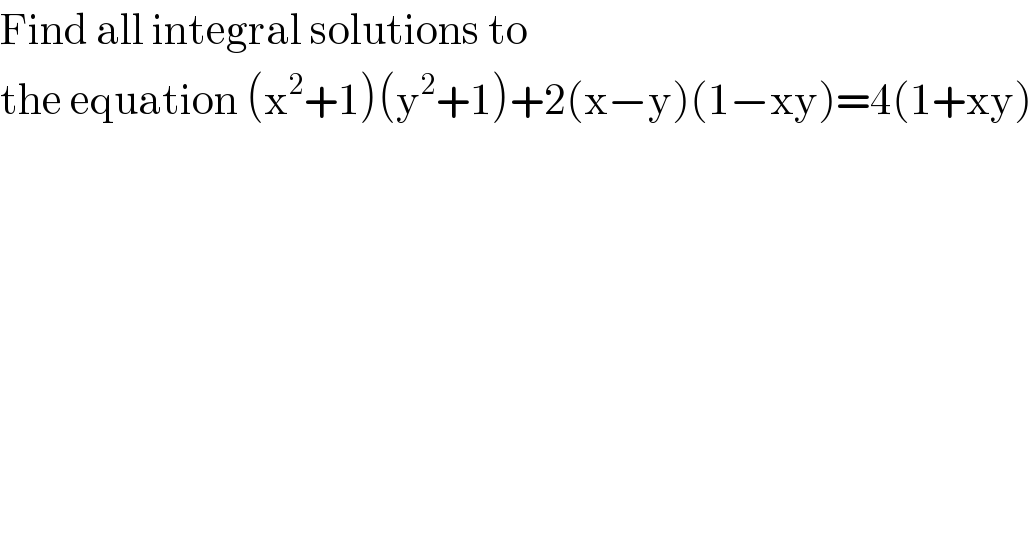
$$\mathrm{Find}\:\mathrm{all}\:\mathrm{integral}\:\mathrm{solutions}\:\mathrm{to}\: \\ $$$$\mathrm{the}\:\mathrm{equation}\:\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)\left(\mathrm{y}^{\mathrm{2}} +\mathrm{1}\right)+\mathrm{2}\left(\mathrm{x}−\mathrm{y}\right)\left(\mathrm{1}−\mathrm{xy}\right)=\mathrm{4}\left(\mathrm{1}+\mathrm{xy}\right) \\ $$
Answered by liberty last updated on 03/Nov/20
![⇔ (xy−1)^2 +(x−y)^2 −2(x−y)(xy−1)=4 ⇔ [ xy−1−(x−y) ]^2 = 4 ⇔ (x+1)(y+1)= ±2 case(1) (x+1)(y+1)=2 → { ((x+1=2)),((y+1=1)) :} , { ((x+1=1)),((y+1=2)) :} → { ((x+1=−2)),((y+1=−1 )) :}, { ((x+1=−1)),((y+1=−2)) :} we get four solutions are {(1,0),(0,1),(−3,−2),(−2,−3)} case(2) (x+1)(y+1)=−2→ { ((x+1=−2)),((y+1=1)) :}, { ((x+1=1)),((y+1=−2)) :} → { ((x+1=−1)),((y+1=2)) :} , { ((x+1=2)),((y+1=−1)) :} also we get four solutions are { (−2,1),(1,−2),(−3,0),(0,−3)} ∵ complete solutions are { (1,0),(0,1),(−3,−2),(−2,−3),(−2,1),(1,−2),(−3,0),(0,−3)}▲](https://www.tinkutara.com/question/Q120818.png)
$$\Leftrightarrow\:\left(\mathrm{xy}−\mathrm{1}\right)^{\mathrm{2}} +\left(\mathrm{x}−\mathrm{y}\right)^{\mathrm{2}} −\mathrm{2}\left(\mathrm{x}−\mathrm{y}\right)\left(\mathrm{xy}−\mathrm{1}\right)=\mathrm{4} \\ $$$$\Leftrightarrow\:\left[\:\mathrm{xy}−\mathrm{1}−\left(\mathrm{x}−\mathrm{y}\right)\:\right]^{\mathrm{2}} \:=\:\mathrm{4} \\ $$$$\Leftrightarrow\:\left(\mathrm{x}+\mathrm{1}\right)\left(\mathrm{y}+\mathrm{1}\right)=\:\pm\mathrm{2}\: \\ $$$$\mathrm{case}\left(\mathrm{1}\right)\:\left(\mathrm{x}+\mathrm{1}\right)\left(\mathrm{y}+\mathrm{1}\right)=\mathrm{2}\:\rightarrow\begin{cases}{\mathrm{x}+\mathrm{1}=\mathrm{2}}\\{\mathrm{y}+\mathrm{1}=\mathrm{1}}\end{cases}\:\:,\begin{cases}{\mathrm{x}+\mathrm{1}=\mathrm{1}}\\{\mathrm{y}+\mathrm{1}=\mathrm{2}}\end{cases} \\ $$$$\:\:\:\:\:\:\rightarrow\begin{cases}{\mathrm{x}+\mathrm{1}=−\mathrm{2}}\\{\mathrm{y}+\mathrm{1}=−\mathrm{1}\:}\end{cases},\:\begin{cases}{\mathrm{x}+\mathrm{1}=−\mathrm{1}}\\{\mathrm{y}+\mathrm{1}=−\mathrm{2}}\end{cases} \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{four}\:\mathrm{solutions}\:\mathrm{are}\:\left\{\left(\mathrm{1},\mathrm{0}\right),\left(\mathrm{0},\mathrm{1}\right),\left(−\mathrm{3},−\mathrm{2}\right),\left(−\mathrm{2},−\mathrm{3}\right)\right\} \\ $$$$\mathrm{case}\left(\mathrm{2}\right)\:\left(\mathrm{x}+\mathrm{1}\right)\left(\mathrm{y}+\mathrm{1}\right)=−\mathrm{2}\rightarrow\begin{cases}{\mathrm{x}+\mathrm{1}=−\mathrm{2}}\\{\mathrm{y}+\mathrm{1}=\mathrm{1}}\end{cases},\:\begin{cases}{\mathrm{x}+\mathrm{1}=\mathrm{1}}\\{\mathrm{y}+\mathrm{1}=−\mathrm{2}}\end{cases} \\ $$$$\:\:\:\:\:\rightarrow\begin{cases}{\mathrm{x}+\mathrm{1}=−\mathrm{1}}\\{\mathrm{y}+\mathrm{1}=\mathrm{2}}\end{cases}\:,\:\begin{cases}{\mathrm{x}+\mathrm{1}=\mathrm{2}}\\{\mathrm{y}+\mathrm{1}=−\mathrm{1}}\end{cases} \\ $$$$\mathrm{also}\:\mathrm{we}\:\mathrm{get}\:\mathrm{four}\:\mathrm{solutions}\:\mathrm{are}\:\left\{\:\left(−\mathrm{2},\mathrm{1}\right),\left(\mathrm{1},−\mathrm{2}\right),\left(−\mathrm{3},\mathrm{0}\right),\left(\mathrm{0},−\mathrm{3}\right)\right\} \\ $$$$\because\:\mathrm{complete}\:\mathrm{solutions}\: \\ $$$$\mathrm{are}\:\left\{\:\left(\mathrm{1},\mathrm{0}\right),\left(\mathrm{0},\mathrm{1}\right),\left(−\mathrm{3},−\mathrm{2}\right),\left(−\mathrm{2},−\mathrm{3}\right),\left(−\mathrm{2},\mathrm{1}\right),\left(\mathrm{1},−\mathrm{2}\right),\left(−\mathrm{3},\mathrm{0}\right),\left(\mathrm{0},−\mathrm{3}\right)\right\}\blacktriangle \\ $$
Commented by bramlexs22 last updated on 03/Nov/20

$$\mathrm{nice}… \\ $$
