Menu Close

Question-122399

Tinku Tara 0 Comments
Pin




Question Number 122399 by ajfour last updated on 16/Nov/20
Commented by ajfour last updated on 16/Nov/20
Find in terms of radii a and b the minimum length of common tangent EF between the axes.
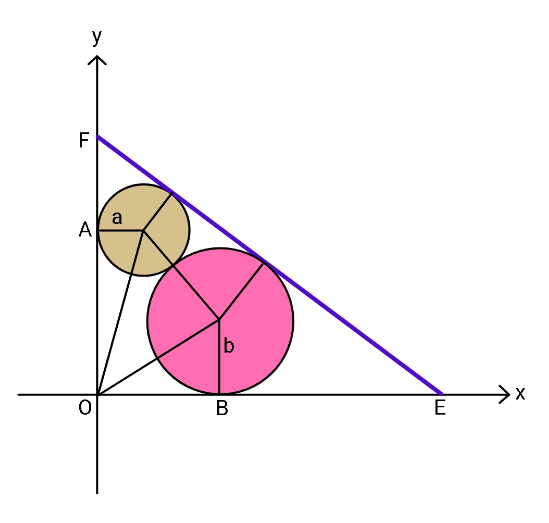
$${Find}\:{in}\:{terms}\:{of}\:{radii}\:{a}\:{and}\:{b} \\ $$$${the}\:{minimum}\:{length}\:{of}\:{common} \\ $$$${tangent}\:{EF}\:{between}\:{the}\:{axes}. \\ $$
Answered by mr W last updated on 16/Nov/20
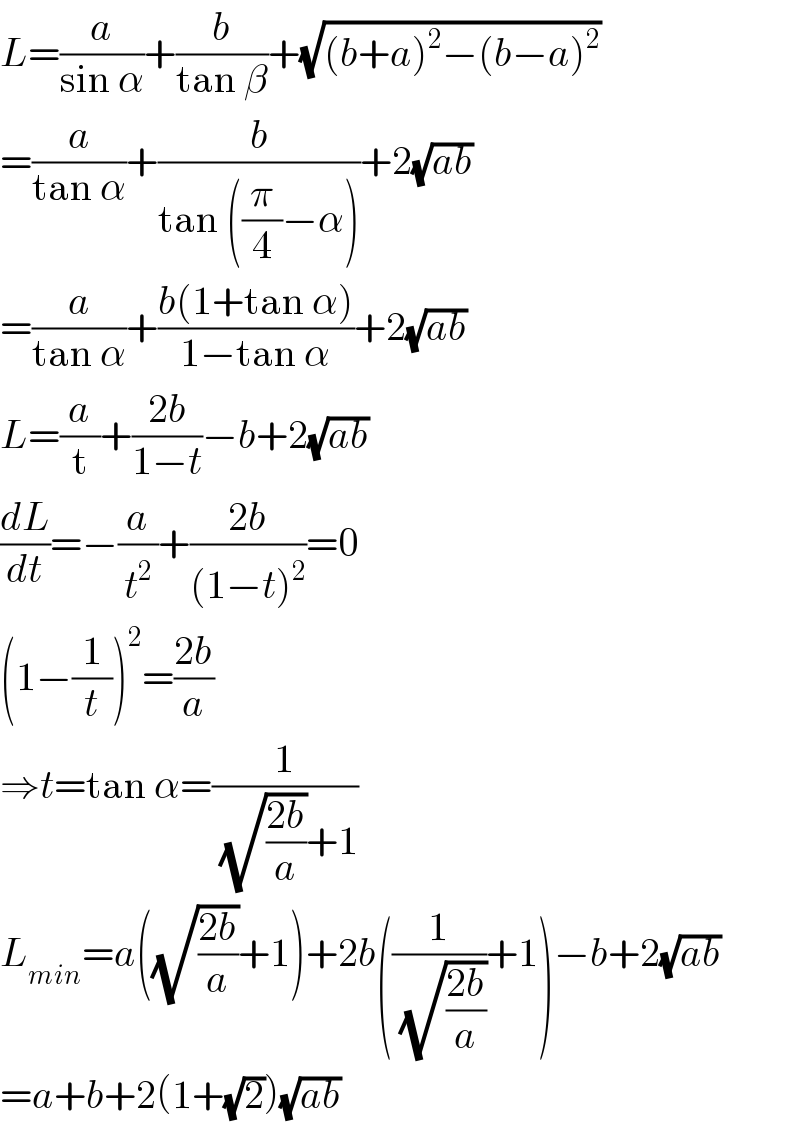
L=(a/(sin α))+(b/(tan β))+(√((b+a)^2 −(b−a)^2 )) =(a/(tan α))+(b/(tan ((π/4)−α)))+2(√(ab)) =(a/(tan α))+((b(1+tan α))/(1−tan α))+2(√(ab)) L=(a/t)+((2b)/(1−t))−b+2(√(ab)) (dL/dt)=−(a/t^2 )+((2b)/((1−t)^2 ))=0 (1−(1/t))^2 =((2b)/a) ⇒t=tan α=(1/( (√((2b)/a))+1)) L_(min) =a((√((2b)/a))+1)+2b((1/( (√((2b)/a))))+1)−b+2(√(ab)) =a+b+2(1+(√2))(√(ab))
$${L}=\frac{{a}}{\mathrm{sin}\:\alpha}+\frac{{b}}{\mathrm{tan}\:\beta}+\sqrt{\left({b}+{a}\right)^{\mathrm{2}} −\left({b}−{a}\right)^{\mathrm{2}} } \\ $$$$=\frac{{a}}{\mathrm{tan}\:\alpha}+\frac{{b}}{\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}−\alpha\right)}+\mathrm{2}\sqrt{{ab}} \\ $$$$=\frac{{a}}{\mathrm{tan}\:\alpha}+\frac{{b}\left(\mathrm{1}+\mathrm{tan}\:\alpha\right)}{\mathrm{1}−\mathrm{tan}\:\alpha}+\mathrm{2}\sqrt{{ab}} \\ $$$${L}=\frac{{a}}{\mathrm{t}}+\frac{\mathrm{2}{b}}{\mathrm{1}−{t}}−{b}+\mathrm{2}\sqrt{{ab}} \\ $$$$\frac{{dL}}{{dt}}=−\frac{{a}}{{t}^{\mathrm{2}} }+\frac{\mathrm{2}{b}}{\left(\mathrm{1}−{t}\right)^{\mathrm{2}} }=\mathrm{0} \\ $$$$\left(\mathrm{1}−\frac{\mathrm{1}}{{t}}\right)^{\mathrm{2}} =\frac{\mathrm{2}{b}}{{a}} \\ $$$$\Rightarrow{t}=\mathrm{tan}\:\alpha=\frac{\mathrm{1}}{\:\sqrt{\frac{\mathrm{2}{b}}{{a}}}+\mathrm{1}} \\ $$$${L}_{{min}} ={a}\left(\sqrt{\frac{\mathrm{2}{b}}{{a}}}+\mathrm{1}\right)+\mathrm{2}{b}\left(\frac{\mathrm{1}}{\:\sqrt{\frac{\mathrm{2}{b}}{{a}}}}+\mathrm{1}\right)−{b}+\mathrm{2}\sqrt{{ab}} \\ $$$$={a}+{b}+\mathrm{2}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)\sqrt{{ab}} \\ $$
Commented by ajfour last updated on 16/Nov/20
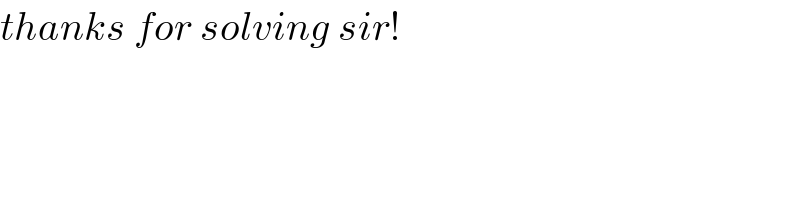
thanks for solving sir!
$${thanks}\:{for}\:{solving}\:{sir}! \\ $$
Answered by ajfour last updated on 16/Nov/20
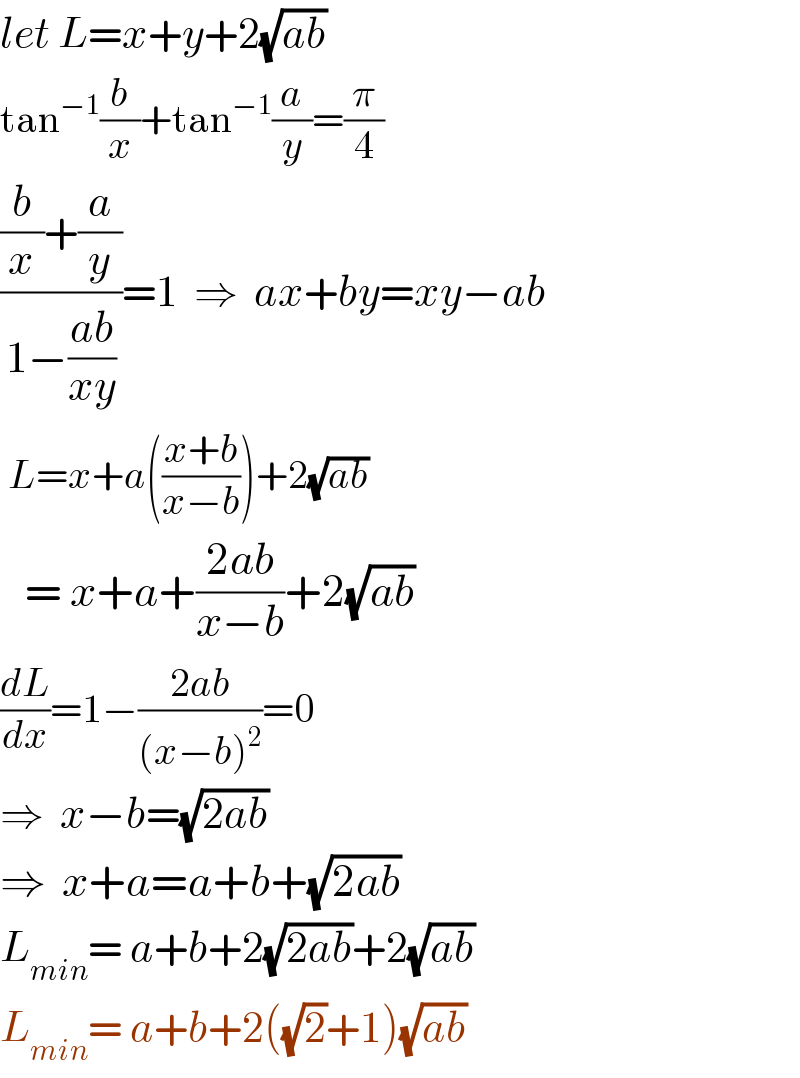
let L=x+y+2(√(ab)) tan^(−1) (b/x)+tan^(−1) (a/y)=(π/4) (((b/x)+(a/y))/(1−((ab)/(xy))))=1 ⇒ ax+by=xy−ab L=x+a(((x+b)/(x−b)))+2(√(ab)) = x+a+((2ab)/(x−b))+2(√(ab)) (dL/dx)=1−((2ab)/((x−b)^2 ))=0 ⇒ x−b=(√(2ab)) ⇒ x+a=a+b+(√(2ab)) L_(min) = a+b+2(√(2ab))+2(√(ab)) L_(min) = a+b+2((√2)+1)(√(ab))
$${let}\:{L}={x}+{y}+\mathrm{2}\sqrt{{ab}} \\ $$$$\mathrm{tan}^{−\mathrm{1}} \frac{{b}}{{x}}+\mathrm{tan}^{−\mathrm{1}} \frac{{a}}{{y}}=\frac{\pi}{\mathrm{4}} \\ $$$$\frac{\frac{{b}}{{x}}+\frac{{a}}{{y}}}{\mathrm{1}−\frac{{ab}}{{xy}}}=\mathrm{1}\:\:\Rightarrow\:\:{ax}+{by}={xy}−{ab} \\ $$$$\:{L}={x}+{a}\left(\frac{{x}+{b}}{{x}−{b}}\right)+\mathrm{2}\sqrt{{ab}} \\ $$$$\:\:\:=\:{x}+{a}+\frac{\mathrm{2}{ab}}{{x}−{b}}+\mathrm{2}\sqrt{{ab}} \\ $$$$\frac{{dL}}{{dx}}=\mathrm{1}−\frac{\mathrm{2}{ab}}{\left({x}−{b}\right)^{\mathrm{2}} }=\mathrm{0} \\ $$$$\Rightarrow\:\:{x}−{b}=\sqrt{\mathrm{2}{ab}}\:\:\: \\ $$$$\Rightarrow\:\:{x}+{a}={a}+{b}+\sqrt{\mathrm{2}{ab}} \\ $$$${L}_{{min}} =\:{a}+{b}+\mathrm{2}\sqrt{\mathrm{2}{ab}}+\mathrm{2}\sqrt{{ab}} \\ $$$${L}_{{min}} =\:{a}+{b}+\mathrm{2}\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)\sqrt{{ab}}\: \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *