Menu Close

calculate-lim-x-0-1-cosx-cos-2x-cos-nx-x-2-with-n-integr-natural-not-0-

Tinku Tara 0 Comments
Pin




Question Number 57405 by Abdo msup. last updated on 03/Apr/19
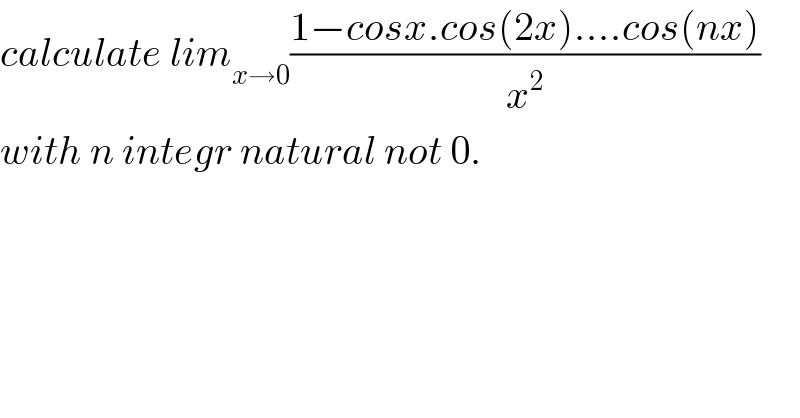
calculate lim_(x→0) ((1−cosx.cos(2x)....cos(nx))/x^2 ) with n integr natural not 0.
$${calculate}\:{lim}_{{x}\rightarrow\mathrm{0}} \frac{\mathrm{1}−{cosx}.{cos}\left(\mathrm{2}{x}\right)….{cos}\left({nx}\right)}{{x}^{\mathrm{2}} } \\ $$$${with}\:{n}\:{integr}\:{natural}\:{not}\:\mathrm{0}. \\ $$
Answered by Smail last updated on 05/Apr/19
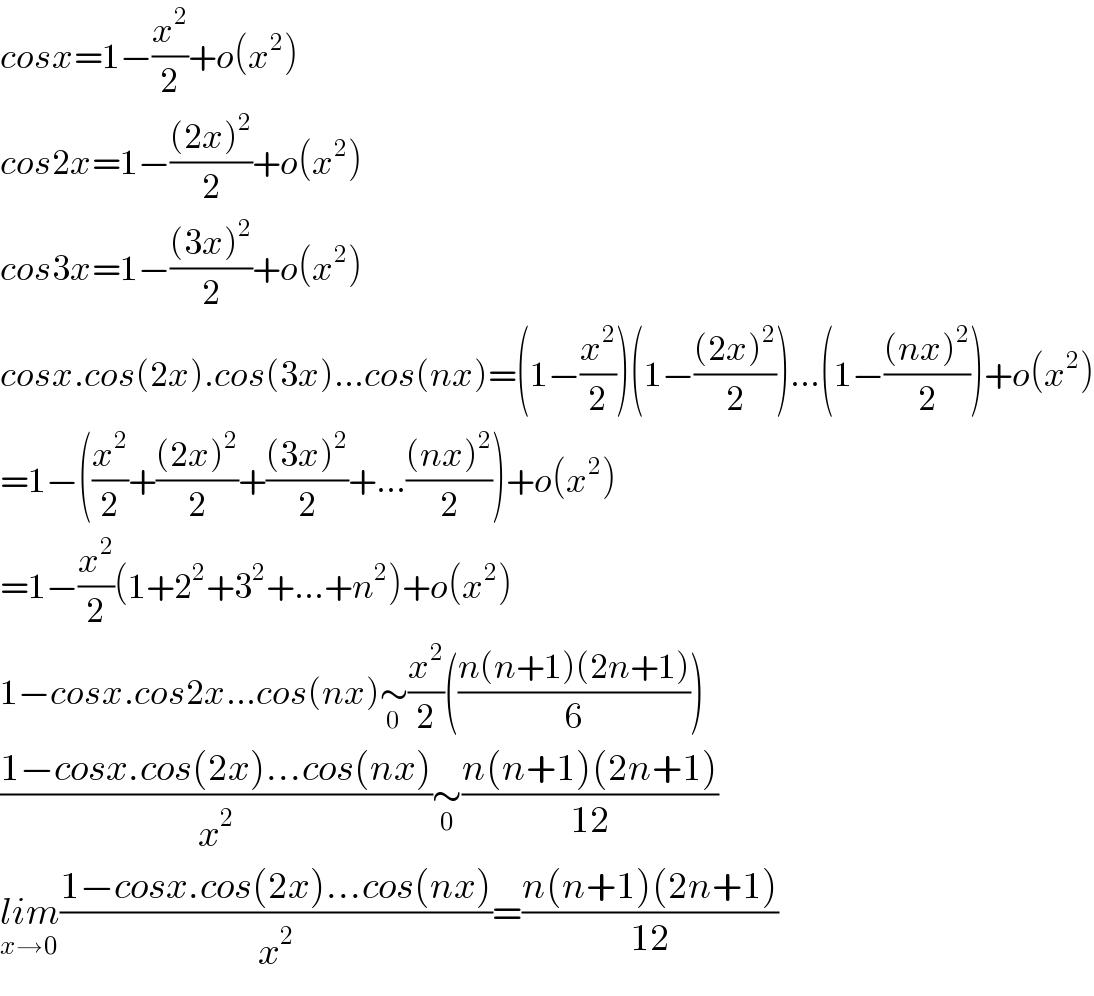
cosx=1−(x^2 /2)+o(x^2 ) cos2x=1−(((2x)^2 )/2)+o(x^2 ) cos3x=1−(((3x)^2 )/2)+o(x^2 ) cosx.cos(2x).cos(3x)...cos(nx)=(1−(x^2 /2))(1−(((2x)^2 )/2))...(1−(((nx)^2 )/2))+o(x^2 ) =1−((x^2 /2)+(((2x)^2 )/2)+(((3x)^2 )/2)+...(((nx)^2 )/2))+o(x^2 ) =1−(x^2 /2)(1+2^2 +3^2 +...+n^2 )+o(x^2 ) 1−cosx.cos2x...cos(nx)∼_0 (x^2 /2)(((n(n+1)(2n+1))/6)) ((1−cosx.cos(2x)...cos(nx))/x^2 )∼_0 ((n(n+1)(2n+1))/(12)) lim_(x→0) ((1−cosx.cos(2x)...cos(nx))/x^2 )=((n(n+1)(2n+1))/(12))
$${cosx}=\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}+{o}\left({x}^{\mathrm{2}} \right) \\ $$$${cos}\mathrm{2}{x}=\mathrm{1}−\frac{\left(\mathrm{2}{x}\right)^{\mathrm{2}} }{\mathrm{2}}+{o}\left({x}^{\mathrm{2}} \right) \\ $$$${cos}\mathrm{3}{x}=\mathrm{1}−\frac{\left(\mathrm{3}{x}\right)^{\mathrm{2}} }{\mathrm{2}}+{o}\left({x}^{\mathrm{2}} \right) \\ $$$${cosx}.{cos}\left(\mathrm{2}{x}\right).{cos}\left(\mathrm{3}{x}\right)…{cos}\left({nx}\right)=\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\right)\left(\mathrm{1}−\frac{\left(\mathrm{2}{x}\right)^{\mathrm{2}} }{\mathrm{2}}\right)…\left(\mathrm{1}−\frac{\left({nx}\right)^{\mathrm{2}} }{\mathrm{2}}\right)+{o}\left({x}^{\mathrm{2}} \right) \\ $$$$=\mathrm{1}−\left(\frac{{x}^{\mathrm{2}} }{\mathrm{2}}+\frac{\left(\mathrm{2}{x}\right)^{\mathrm{2}} }{\mathrm{2}}+\frac{\left(\mathrm{3}{x}\right)^{\mathrm{2}} }{\mathrm{2}}+…\frac{\left({nx}\right)^{\mathrm{2}} }{\mathrm{2}}\right)+{o}\left({x}^{\mathrm{2}} \right) \\ $$$$=\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\left(\mathrm{1}+\mathrm{2}^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} +…+{n}^{\mathrm{2}} \right)+{o}\left({x}^{\mathrm{2}} \right) \\ $$$$\mathrm{1}−{cosx}.{cos}\mathrm{2}{x}…{cos}\left({nx}\right)\underset{\mathrm{0}} {\sim}\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\left(\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{6}}\right) \\ $$$$\frac{\mathrm{1}−{cosx}.{cos}\left(\mathrm{2}{x}\right)…{cos}\left({nx}\right)}{{x}^{\mathrm{2}} }\underset{\mathrm{0}} {\sim}\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{12}} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{\mathrm{1}−{cosx}.{cos}\left(\mathrm{2}{x}\right)…{cos}\left({nx}\right)}{{x}^{\mathrm{2}} }=\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{12}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *