Question Number 136739 by Ar Brandon last updated on 25/Mar/21

$$\mathrm{Given}\:\mathrm{0}<\mathrm{a}<\mathrm{b},\:\mathrm{prove}\:\mathrm{that} \\ $$$$\frac{\left(\mathrm{b}−\mathrm{a}\right)^{\mathrm{2}} }{\mathrm{8b}}\leqslant\frac{\mathrm{a}+\mathrm{b}}{\mathrm{2}}−\sqrt{\mathrm{ab}}\leqslant\frac{\left(\mathrm{b}−\mathrm{a}\right)^{\mathrm{2}} }{\mathrm{8a}} \\ $$
Answered by snipers237 last updated on 26/Mar/21
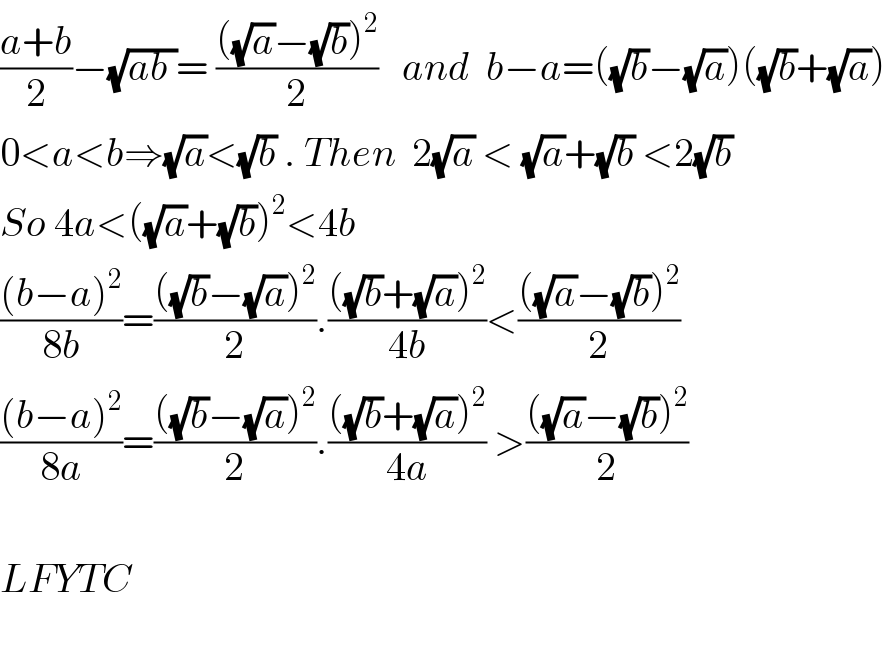
$$\frac{{a}+{b}}{\mathrm{2}}−\sqrt{{ab}\:}=\:\frac{\left(\sqrt{{a}}−\sqrt{{b}}\right)^{\mathrm{2}} }{\mathrm{2}}\:\:\:{and}\:\:{b}−{a}=\left(\sqrt{{b}}−\sqrt{{a}}\right)\left(\sqrt{{b}}+\sqrt{{a}}\right) \\ $$$$\mathrm{0}<{a}<{b}\Rightarrow\sqrt{{a}}<\sqrt{{b}}\:.\:{Then}\:\:\mathrm{2}\sqrt{{a}}\:<\:\sqrt{{a}}+\sqrt{{b}}\:<\mathrm{2}\sqrt{{b}}\: \\ $$$${So}\:\mathrm{4}{a}<\left(\sqrt{{a}}+\sqrt{{b}}\right)^{\mathrm{2}} <\mathrm{4}{b} \\ $$$$\frac{\left({b}−{a}\right)^{\mathrm{2}} }{\mathrm{8}{b}}=\frac{\left(\sqrt{{b}}−\sqrt{{a}}\right)^{\mathrm{2}} }{\mathrm{2}}.\frac{\left(\sqrt{{b}}+\sqrt{{a}}\right)^{\mathrm{2}} }{\mathrm{4}{b}}<\frac{\left(\sqrt{{a}}−\sqrt{{b}}\right)^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\frac{\left({b}−{a}\right)^{\mathrm{2}} }{\mathrm{8}{a}}=\frac{\left(\sqrt{{b}}−\sqrt{{a}}\right)^{\mathrm{2}} }{\mathrm{2}}.\frac{\left(\sqrt{{b}}+\sqrt{{a}}\right)^{\mathrm{2}} }{\mathrm{4}{a}}\:>\frac{\left(\sqrt{{a}}−\sqrt{{b}}\right)^{\mathrm{2}} }{\mathrm{2}}\: \\ $$$$ \\ $$$${LFYTC} \\ $$$$ \\ $$
Commented by Ar Brandon last updated on 26/Mar/21

$$\mathrm{Thanks} \\ $$
