Question Number 58627 by rahul 19 last updated on 26/Apr/19
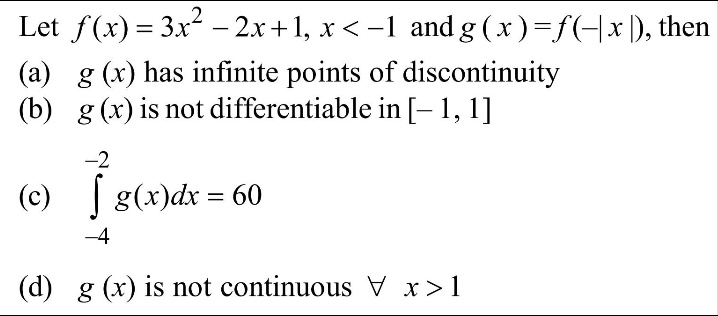
Commented by rahul 19 last updated on 26/Apr/19

$${Ans}:\:{a},{b},{c} \\ $$
Commented by tanmay last updated on 26/Apr/19
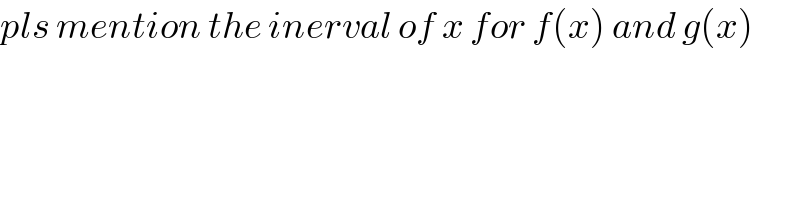
$${pls}\:{mention}\:{the}\:{inerval}\:{of}\:{x}\:{for}\:{f}\left({x}\right)\:{and}\:{g}\left({x}\right) \\ $$$$ \\ $$
Commented by rahul 19 last updated on 26/Apr/19
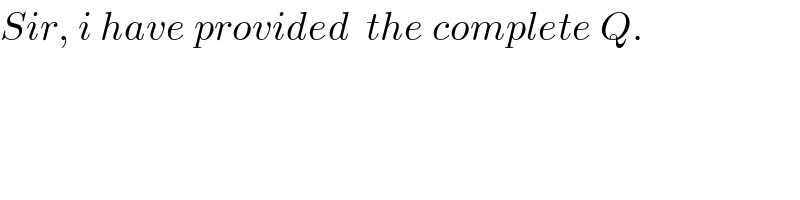
$${Sir},\:{i}\:{have}\:{provided}\:\:{the}\:{complete}\:{Q}. \\ $$
Answered by tanmay last updated on 27/Apr/19
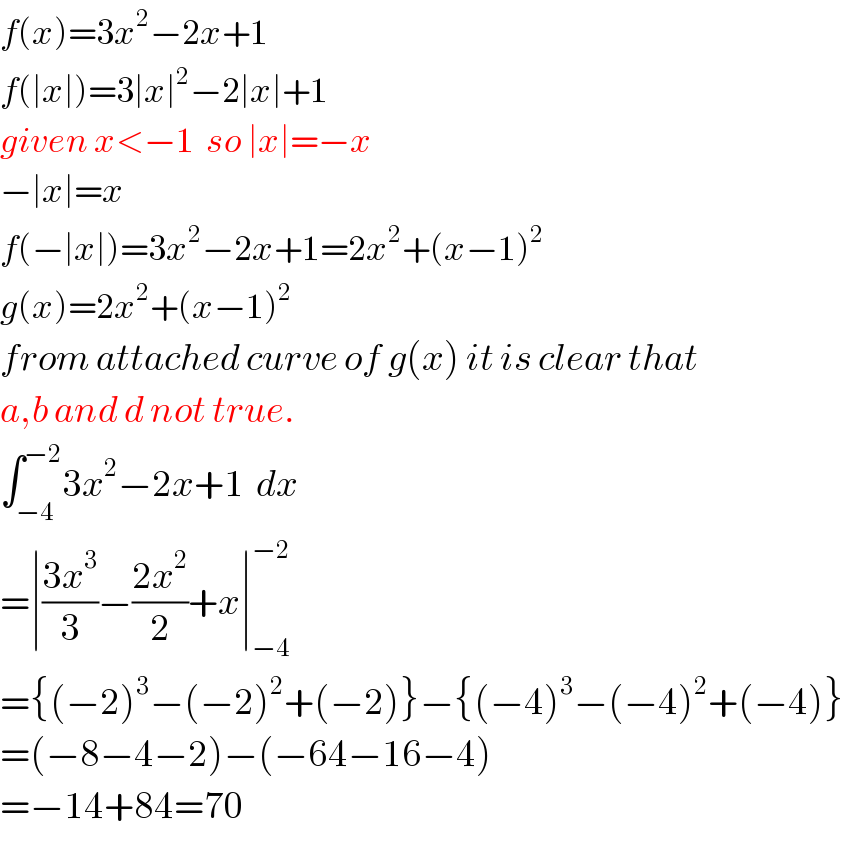
$${f}\left({x}\right)=\mathrm{3}{x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{1} \\ $$$${f}\left(\mid{x}\mid\right)=\mathrm{3}\mid{x}\mid^{\mathrm{2}} −\mathrm{2}\mid{x}\mid+\mathrm{1} \\ $$$${given}\:{x}<−\mathrm{1}\:\:{so}\:\mid{x}\mid=−{x} \\ $$$$−\mid{x}\mid={x} \\ $$$${f}\left(−\mid{x}\mid\right)=\mathrm{3}{x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{1}=\mathrm{2}{x}^{\mathrm{2}} +\left({x}−\mathrm{1}\right)^{\mathrm{2}} \\ $$$${g}\left({x}\right)=\mathrm{2}{x}^{\mathrm{2}} +\left({x}−\mathrm{1}\right)^{\mathrm{2}} \\ $$$${from}\:{attached}\:{curve}\:{of}\:{g}\left({x}\right)\:{it}\:{is}\:{clear}\:{that} \\ $$$${a},{b}\:{and}\:{d}\:{not}\:{true}.\: \\ $$$$\int_{−\mathrm{4}} ^{−\mathrm{2}} \mathrm{3}{x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{1}\:\:{dx} \\ $$$$=\mid\frac{\mathrm{3}{x}^{\mathrm{3}} }{\mathrm{3}}−\frac{\mathrm{2}{x}^{\mathrm{2}} }{\mathrm{2}}+{x}\mid_{−\mathrm{4}} ^{−\mathrm{2}} \\ $$$$=\left\{\left(−\mathrm{2}\right)^{\mathrm{3}} −\left(−\mathrm{2}\right)^{\mathrm{2}} +\left(−\mathrm{2}\right)\right\}−\left\{\left(−\mathrm{4}\right)^{\mathrm{3}} −\left(−\mathrm{4}\right)^{\mathrm{2}} +\left(−\mathrm{4}\right)\right\} \\ $$$$=\left(−\mathrm{8}−\mathrm{4}−\mathrm{2}\right)−\left(−\mathrm{64}−\mathrm{16}−\mathrm{4}\right) \\ $$$$=−\mathrm{14}+\mathrm{84}=\mathrm{70} \\ $$
Commented by tanmay last updated on 27/Apr/19

Commented by rahul 19 last updated on 28/Apr/19

$${thank}\:{u}\:{sir}. \\ $$
