Question Number 124634 by bemath last updated on 05/Dec/20
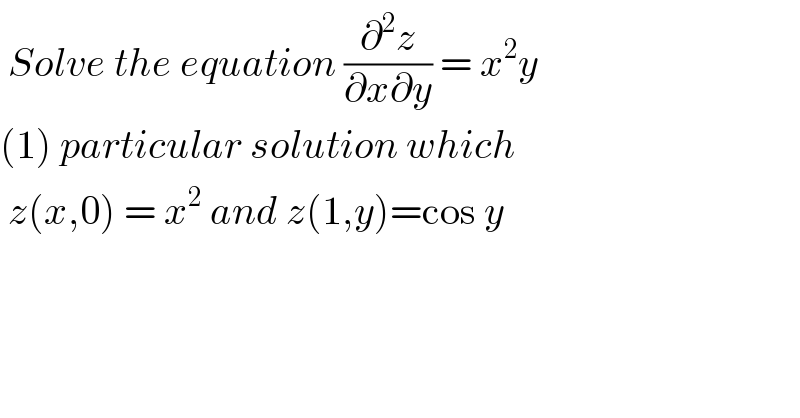
$$\:{Solve}\:{the}\:{equation}\:\frac{\partial^{\mathrm{2}} {z}}{\partial{x}\partial{y}}\:=\:{x}^{\mathrm{2}} {y} \\ $$$$\left(\mathrm{1}\right)\:{particular}\:{solution}\:{which}\: \\ $$$$\:{z}\left({x},\mathrm{0}\right)\:=\:{x}^{\mathrm{2}} \:{and}\:{z}\left(\mathrm{1},{y}\right)=\mathrm{cos}\:{y} \\ $$$$ \\ $$
Answered by liberty last updated on 05/Dec/20
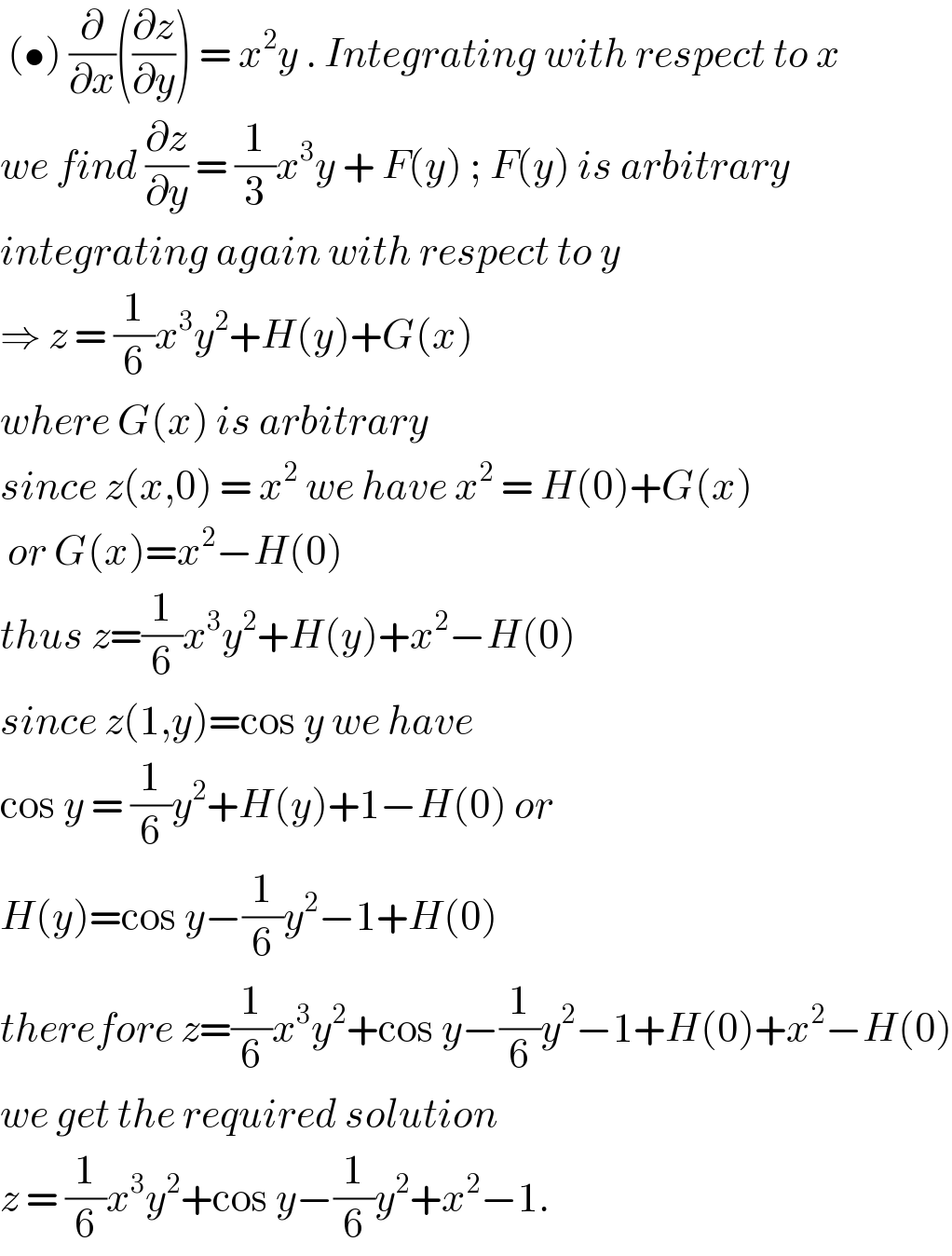
$$\:\left(\bullet\right)\:\frac{\partial}{\partial{x}}\left(\frac{\partial{z}}{\partial{y}}\right)\:=\:{x}^{\mathrm{2}} {y}\:.\:{Integrating}\:{with}\:{respect}\:{to}\:{x} \\ $$$${we}\:{find}\:\frac{\partial{z}}{\partial{y}}\:=\:\frac{\mathrm{1}}{\mathrm{3}}{x}^{\mathrm{3}} {y}\:+\:{F}\left({y}\right)\:;\:{F}\left({y}\right)\:{is}\:{arbitrary} \\ $$$${integrating}\:{again}\:{with}\:{respect}\:{to}\:{y}\: \\ $$$$\Rightarrow\:{z}\:=\:\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{3}} {y}^{\mathrm{2}} +{H}\left({y}\right)+{G}\left({x}\right) \\ $$$${where}\:{G}\left({x}\right)\:{is}\:{arbitrary}\: \\ $$$${since}\:{z}\left({x},\mathrm{0}\right)\:=\:{x}^{\mathrm{2}} \:{we}\:{have}\:{x}^{\mathrm{2}} \:=\:{H}\left(\mathrm{0}\right)+{G}\left({x}\right) \\ $$$$\:{or}\:{G}\left({x}\right)={x}^{\mathrm{2}} −{H}\left(\mathrm{0}\right)\: \\ $$$${thus}\:{z}=\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{3}} {y}^{\mathrm{2}} +{H}\left({y}\right)+{x}^{\mathrm{2}} −{H}\left(\mathrm{0}\right) \\ $$$${since}\:{z}\left(\mathrm{1},{y}\right)=\mathrm{cos}\:{y}\:{we}\:{have}\: \\ $$$$\mathrm{cos}\:{y}\:=\:\frac{\mathrm{1}}{\mathrm{6}}{y}^{\mathrm{2}} +{H}\left({y}\right)+\mathrm{1}−{H}\left(\mathrm{0}\right)\:{or} \\ $$$${H}\left({y}\right)=\mathrm{cos}\:{y}−\frac{\mathrm{1}}{\mathrm{6}}{y}^{\mathrm{2}} −\mathrm{1}+{H}\left(\mathrm{0}\right) \\ $$$${therefore}\:{z}=\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{3}} {y}^{\mathrm{2}} +\mathrm{cos}\:{y}−\frac{\mathrm{1}}{\mathrm{6}}{y}^{\mathrm{2}} −\mathrm{1}+{H}\left(\mathrm{0}\right)+{x}^{\mathrm{2}} −{H}\left(\mathrm{0}\right) \\ $$$${we}\:{get}\:{the}\:{required}\:{solution}\: \\ $$$${z}\:=\:\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{3}} {y}^{\mathrm{2}} +\mathrm{cos}\:{y}−\frac{\mathrm{1}}{\mathrm{6}}{y}^{\mathrm{2}} +{x}^{\mathrm{2}} −\mathrm{1}.\: \\ $$
