Menu Close

find-solution-d-2-y-dx-2-4-dy-dx-3-cosec-

Tinku Tara 0 Comments
Pin




Question Number 125691 by fajri last updated on 13/Dec/20
find solution : (d^2 y/dx^(2 ) ) + 4(dy/dx) = 3 cosec θ
$${find}\:{solution}\:: \\ $$$$\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}\:} }\:+\:\mathrm{4}\frac{{dy}}{{dx}}\:=\:\mathrm{3}\:{cosec}\:\theta \\ $$
Answered by mathmax by abdo last updated on 14/Dec/20
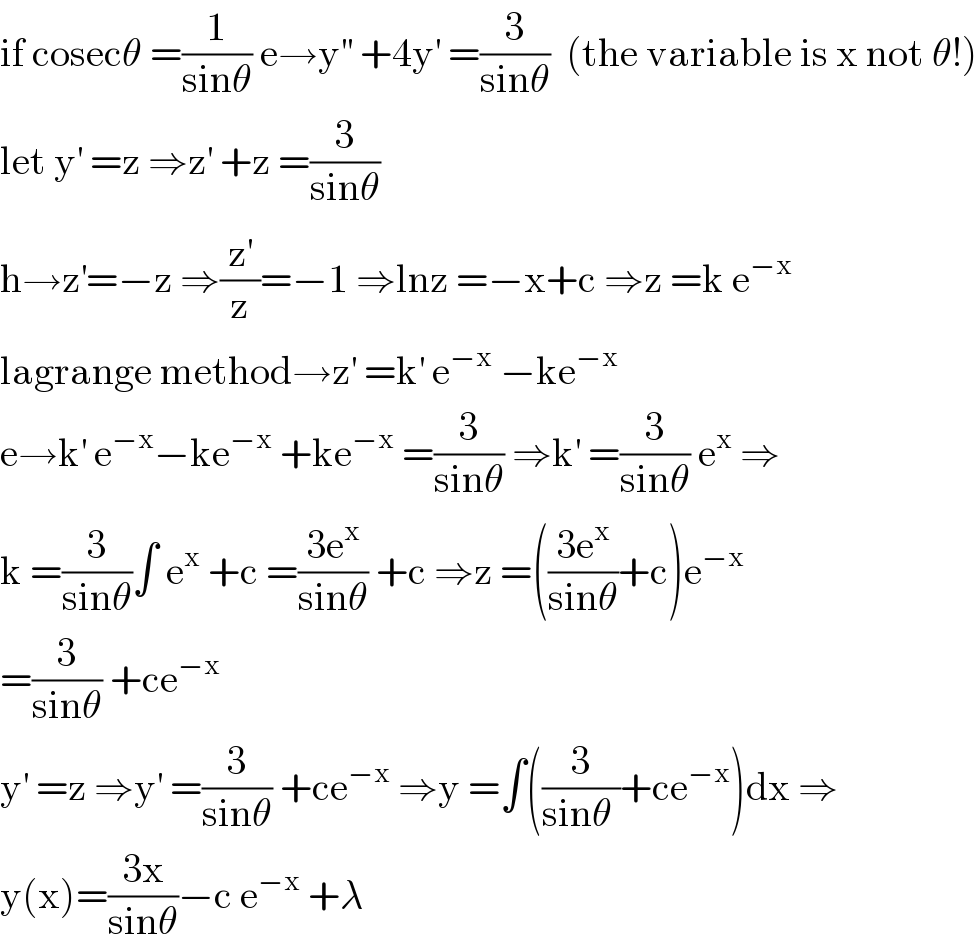
if cosecθ =(1/(sinθ)) e→y^(′′) +4y^′ =(3/(sinθ)) (the variable is x not θ!) let y^′ =z ⇒z^′ +z =(3/(sinθ)) h→z^′ =−z ⇒(z^′ /z)=−1 ⇒lnz =−x+c ⇒z =k e^(−x) lagrange method→z^′ =k^′ e^(−x) −ke^(−x) e→k^′ e^(−x) −ke^(−x) +ke^(−x) =(3/(sinθ)) ⇒k^′ =(3/(sinθ)) e^x ⇒ k =(3/(sinθ))∫ e^x +c =((3e^x )/(sinθ)) +c ⇒z =(((3e^x )/(sinθ))+c)e^(−x) =(3/(sinθ)) +ce^(−x) y^′ =z ⇒y^′ =(3/(sinθ)) +ce^(−x) ⇒y =∫((3/(sinθ ))+ce^(−x) )dx ⇒ y(x)=((3x)/(sinθ))−c e^(−x) +λ
$$\mathrm{if}\:\mathrm{cosec}\theta\:=\frac{\mathrm{1}}{\mathrm{sin}\theta}\:\mathrm{e}\rightarrow\mathrm{y}^{''} \:+\mathrm{4y}^{'} \:=\frac{\mathrm{3}}{\mathrm{sin}\theta}\:\:\left(\mathrm{the}\:\mathrm{variable}\:\mathrm{is}\:\mathrm{x}\:\mathrm{not}\:\theta!\right) \\ $$$$\mathrm{let}\:\mathrm{y}^{'} \:=\mathrm{z}\:\Rightarrow\mathrm{z}^{'} \:+\mathrm{z}\:=\frac{\mathrm{3}}{\mathrm{sin}\theta} \\ $$$$\mathrm{h}\rightarrow\mathrm{z}^{'} =−\mathrm{z}\:\Rightarrow\frac{\mathrm{z}^{'} }{\mathrm{z}}=−\mathrm{1}\:\Rightarrow\mathrm{lnz}\:=−\mathrm{x}+\mathrm{c}\:\Rightarrow\mathrm{z}\:=\mathrm{k}\:\mathrm{e}^{−\mathrm{x}} \\ $$$$\mathrm{lagrange}\:\mathrm{method}\rightarrow\mathrm{z}^{'} \:=\mathrm{k}^{'} \:\mathrm{e}^{−\mathrm{x}} \:−\mathrm{ke}^{−\mathrm{x}} \\ $$$$\mathrm{e}\rightarrow\mathrm{k}^{'} \:\mathrm{e}^{−\mathrm{x}} −\mathrm{ke}^{−\mathrm{x}} \:+\mathrm{ke}^{−\mathrm{x}} \:=\frac{\mathrm{3}}{\mathrm{sin}\theta}\:\Rightarrow\mathrm{k}^{'} \:=\frac{\mathrm{3}}{\mathrm{sin}\theta}\:\mathrm{e}^{\mathrm{x}} \:\Rightarrow \\ $$$$\mathrm{k}\:=\frac{\mathrm{3}}{\mathrm{sin}\theta}\int\:\mathrm{e}^{\mathrm{x}} \:+\mathrm{c}\:=\frac{\mathrm{3e}^{\mathrm{x}} }{\mathrm{sin}\theta}\:+\mathrm{c}\:\Rightarrow\mathrm{z}\:=\left(\frac{\mathrm{3e}^{\mathrm{x}} }{\mathrm{sin}\theta}+\mathrm{c}\right)\mathrm{e}^{−\mathrm{x}} \\ $$$$=\frac{\mathrm{3}}{\mathrm{sin}\theta}\:+\mathrm{ce}^{−\mathrm{x}} \\ $$$$\mathrm{y}^{'} \:=\mathrm{z}\:\Rightarrow\mathrm{y}^{'} \:=\frac{\mathrm{3}}{\mathrm{sin}\theta}\:+\mathrm{ce}^{−\mathrm{x}} \:\Rightarrow\mathrm{y}\:=\int\left(\frac{\mathrm{3}}{\mathrm{sin}\theta\:}+\mathrm{ce}^{−\mathrm{x}} \right)\mathrm{dx}\:\Rightarrow \\ $$$$\mathrm{y}\left(\mathrm{x}\right)=\frac{\mathrm{3x}}{\mathrm{sin}\theta}−\mathrm{c}\:\mathrm{e}^{−\mathrm{x}} \:+\lambda \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *